Hashi Puzzles
One of the things I’ve most liked about introducing my students to new logic puzzles is that they’ve been new to me as well! I was initially hesitant to try the hashiwokakero puzzles with my students because they sounded scary. I learned about these puzzles from reading the slides from Jeffrey Wanko’s NCTM presentation. You can check out the slides from his various presentations on using logic puzzles in the math classroom on his website.
My kids preferred calling these “Bridge” puzzles. And, I’m totally okay with that. Though, I find myself thinking of them as “Hashi” puzzles.
I put a puzzle on the SMARTBoard for us to solve together as a class. After working through it together with a few bumps along the way, I gave students their own two puzzles to solve. (Puzzles B and C from the previously linked document).
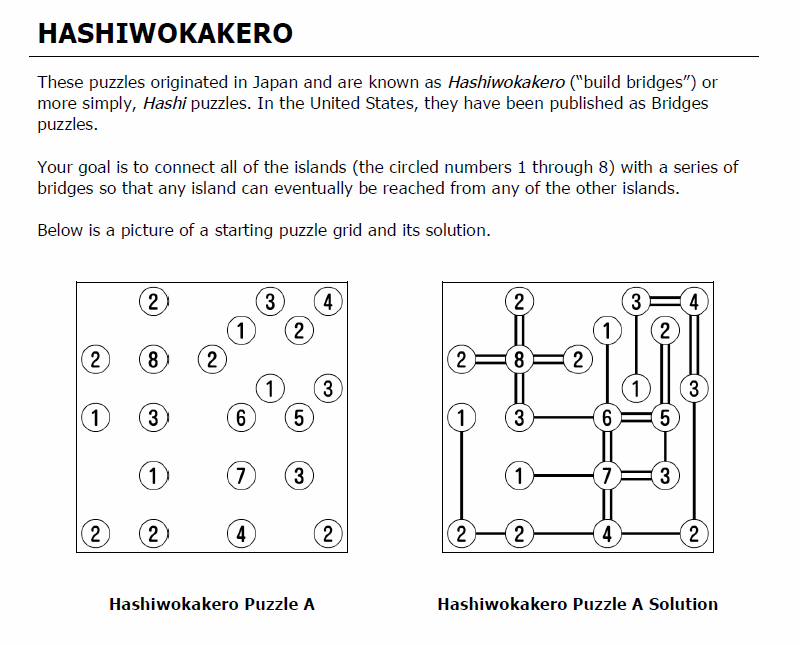
Here are the rules for hashi puzzles:
• The bridges must begin and end at islands, traveling in a straight line (horizontally or
vertically)
• The bridges must not cross any other bridges or islands
• No more than two bridges can connect a pair of islands
• The total number of bridges connected to each island must match the number on that
island
Several students decided these were their favorite logic puzzles yet.
I let one of my classes vote one day as to which puzzle they would like a chance to do again, and these puzzles were a huge winner by a landslide.




Awesome resources! Thank you so much for sharing! 🙂
You're welcome!
Thanks for sharing! I teach an elective Problem Solving Seminar for 7th graders. They love Ken Ken and I am going to try some of these with them over the next few weeks.
That class sounds like it would be a blast to teach!
The link to the website of lesson plans for logic puzzles doesn't seem to be working. 🙁
It's working for me. Send me an e-mail, and I will send you the PDFs.
PertierraM@duvalschools.org
I wonder if there is a version in which you can provide "moving islands," so that the students must place the numbered islands in a way to enable the building of bridges within the rules. (This problem is made a bit easier if any straight line bridges — no curves; yes diagonals — are allowed, rather than just horizontal and vertical bridges.)