| | | |

Factoring Polynomials Using the Box Method Directions

I decided to put together a set of step-by-step directions for factoring polynomials using the box method.

When I posted my interactive notebook pages for our Algebra 1 unit on Polynomials, I said that I was going to post step-by-step photographs of how to use the box method to factor polynomials.  Here’s that post.

Begin by drawing a box.  Quadratic trinomials require a 2 x 2 box for factoring.  This box will also work for difference of squares factoring.

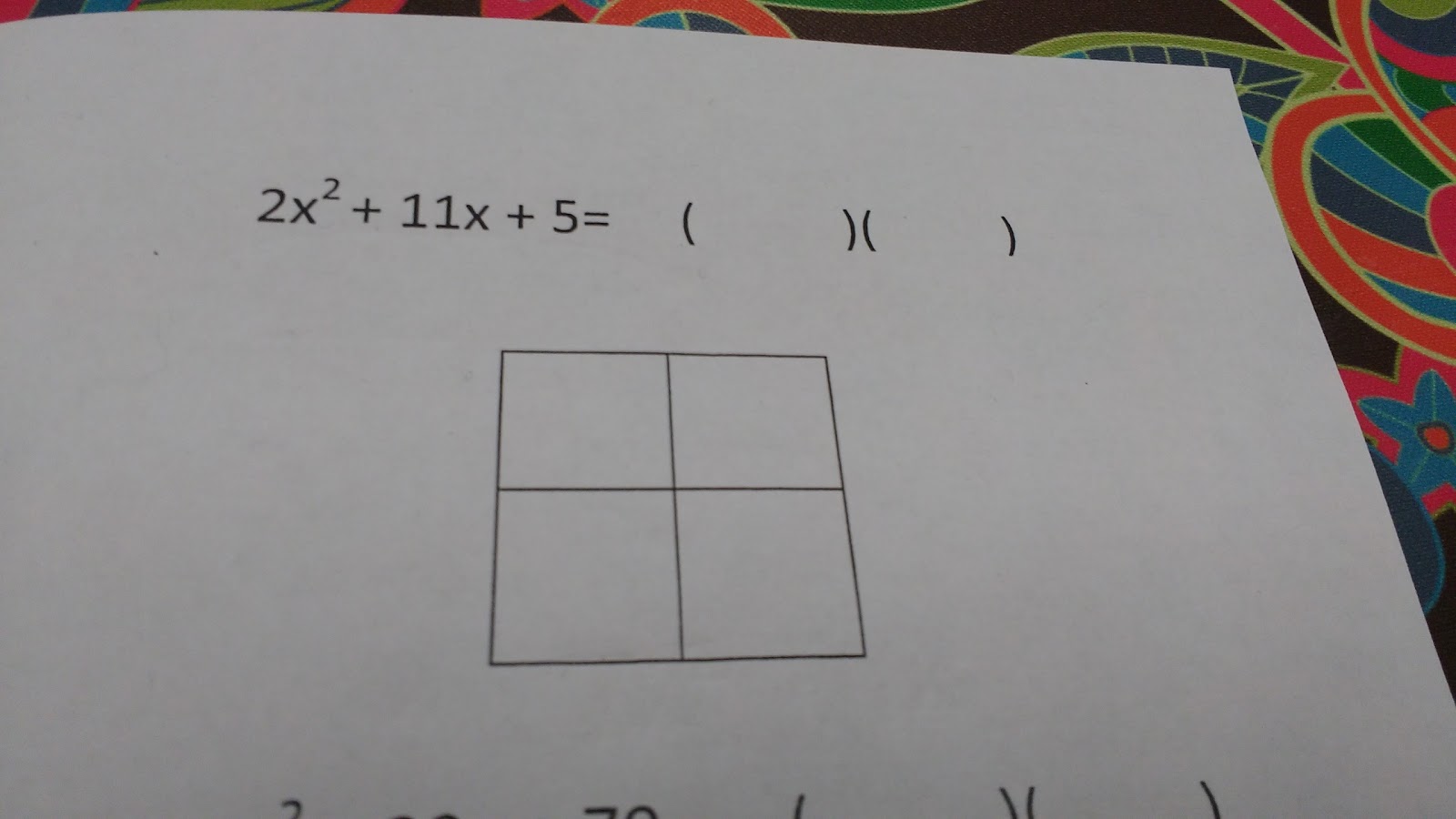
ALWAYS check to see if you can factor out a GCF from the polynomial first.  If you can, this goes in front of the parentheses in your answer.  This year, I added a space between the equal sign and the first parenthesis to give students a place to write the GCF.

There isn’t a number that divides evenly into 2, 11, and 5, so we can skip this step!

The x squared term is written in the top left hand box.  The constant term is written in the bottom right hand box.  Students should be used to this as a result of lots of multiplying polynomial problems using the box method!

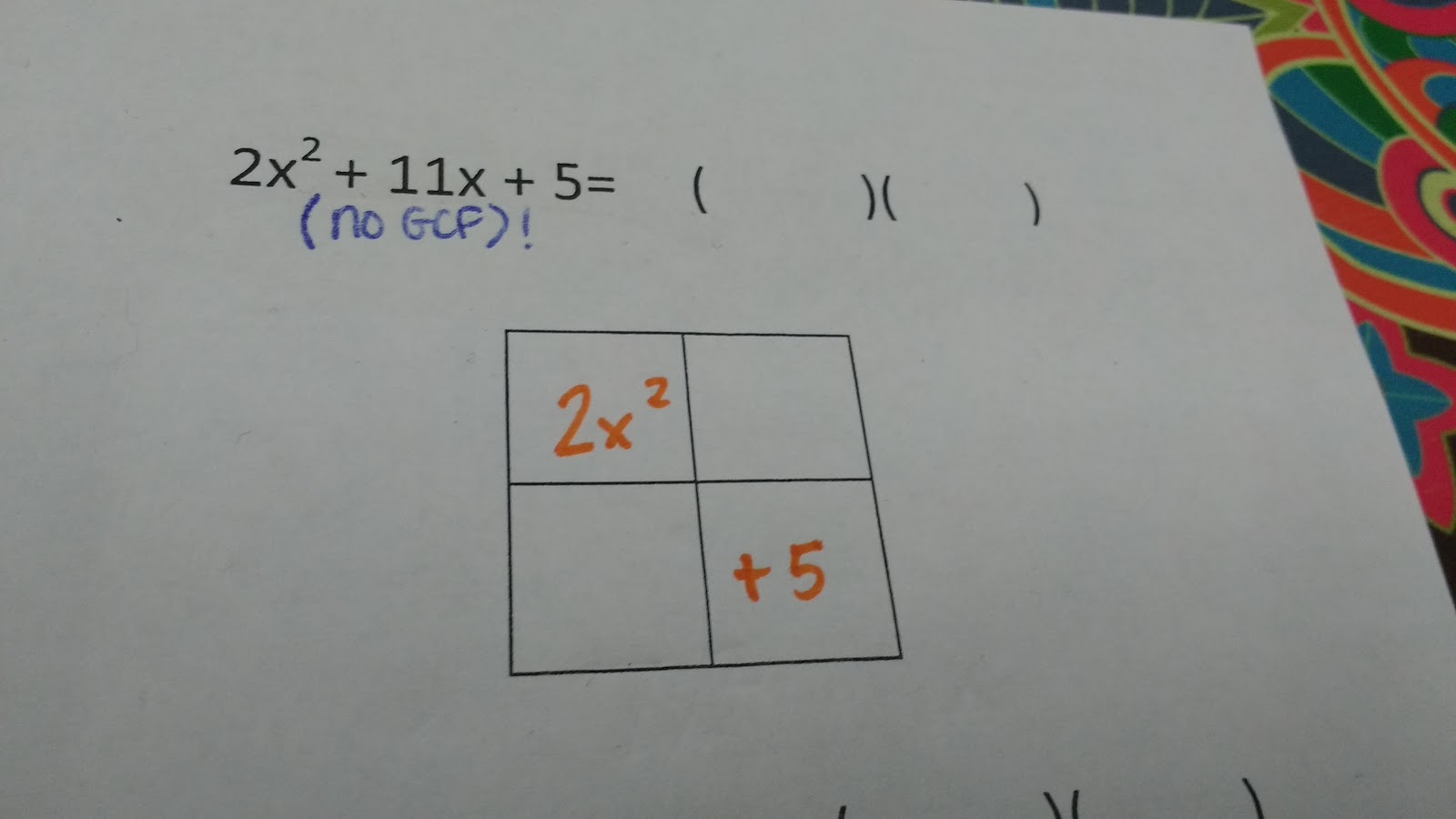
This takes care of the 2x^2 and the 5 from 2x^2 + 11x + 5.  The 11x term tells me that my two missing boxes add to 11x.  I have my students draw an arrow and write this fact next to the arrow.

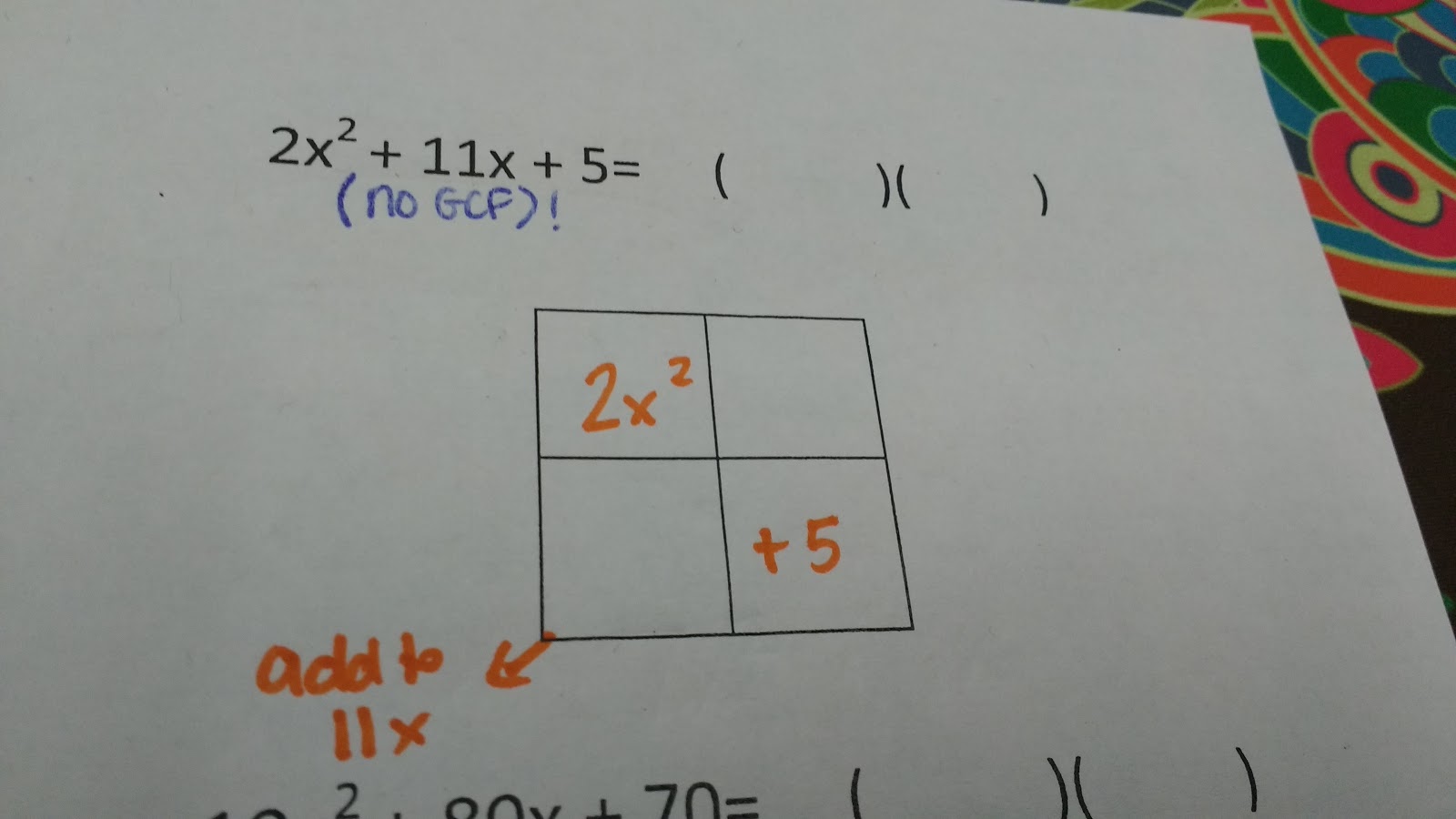
We know from examining patterns with multiplying polynomials that are two missing boxes multiply to the exact same value as the two boxes we have filled in.

This means we are looking for two terms that add to 11x and multiply to 10x^2.

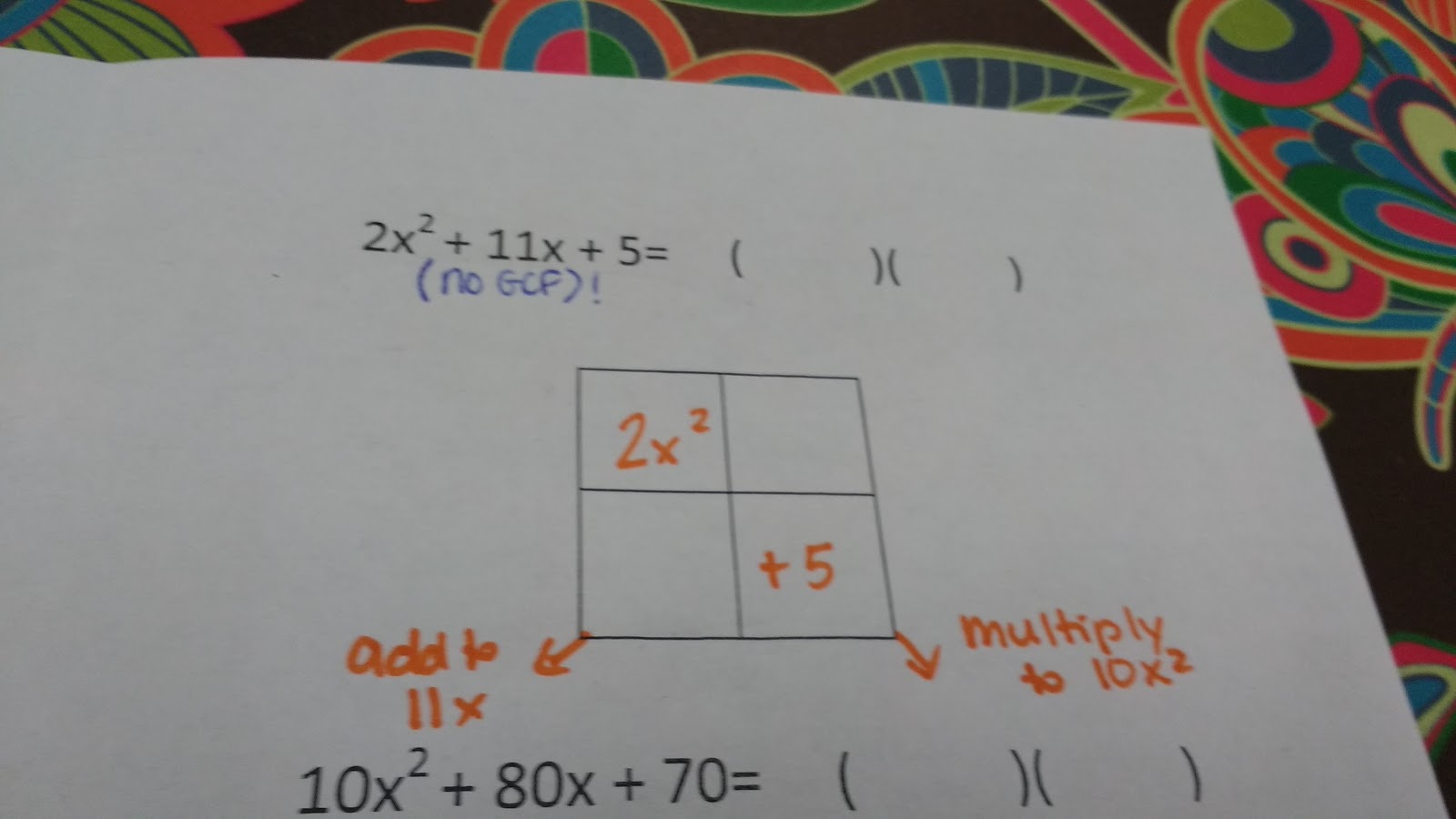
Those two terms are 10x and 1x.  Write those terms in the missing boxes.

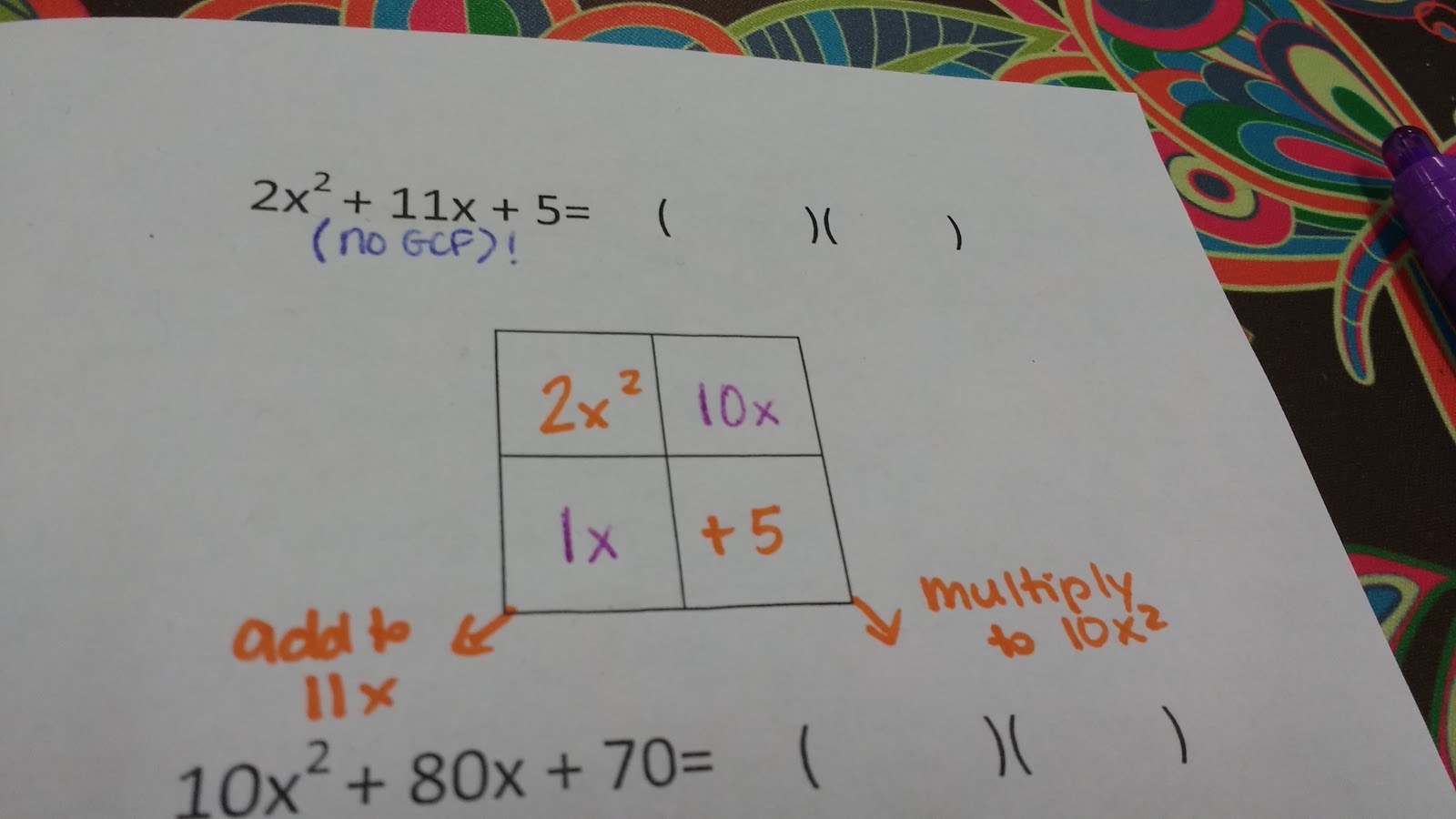
Now, we’re almost done.  We need to figure out what to put on the outside of our boxes to give us these multiplication results.  I have my students begin by picking two boxes and finding the GCF of those two boxes.

For example, the GCF of 2x^2 and 10x is 2x.  So, I can write 2x on the outside of those two boxes.

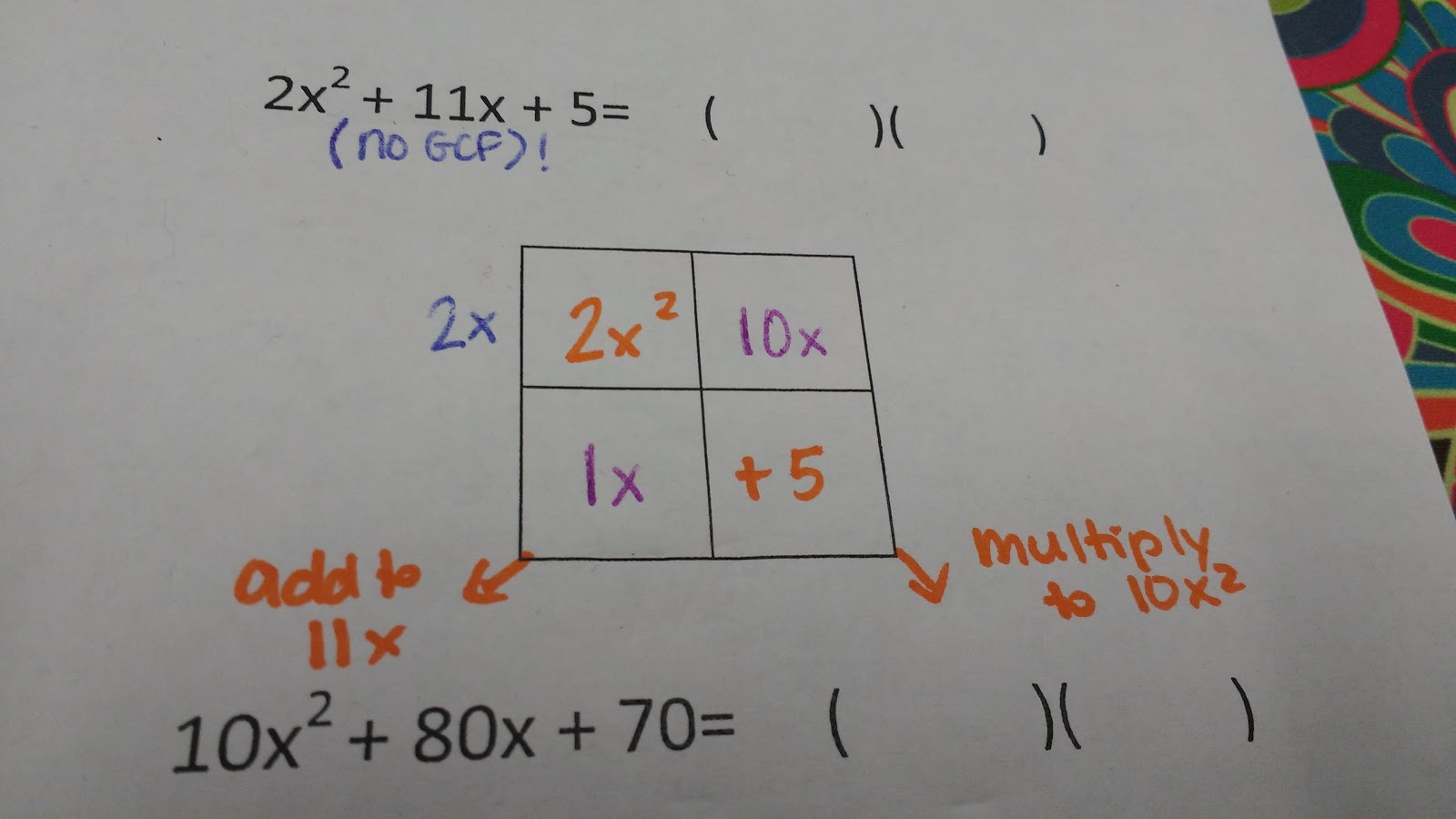
Similarly, x is the GCF of 2x^2 and 1x.

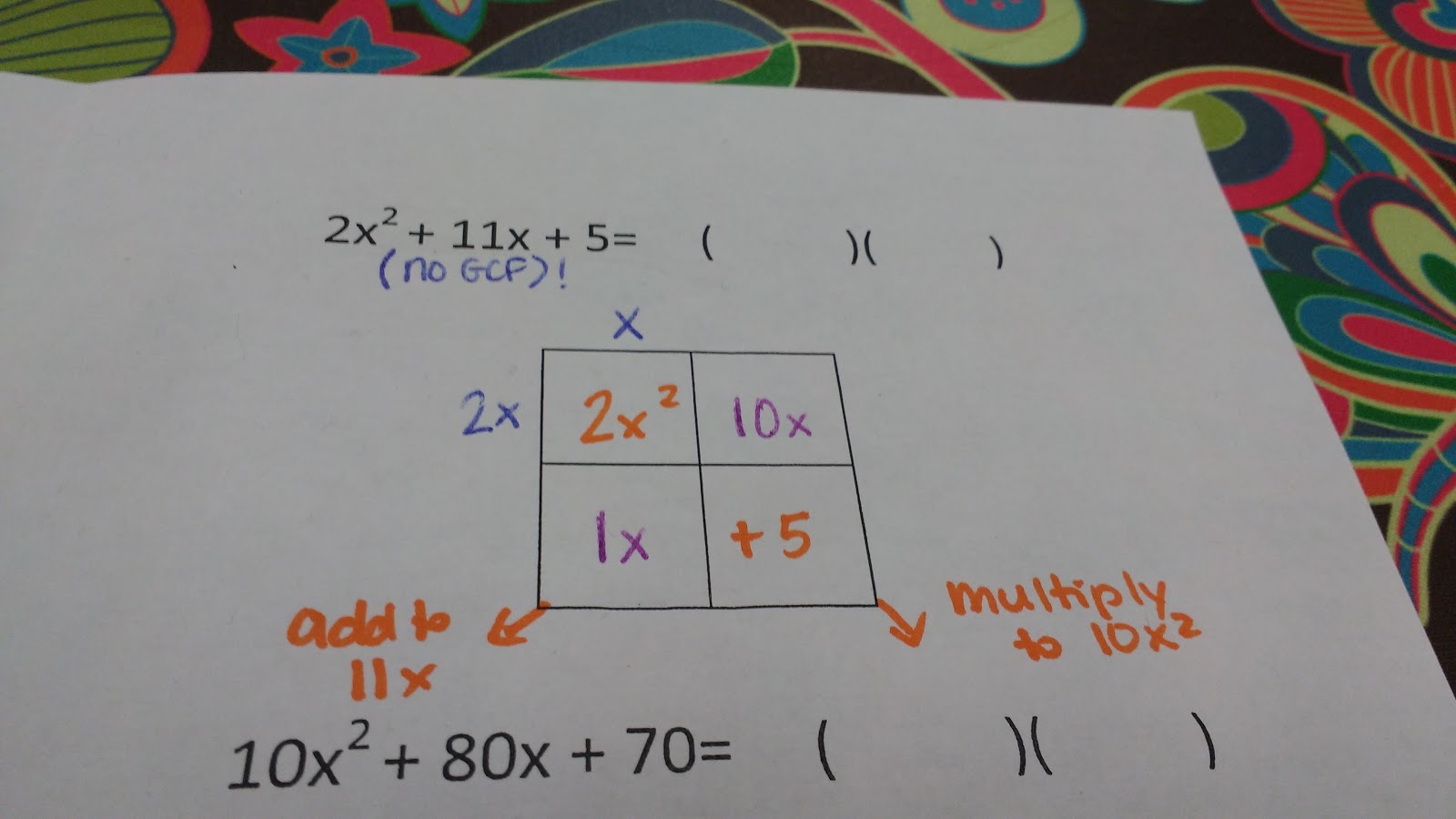
5 is the GCF of 10x and 5.

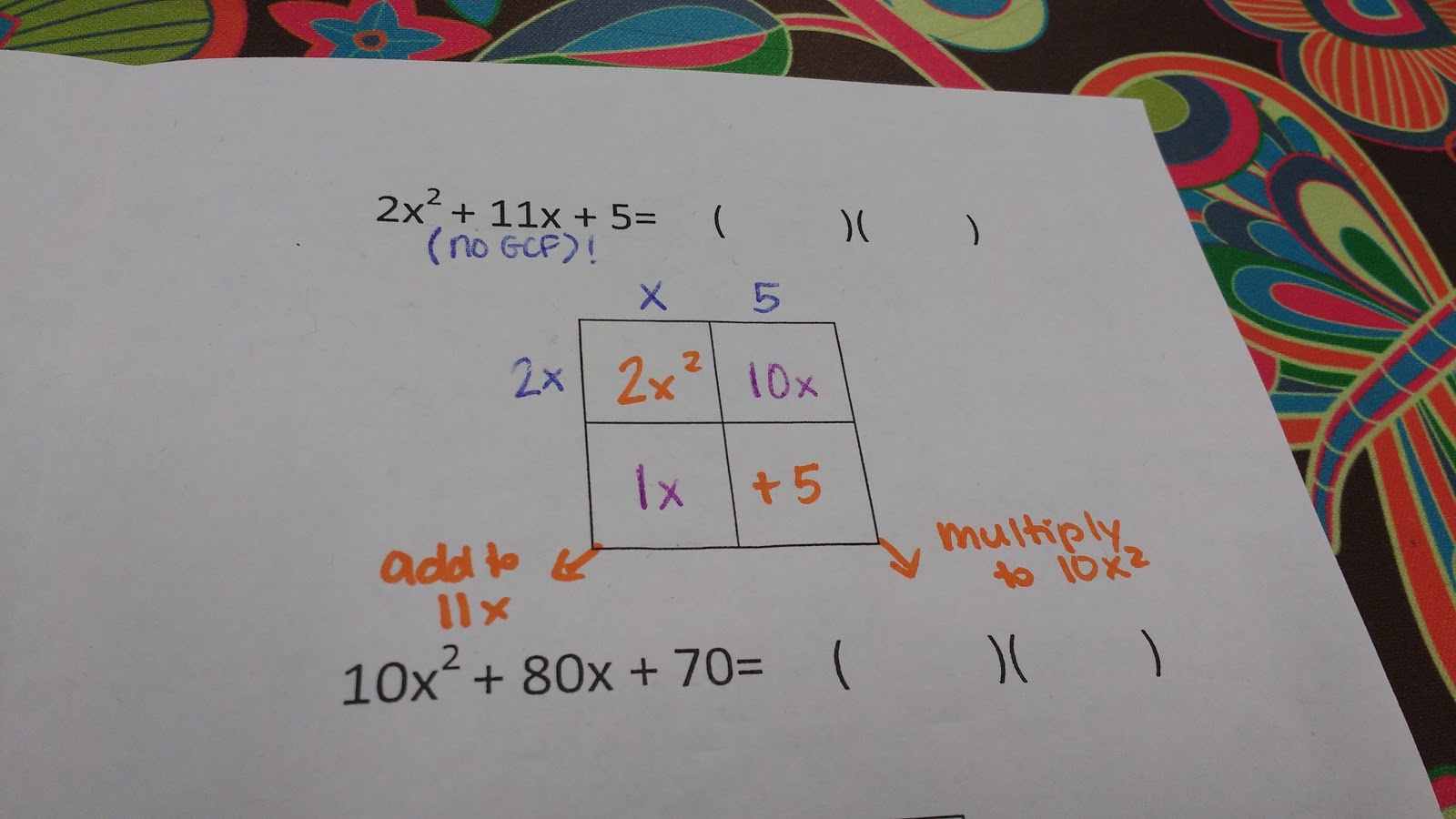
And, 1 is the GCF of 1x and 5.

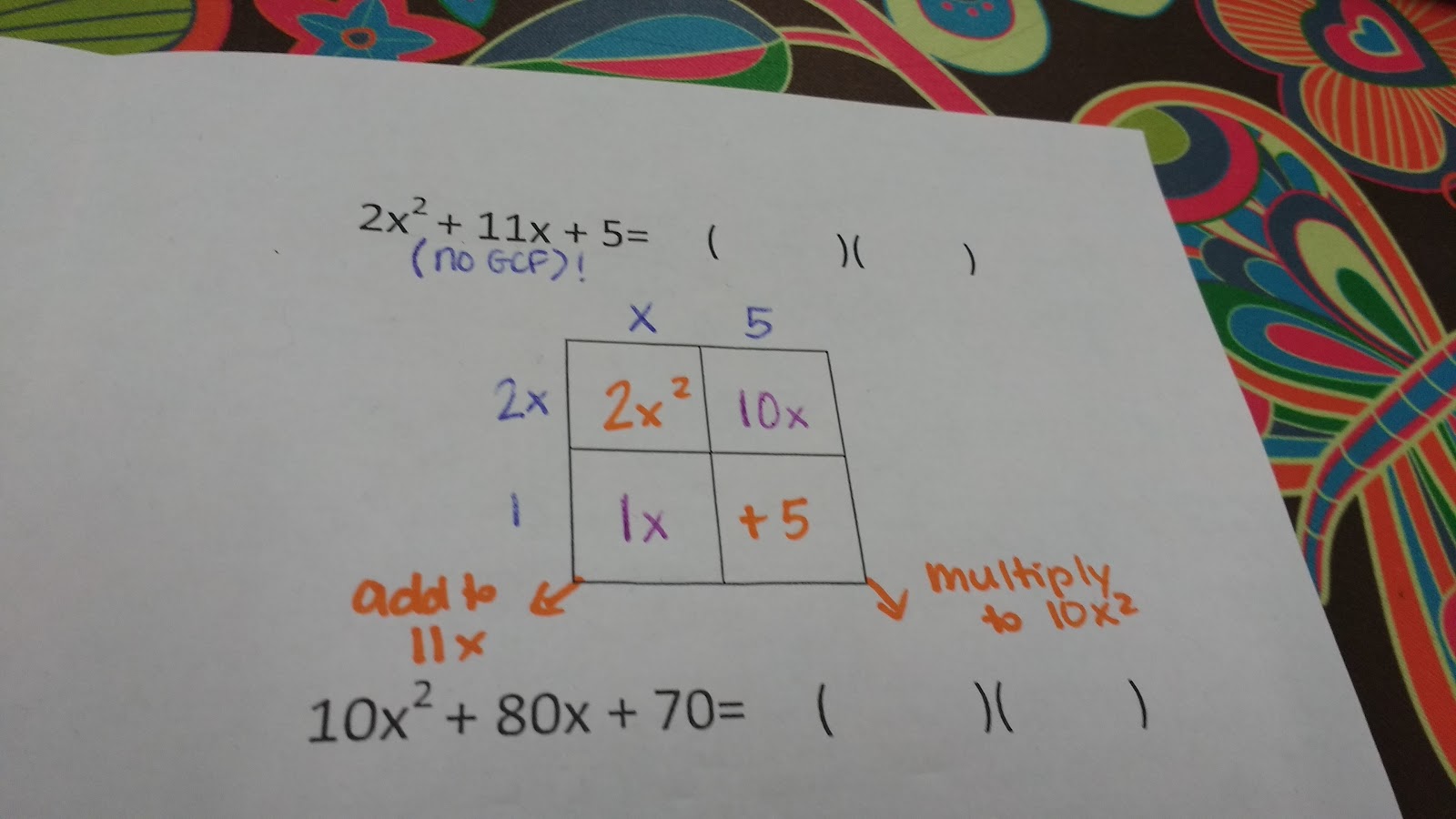
At this point, I instruct my students to always stop and double check that their multiplication is correct.  Every once in a while, there will be an issue with positives and negatives that needs to be cleared up.

Once everything is double-checked, it’s time to write the answer.  Since we didn’t have a GCF to factor out at the beginning, we don’t need to worry about putting a value in front of the parentheses.  

The terms from the side of the box (2x and +1) go in one set of parentheses.  The terms from the top of the box (x and +5) go in the other set of parentheses.  We have successfully factored a quadratic trinomial!

Let’s try another one!

First, we need to check and see if we can factor out a GCF.  10, 80, and 70 are all divisible by 10.

When I divide all three terms by 10, I am left with x^2 + 8x + 7 to factor.

I’m going to go ahead and take the 10 I factored out and put it in front of the parentheses for my final answer.

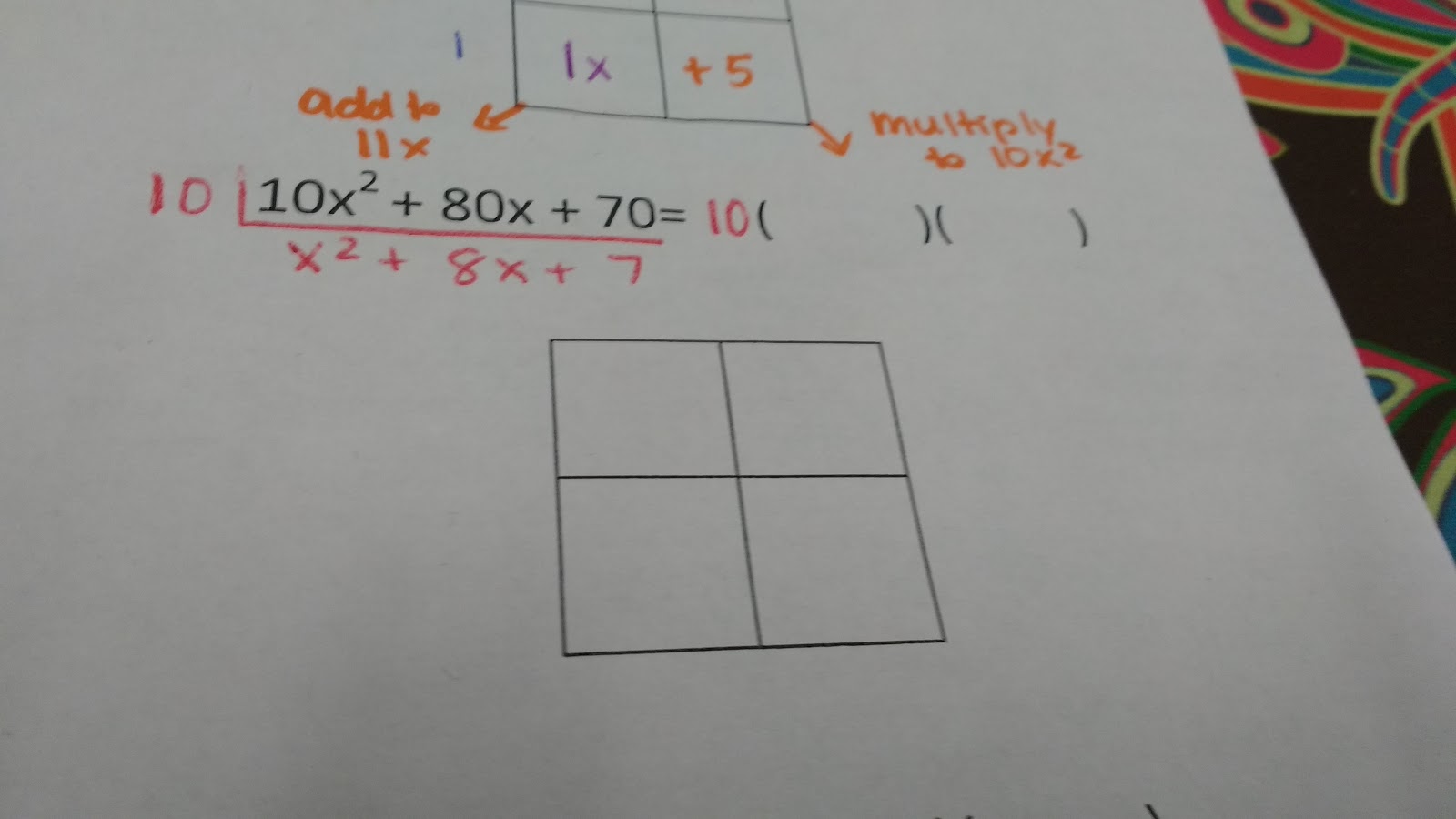
My x^2 term goes in the top left square.  My constant of 7 goes in the bottom right square.

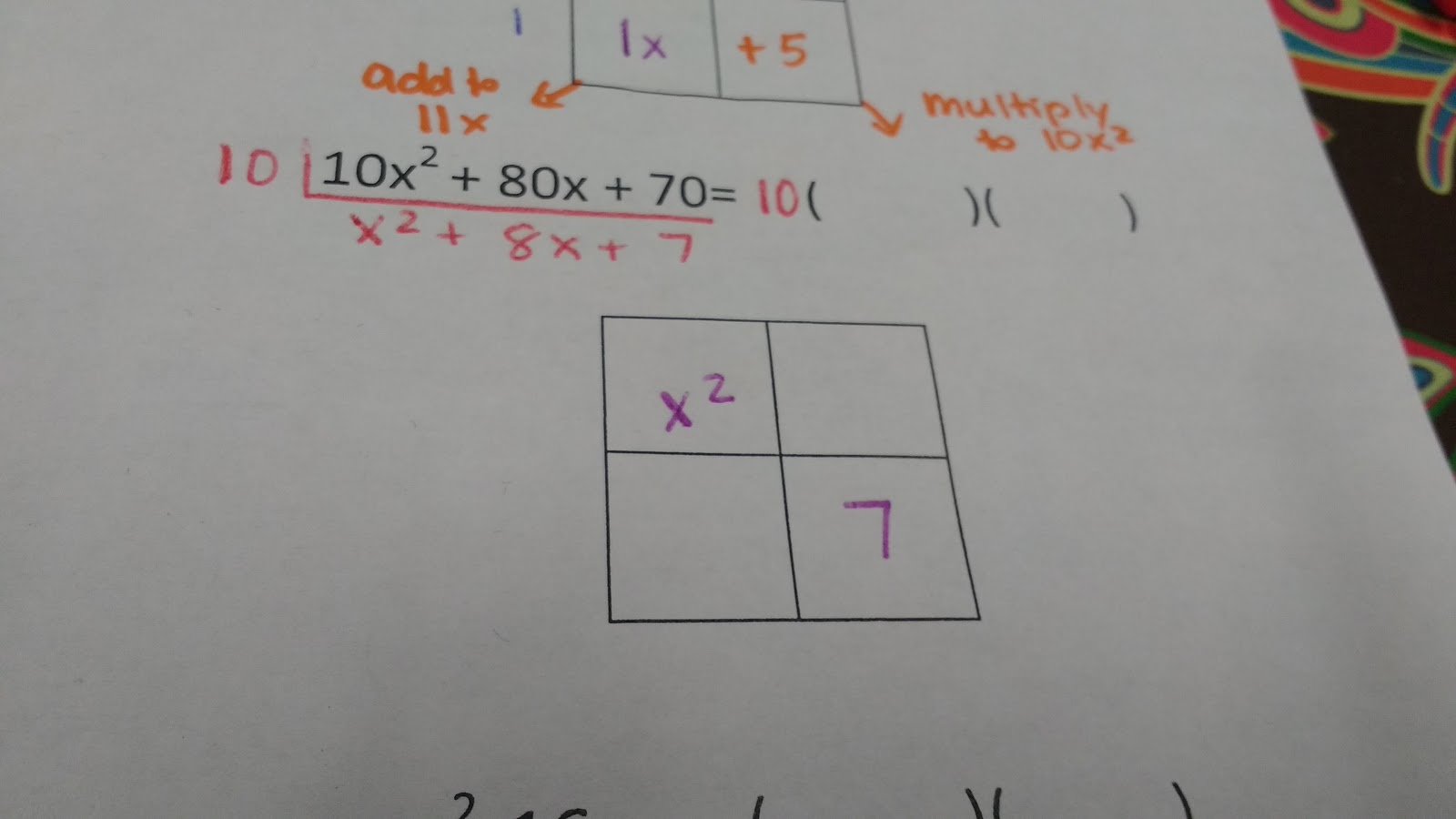
My two empty boxes must add up to 8x.

Lots of terms add up to 8x, so I need more information.  I know that my two missing terms multiply to the same value as the two given terms.  So, I’m looking for two terms that add to 8x and multiply to 7x^2.

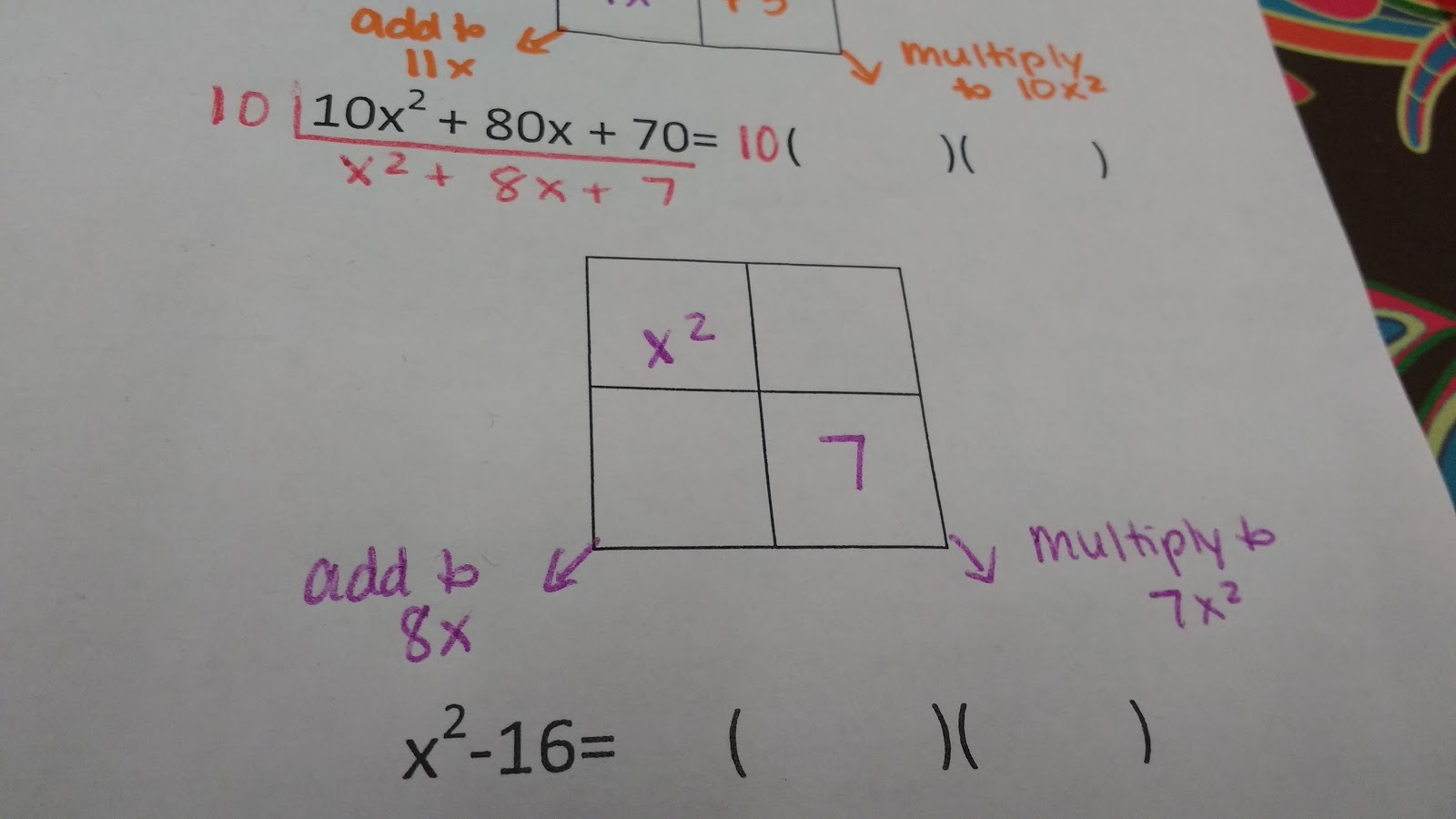
Those two terms would have to be 7x and 1x.  So, I write those in the missing boxes.

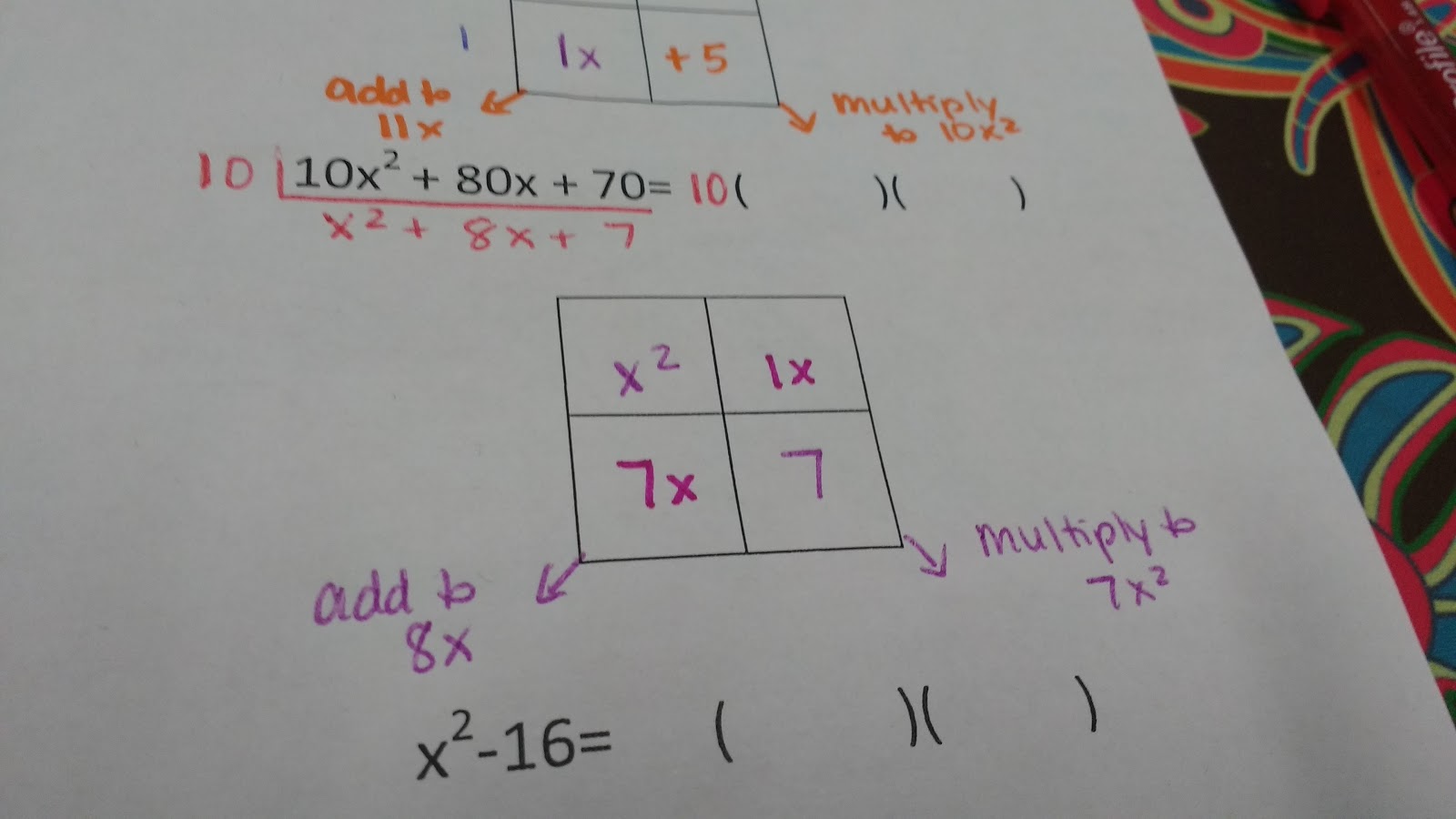
Now, I start looking for the values that go on the outside of the boxes.  The GCF of x^2 and 1x is x.

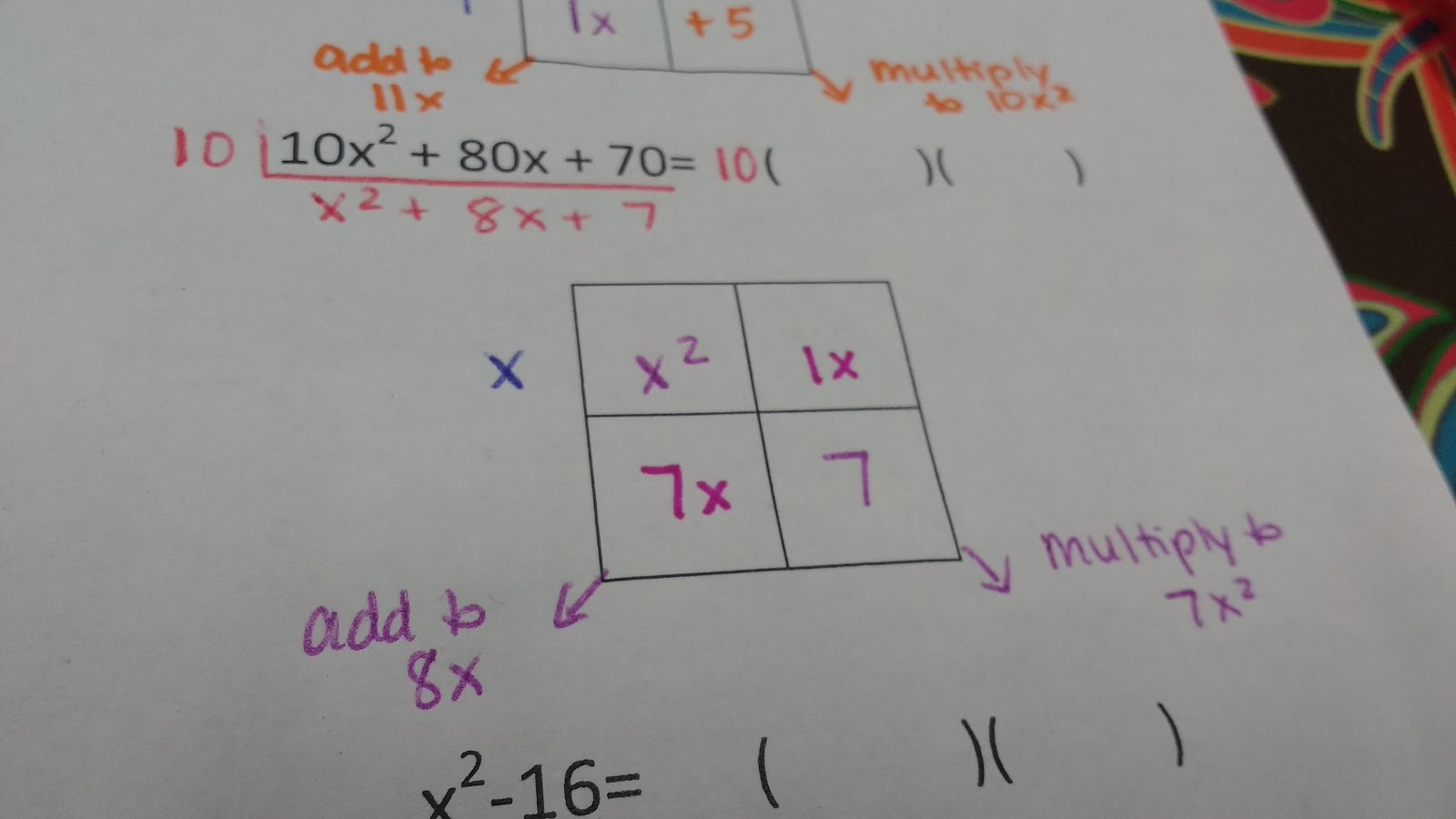
Similarly, the GCF of x^2 and 7x is x.

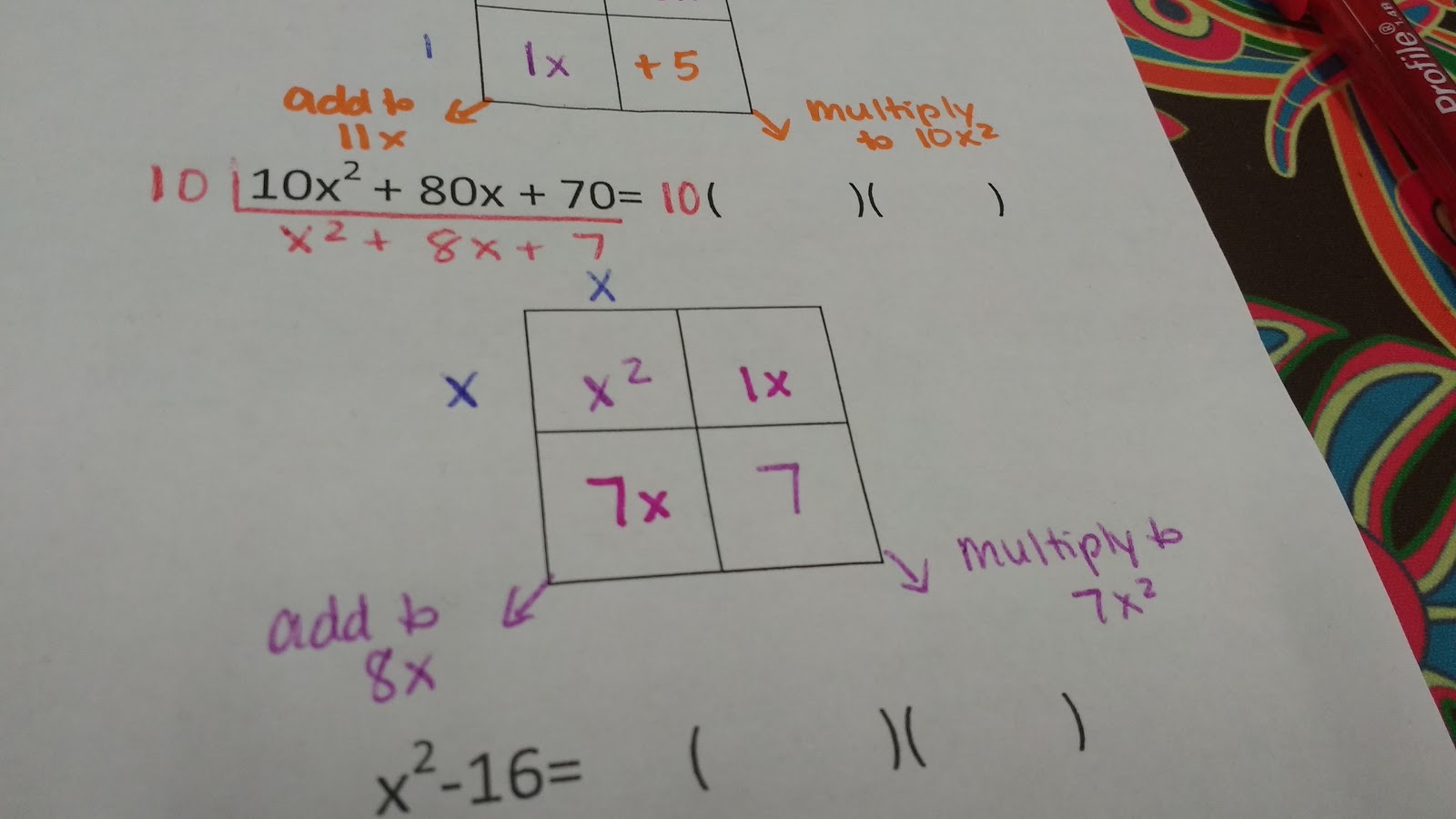
The GCF of 1x and 7 is 1.

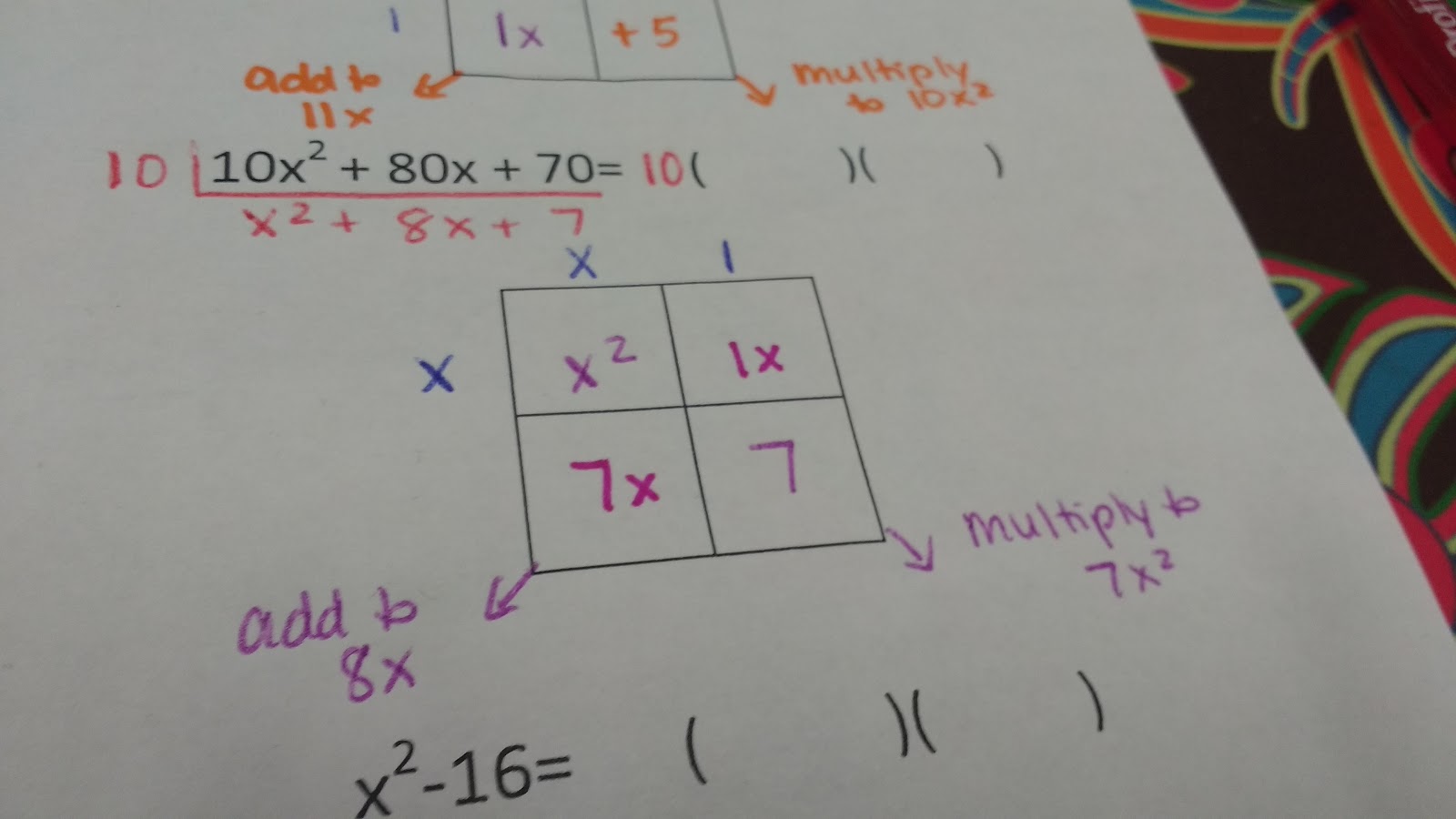
And, the GCF of 7x and 7 is 7.

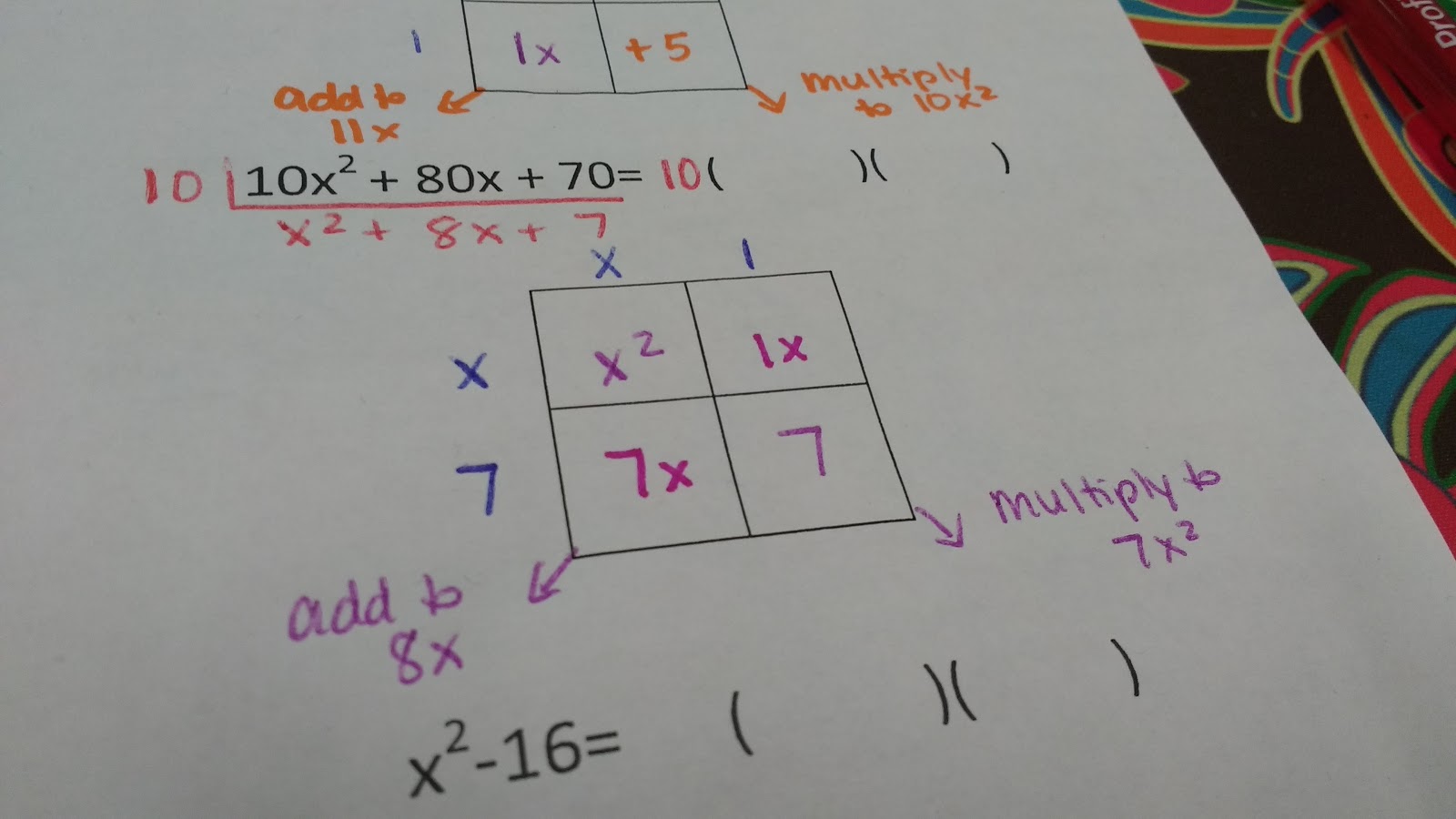
Now, I need to stop and double check that all of my multiplication works out correctly.  If it does, I’m ready to write my final answer.  The 10 is already written outside my parentheses.  The x and +1 from the top of the box go in one set of parentheses.  The x and +7 from the side of the box go in the other set of parentheses.

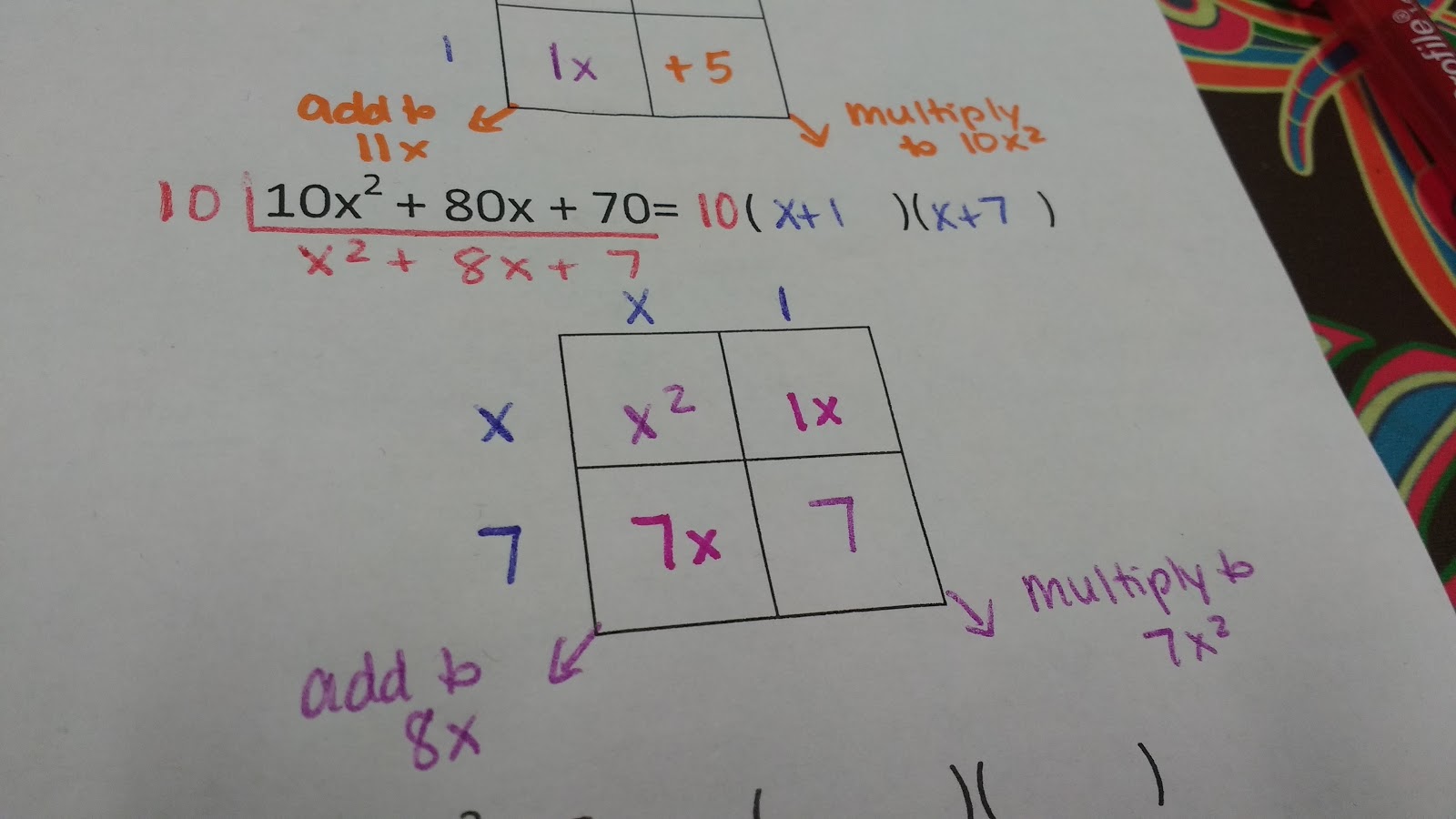
We’ve finished another problem!  I have one more example I want to show you.  I don’t teach factoring difference of squares as a separate topic.  I make my students use the exact same box method.  This keeps factoring the difference of squares from seeming like a “trick” to memorize.

x^2 – 16 can be rewritten as x^2 + 0x – 16.

There is no GCF to factor out.  So, I can write x^2 in the top left box and -16 in the bottom right box.

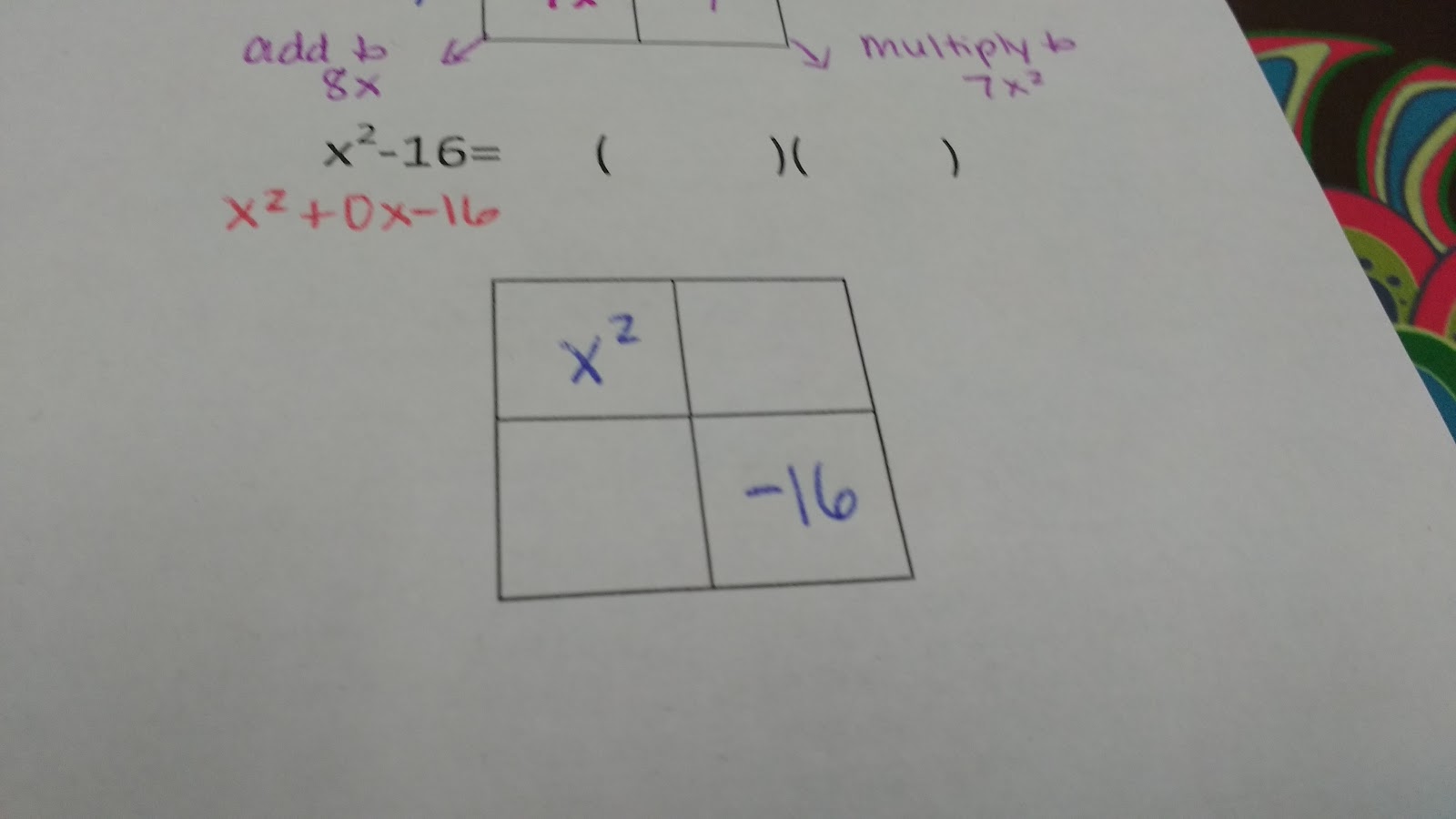
The middle 0x term tells me that my two missing boxes add to 0x.  At first, students ALWAYS think this is impossible.

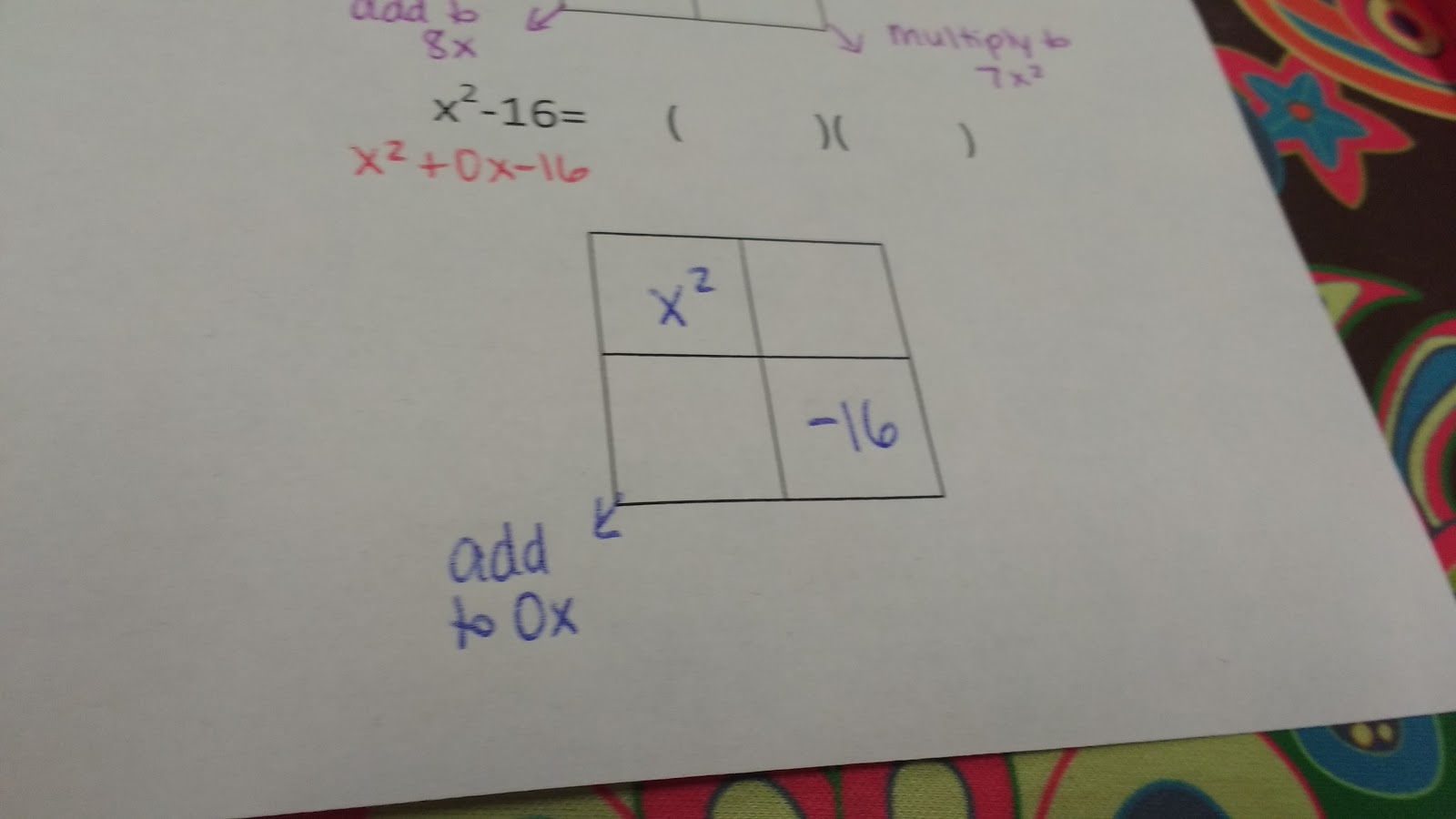
The two boxes that are given multiply to the same value as the two boxes that are missing.  So, I know I am looking for two terms that add to 0x and multiply to -16x^2.

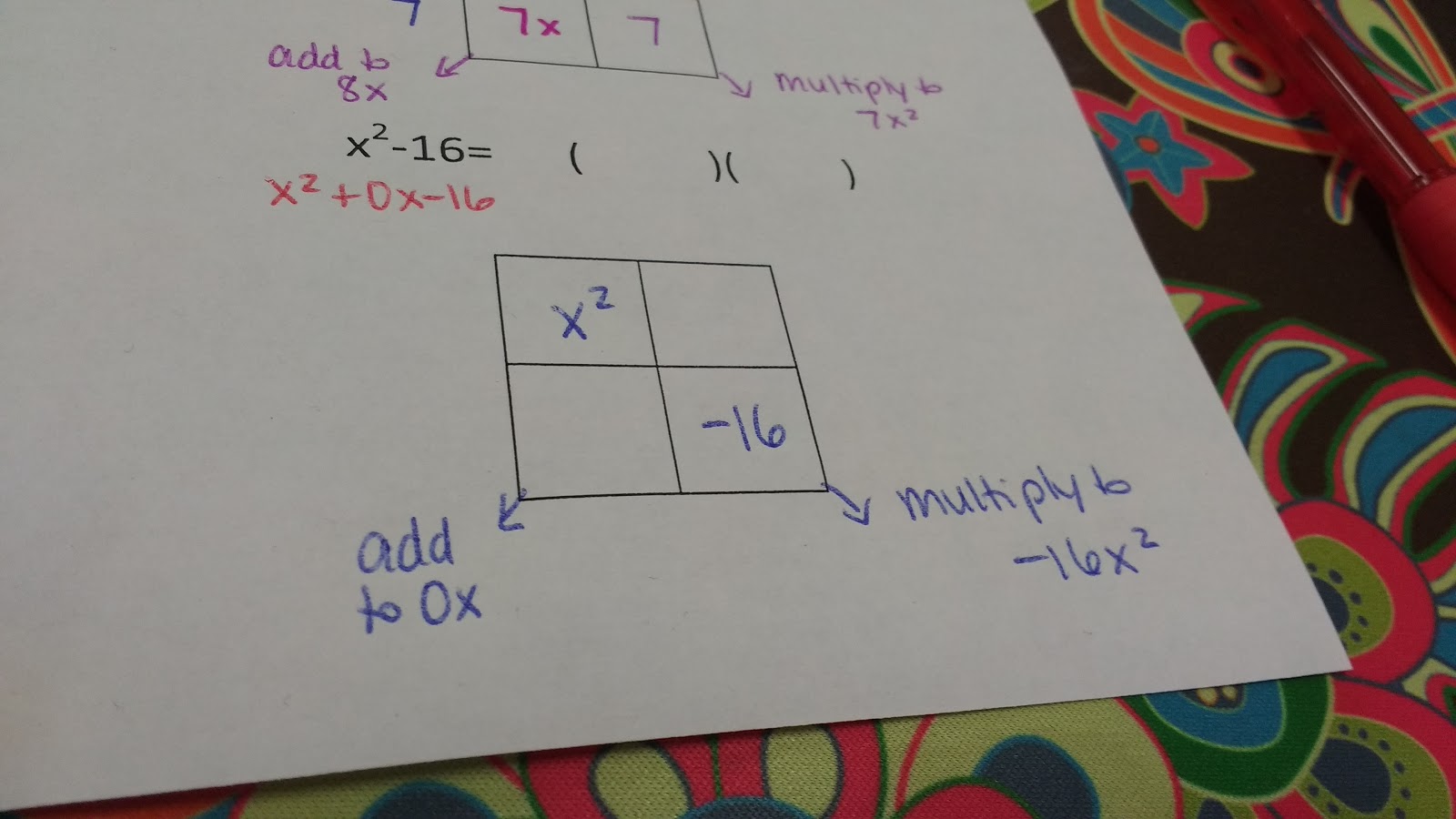
Those two terms would be -4x and +4x.  So, I need to write those in my empty boxes.

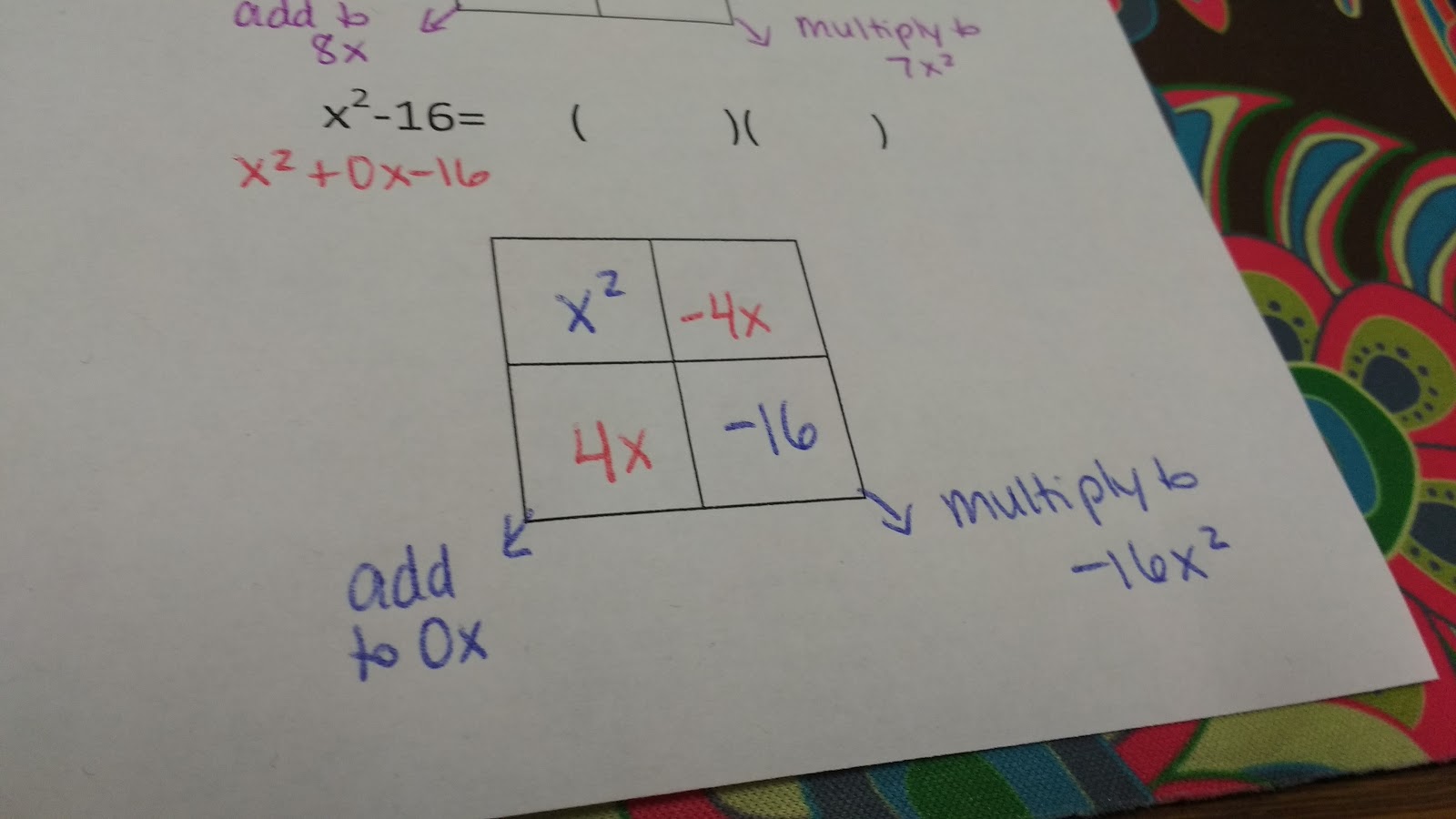
x^2 and -4x have a GCF of x.

x^2 and 4x also have a GCF of x.

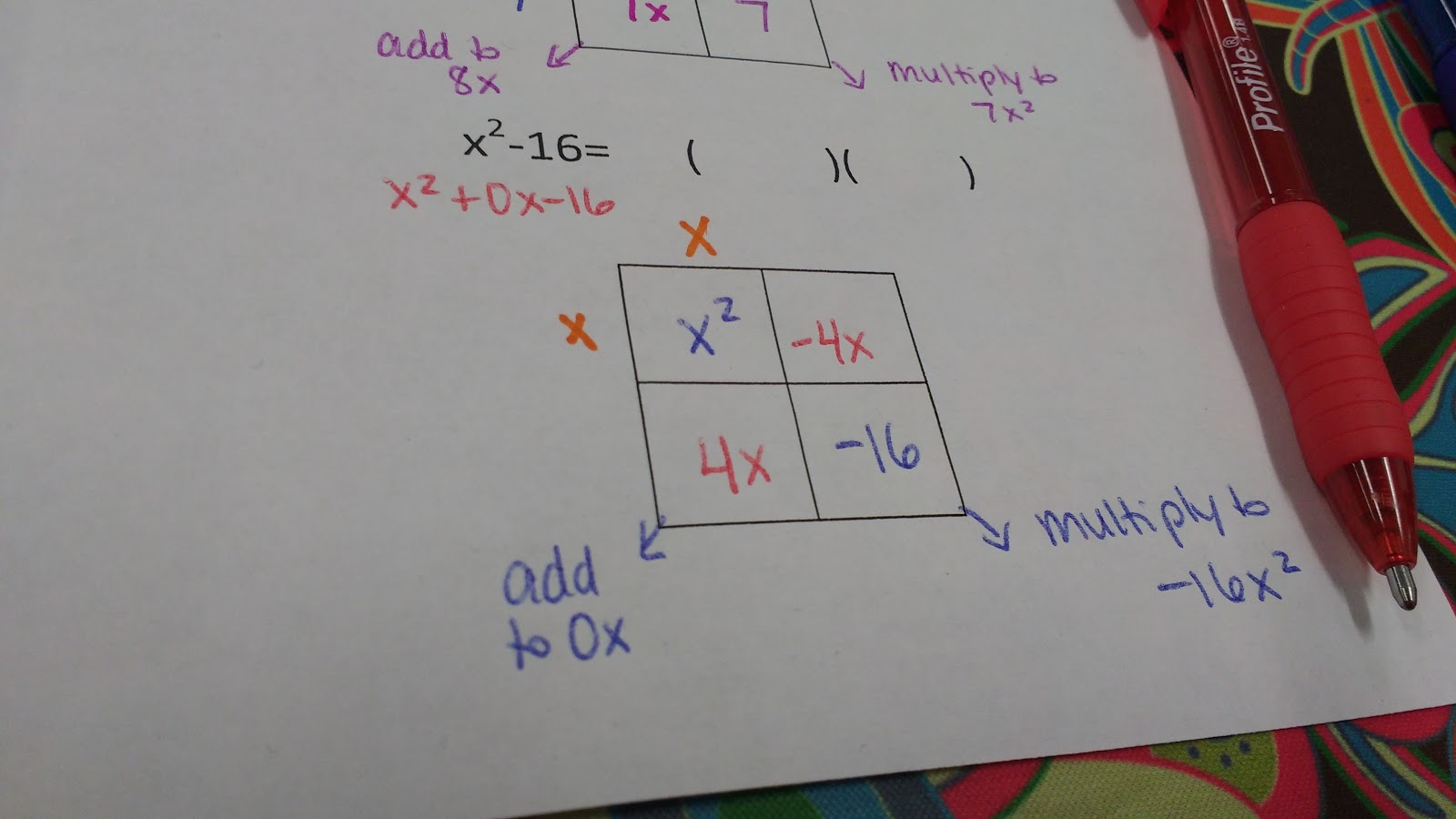
The GCF of -4x and -16 is -4.

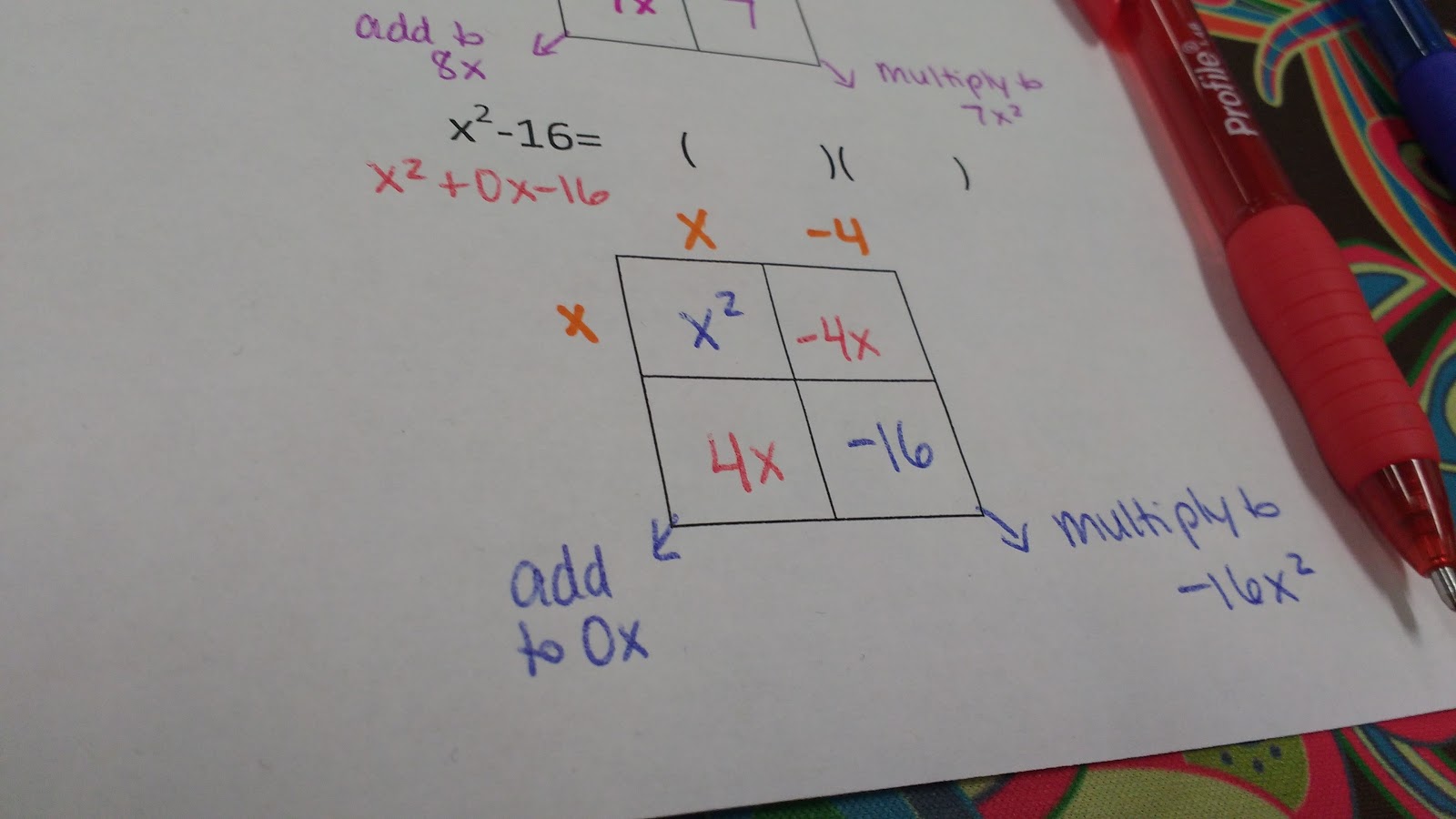
Meanwhile, the GCF of x^2 and 4x is 4.

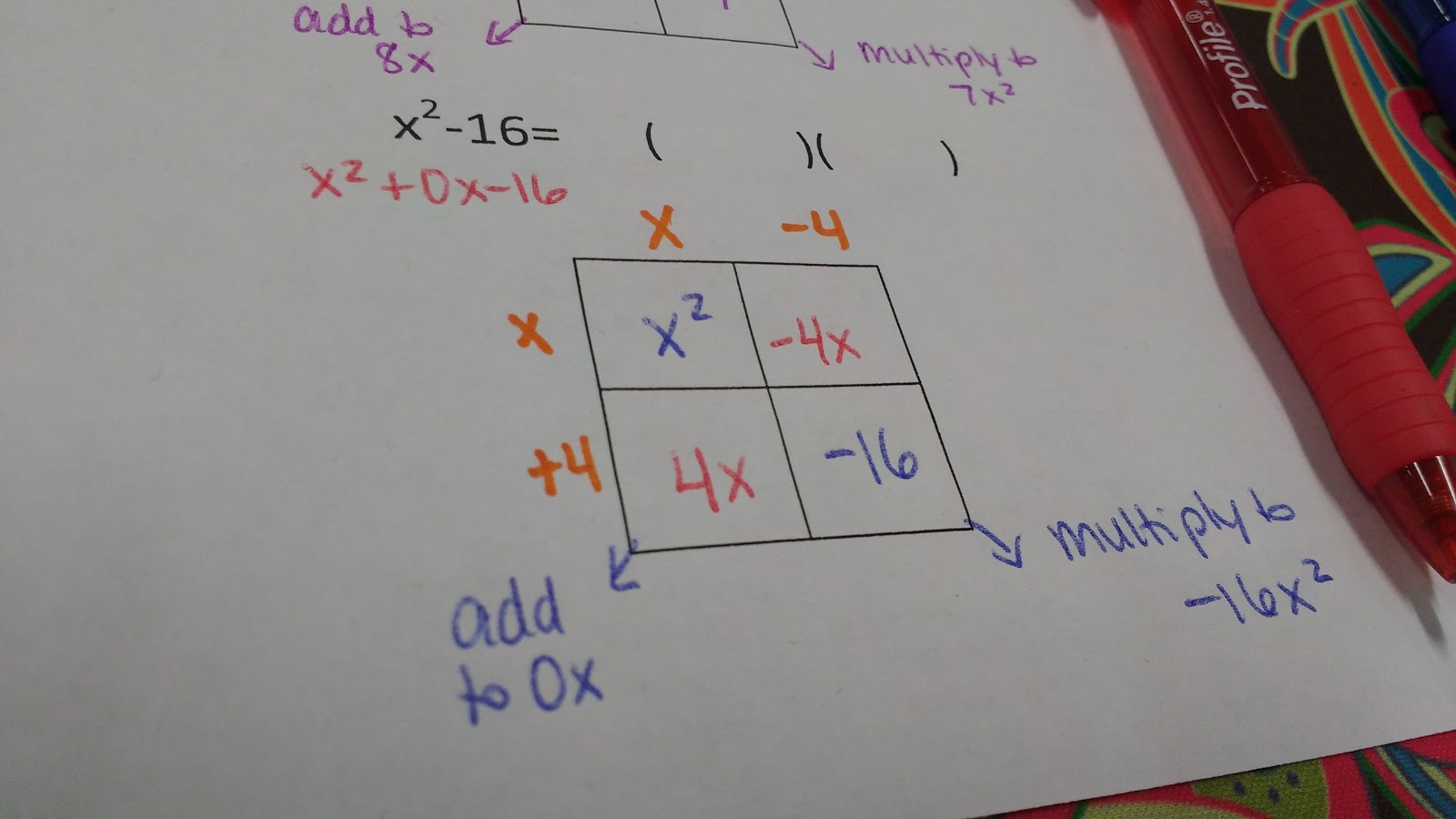
So, I can write my answer using the x +4 from the side and the x -4 from the top.  x^2 – 16 is the same as (x+4)(x-4).

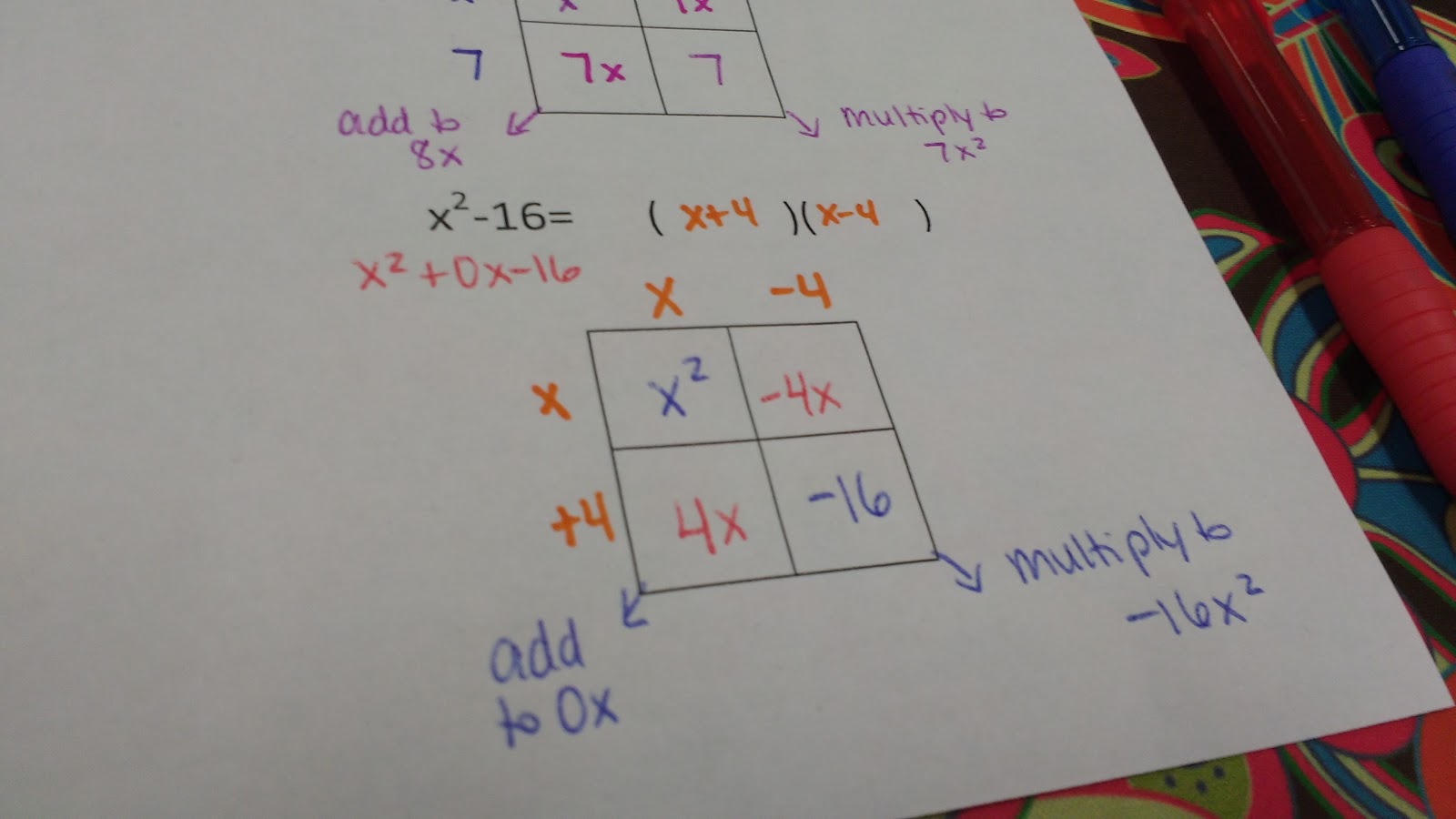
And, that’s the beautiful box method.  I hope this step-by-step explanation has helped clear up any questions you might have had about how the box method works.  If you still have questions, please leave them in the comments!

Want to give students some practice factoring? Check out this factoring puzzle.

More Resources for Teaching Quadratics

Sarah Carter teaches high school math in her hometown of Coweta, Oklahoma. She currently teaches AP Precalculus, AP Calculus AB, and Statistics. She is passionate about sharing creative and hands-on teaching ideas with math teachers around the world through her blog, Math = Love.

Similar Posts

13 Comments

  1. I started using the box method after you first shared it (last year? two years ago?) and I absolutely love it. It was very similar to the way I had taught factoring, but I incorporated it at the same time as multiplying with the box method. It made everything so much more organized and easy to follow. But I love the addition of writing outside the corners what needs to add and what needs to multiply. I had always SAID this, but I never wrote it. Why? No clue. It seems so simple. So thanks for sharing this so I could gather that one more tip. Again, I love boxes!!!

  2. Well an alternate method to the grid is to use the harbour bridge method

    looking at a quadratic ax^2 + bx + c

    multiply a and c find the factors that add to b

    so in your example

    2x^2 + 11x + 5 2 x 5 = 10 factors are 1 + 10

    so write it as

    (2x + 10)(2x + 1)
    ——————
    2

    now factor the binomials

    2(x + 5)(2x + 1)
    —————
    2

    rmove common factors from the numerator and denominator

    leaving

    (x + 5)(2x + 1)

    this method always works… and avoids guess and check….

    so looking at

    15 – x – 6x^2 same process 15 x -6 = -90

    since b = -1 the larger factor is negative -10 and 9

    (9 – 6x)(10 – 6x)
    ——————
    -6

    now factor the binomials

    3( 3 – 2x)(-2)(5 + 3x)
    ———————-
    -6

    remove common factors and you have (3 – 2x)(5 + 3x) as the solution.

    Hope it makes sense and gives you another method.
    (

    1. Another advantage of the harbour bridge method, which I use too, is that you don't need to complete the factorisation if you are finding the zeroes of the quadratic.

    2. I believe that this method fails when there is a common factor among all the terms that the students don't recognize. For example, 10x^2 + 80x + 70. If a student didn't recognize that there's a GCF (like many of mine don't), you would multiply 10*70 = 700 and then find two numbers that multiply to 700 and add to 80. This would be 70 and 10. Writing (10x^2 + 70)(x^2 + 10) all over 10.
      Then you would write it as 10(x^2 + 7)(x^2 + 10) all over ten and leaves (x^2 + 7)(x^2 + 10) as the final answer, which would be incomplete.
      Am I missing something here?

      1. If you don’t factor the GCF, you should get a box like this:
        10x 70
        ________________
        x | 10x^2 | 70x |
        ________________
        1 | 10 x | 70 |
        ________________

        You then get (10x +70)(x+1). You can factor the ten out of the first expression to give you 10 (x+7) (x+1). It is messy, but still works.

  3. LOVE! I start my unit on polynomials and factoring this week! This will be so helpful! I have used this in the past, but your explanation and examples are so easy to follow! Can I use your pics in my powerpoint when I teach this?

    Also, I know it works because I had a student come to me to get some help. I taught him algebra 2 years ago, and he needed help with factoring…..He remembered that we used the box method.

  4. Thanks so much for this post today Sarah! I am teaching factoring this week and this post is SO CLEAR and easy to understand. I love the box method and my students last year really responded to it for multiplying polynomials, but we didn't use it as much for factoring. I am so excited to share it with them this year with my new clarity!

  5. Love it but I think it's interesting how your pics get closer and closer to what looks like you're bed as you're working more problems. I hope you didn't stay up too late doing this!

    1. It's actually not my bed. 🙂 The background is a mouse pad/keyboard pad that sits on my desk at school. Definitely guilty of staying up late blogging, though!

  7. what happens when students want to place the terms in different order? like the 10x on your first example on the lower left-hand box?

Comments are closed.