|

Guest Post: Solving Absolute Value Equations with a Little Creativity

Good morning!  Today, I’m happy to share a guest post with you from Beaura Cavalier. She is a high school math teacher in Arkansas, and I am so excited to share with you what she has to say about teaching students to solve absolute value equations with a little creativity.  I’ll let her take it away!  

I was trying to find a way to help my Algebra 1 students remember how to do Absolute Value Equations. During a class, I came up with the following analogy.

Okay, so here is the problem.

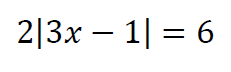
absolute value equation 2|3x-1|=6.

The prisoners (3x – 1) are in jail (absolute value bars).

They want to get out of jail, but first they have to wait until their visitors (any number outside of the absolute value bars) leave. So, add, subtract, multiply, or divide (on both sides of the equation) to get their visitors to leave.

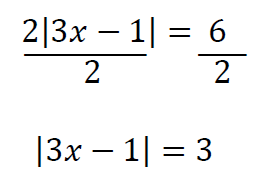
process of solving absolute value equation.

Now that you have the prisoners all by themselves (in their jail cell) on one side of the equal sign, they prisoners can get parole!

Once they are released from jail, they have two options. They can choose to be good (equal to the positive of whatever is on the other side of the equal sign) or they can choose to be bad (equal to the negative of what is on the other side of the equal sign).

two linear equations.

 Then solve both equations to get two values for x.

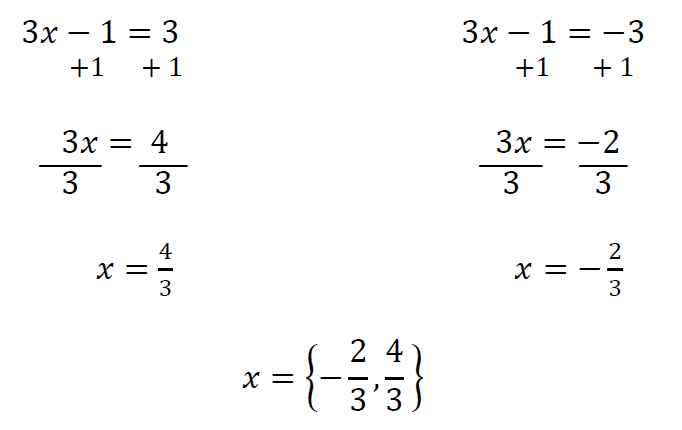
process of solving 2 linear equations.

The students laughed at my ideas, but now they are quick to point out that the prisoners have the choice of being bad or good, so x has two values!

Thank you so much Beaura for sharing a bit of your classroom with us!  My leveled practice cards for solving absolute value equations would be the perfect practice opportunity to go along with this lesson.

More Activities for Teaching Absolute Value Equations and Inequalities

Sarah Carter teaches high school math in her hometown of Coweta, Oklahoma. She currently teaches AP Precalculus, AP Calculus AB, and Statistics. She is passionate about sharing creative and hands-on teaching ideas with math teachers around the world through her blog, Math = Love.

Similar Posts

11 Comments

  1. LOVE this! Big props to you, Beaura! I am going to use it next month when we get to abs value functions. Thank you!
    -snapdragon

  2. Sarah – thanks again for all you do on your blog. I'm wondering about this idea of explaining something mathematical in a way that doesn't use mathematics. In the elementary school building I am in, I frequently have conversations with the reading specialist about things she hears teachers say to students about reading. For example she will hear teachers say "We have to read every word in the sentence because words that don't get read have their feelings hurt." Firstly, words do not have feelings, and in reality, we have to read every word in the sentence because voice-print match is a really important skill that kids need to have. So, I need to say sort of the same thing about Beaura's post. If we want to build mathematical understanding in students, we have to do it through mathematics. Mathematical thinking can only be developed through thinking about mathematics. As soon as it becomes about something else (jails and prisoners) then it isn't math. Perhaps an alternative, in this case, would be to use some sort of graphing technology to examine some absolute value graphs and build some mental velcro for students around what the graphs of absolute value functions look like. Eventually we might ask students when (and if) a particular graph has a particular y value. It will become clear that some of those situations have no solution, some have one, and some have two. Exploring each of those situations graphically and eventually pivoting to the algebraic solution might paint a picture that is mathematically sound and robust. It's hard to "unsee" an absolute value graph with a horizontal line cutting through it in two places, or no places. It might extend any study of transformations of graphs that we have started or it might help lay the groundwork for solving quadratic equations. I'm curious what your other readers think.

    3. Aaron, For many of my students, mathematical thinking is a struggle. Just as math teachers use "Please Excuse My Dear Aunt Sally" to help students remember the Order of Operations, my prisoner description is just a memory tool to help them remember the order of the steps to solve absolute value equations.

    4. @Beaura I would write a similar reply regarding teacher use of PEMDAS. I would point readers to the article "Questioning the Order of Operations" in Mathematics Teaching In The Middle School, October 2016. MTMS has additional articles on this topic from March of 2011, December of 2002. Teaching Children Mathematics has articles from February 2012 and August 2015 specifically on PEMDAS and more generally on forgoing rule, memory driven mathematics instruction. I'd be happy to provide PDF's of these articles (just don't tell NCTM).

      We need to get away from the idea that math is about rules and remembering stuff. It isn't. It's about structures and strategies and flexible thinking. In what ways can our instruction engender flexible strategic thinking rather than an adherence to rules, mnemonics, and organizational tools?

  3. I have a comparable one I've used for middle-schoolers since a class first sniggered: when things cuddle up, they multiply.

    1. I use that too! And then I tell them that the product is their 'maths baby'. So many groans, so much remembering.

  4. Love this! I have a similar jail story that I use when simplifying radicals! Thanks for the inspiration!

Comments are closed.