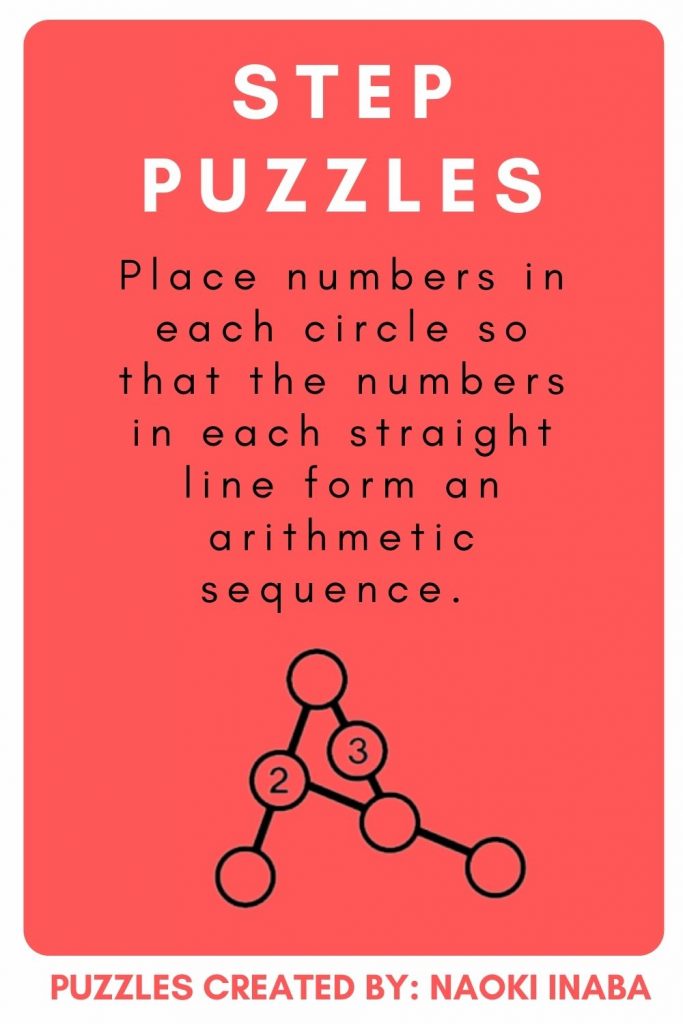
Step Puzzles (Arithmetic Sequence Puzzles)

You’ll need some logical thinking in order to fill in the missing circles on these step puzzles from Naoki Inaba so the numbers on each line form an arithmetic sequence.

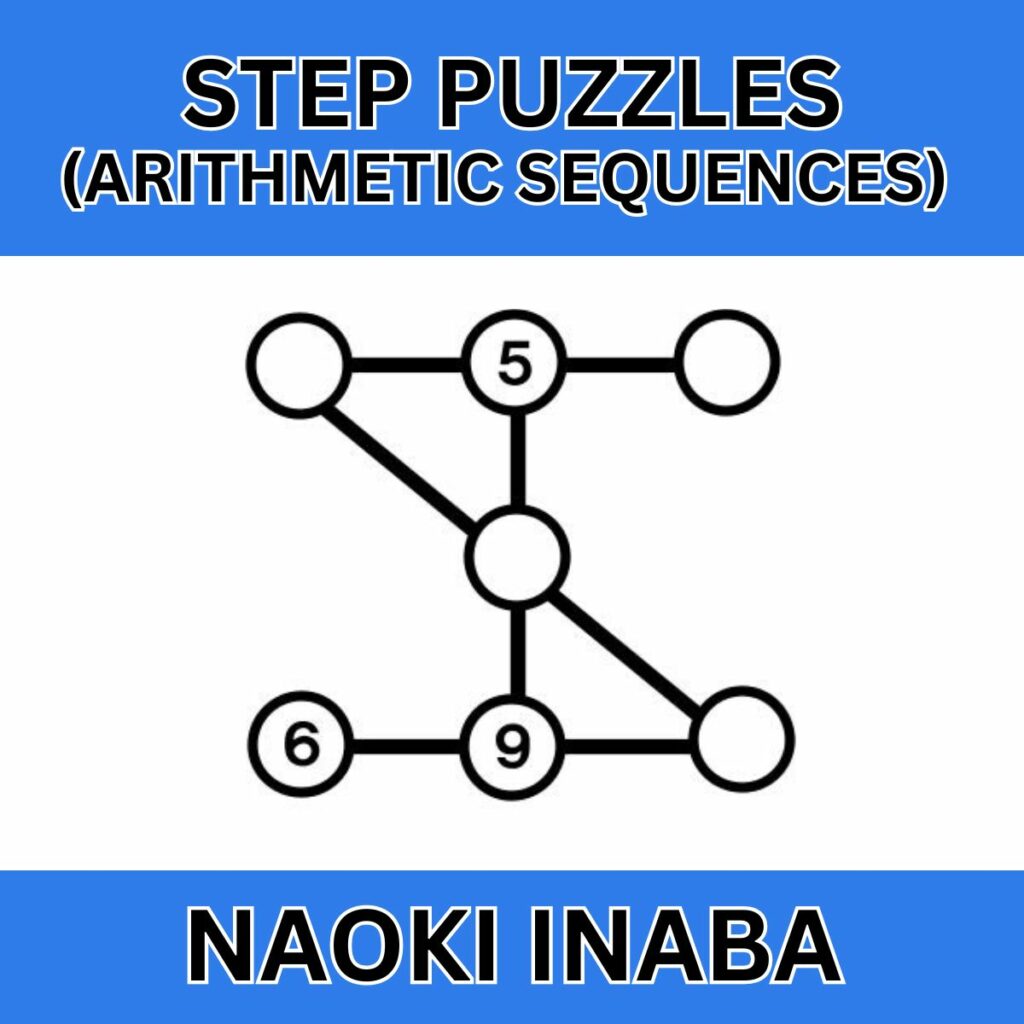
Puzzle Source

These step puzzles are the creation of Naoki Inaba, a prolific logic puzzle creator from Japan. I was first introduced to Inaba and his puzzles in 2016 when I discovered his area maze puzzles.

Wanting even more puzzles, I found myself on his website (written entirely in Japanese). Since then, I have translated many of his puzzles to English for use in my classroom and yours!

What are Step Puzzles?

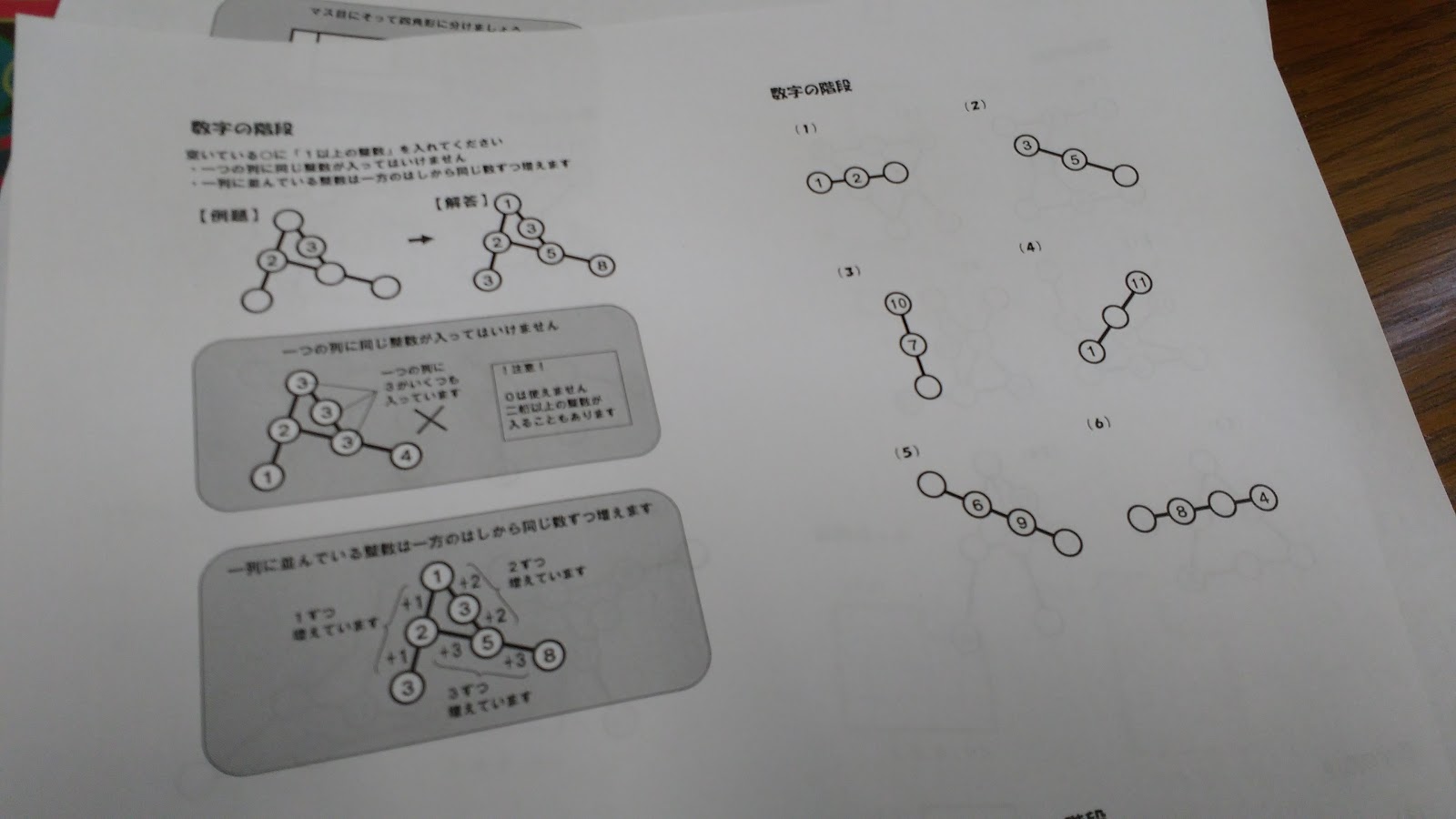
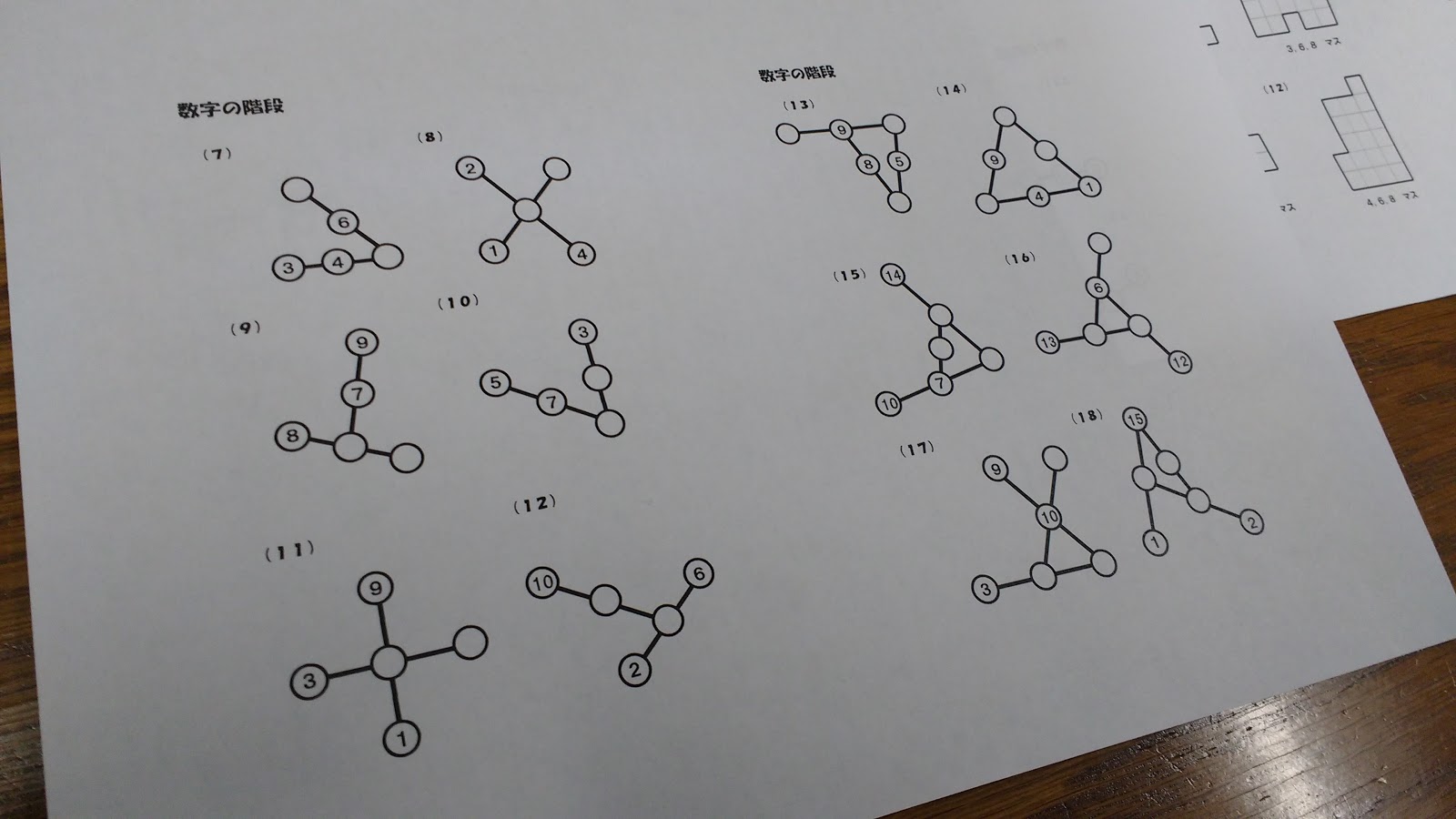
Step Puzzles involve finding numbers to place in the empty circles so that the numbers along each line form an arithmetic sequence.

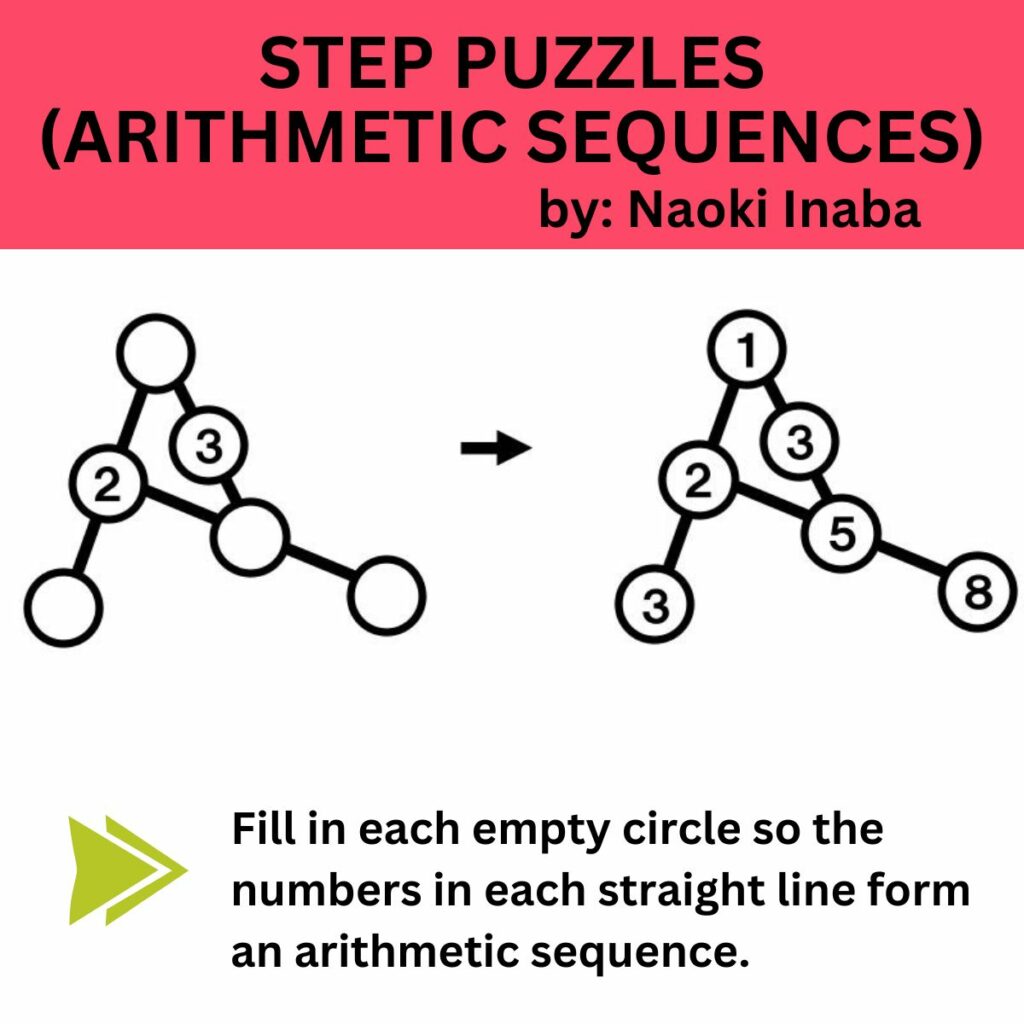
I like to introduce step puzzles to students by showing them a puzzle and its solution and asking them to figure out the rules of the puzzle.

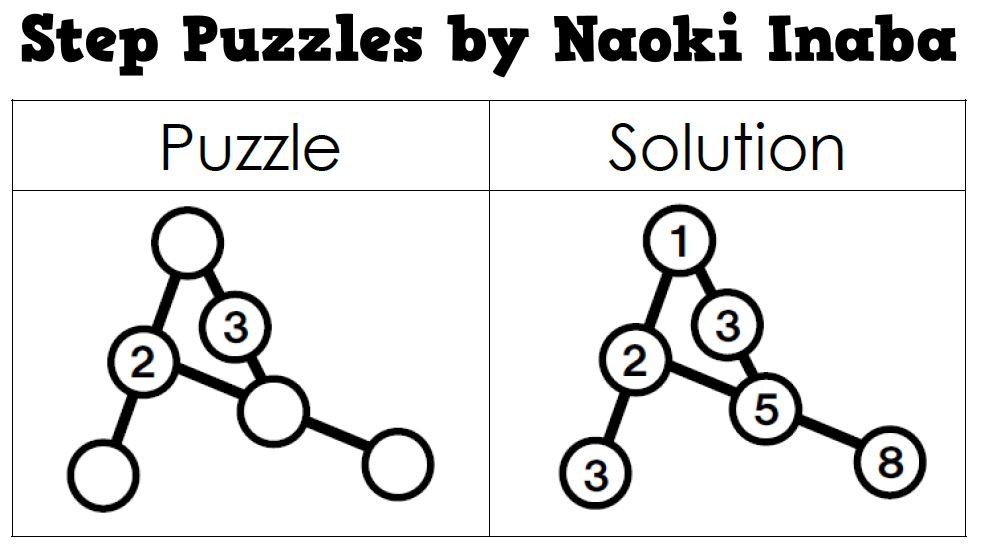
Because of this problem-solving based introduction to step puzzles, I have not typed out the instructions on the puzzle sheet that I give to students.

I have another reason for this. These puzzles are accessible for students as young as elementary school. They are still engaging for middle school and high school students.

But, with these age groups, students can be instructed to place numbers in each circle so that each line forms an “arithmetic sequence.” This proper vocabulary would likely intimidate an elementary student who could easily tackle these puzzles with an age appropriate introduction.

The puzzles start out quite simply. 

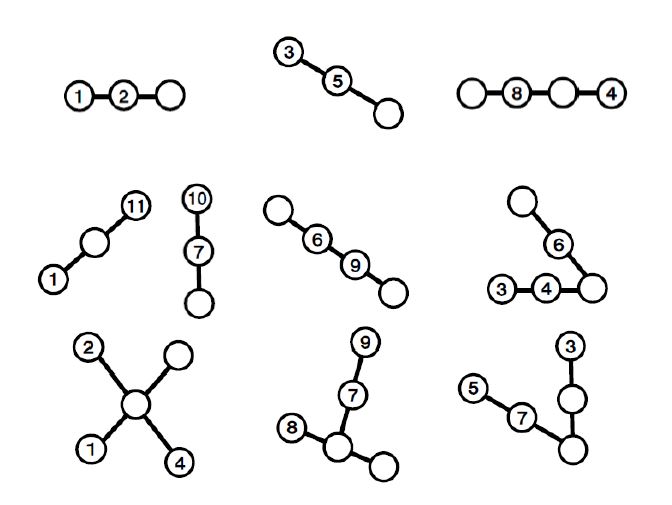
Sometimes my students have used the first puzzle to make an incorrect assumption about how the puzzles work. They assume that 1, 2, 3 is the answer to the first puzzle because 1 + 2 = 3. When really, it is 1, 2, 3 because 1 + 1 = 2 and 2 + 1 = 3.

You can check student understanding of this by having them solve the second step puzzle 3, 5, ___. The correct answer is 7. Students with the prior misunderstanding would answer 8.

I’ve found that once I set students straight from this misunderstanding that they seem to be good to start tackling the puzzles on their own.

The puzzles quickly progress in difficulty. Soon students have to start figuring out where to start solving the puzzle. If students start in the wrong place, the puzzle will seem impossible.

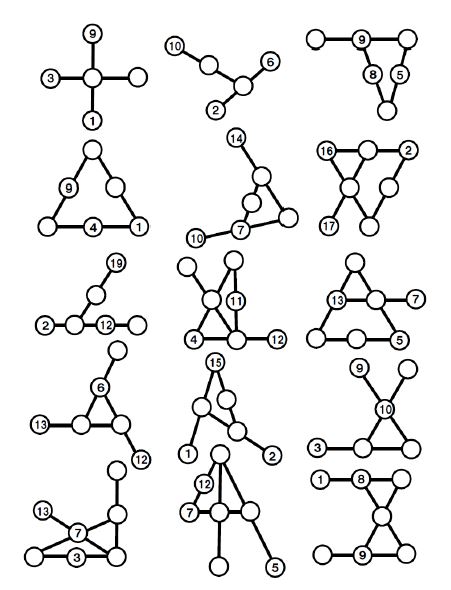
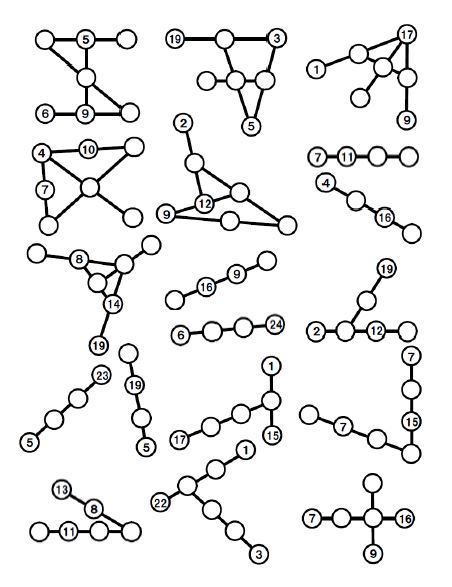
Step Puzzle Variations

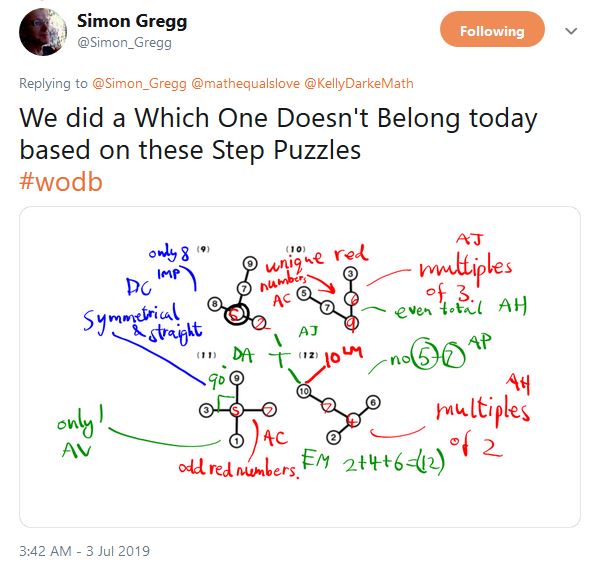
Step Puzzle WODB Puzzle

Check out how Simon Gregg combined WODB (Which One Doesn’t Belong) with Step Puzzles!

Pin for Later

Not ready to use these puzzles in your classroom just yet? Pin them for later on pinterest!