Notable Numbers – A Math Teachers’ Circle Session on Happy Numbers and Friedman Numbers

Earlier this week, I led a session called “Notable Numbers” for the Central Oklahoma Math Teachers’ Circle. I’ve been a member of the Tulsa Math Teachers’ Circle for over five years now. Sadly, due to COVID, we haven’t met for the past year. The Central OK Math Teachers’ Circle is currently meeting virtually via Webex, so this was an entirely new experience for me.

When I was first approached about leading a session, I definitely battled with a bit of imposter syndrome. I knew I was qualified to lead, but I also had set through so many Math Teachers’ Circle sessions over the years that were led by PhDs and people way more qualified than I will ever be. I decided to go for it and agree before my brain had a chance to talk me out of it. I’m so glad I did!

It took me several weeks to come up with a session. My problem wasn’t that I didn’t have any good ideas but that I had too many ideas. I wanted to do ALL the math and ALL the puzzles, but you can only fit so much in an hour and fifteen minute session. I eventually chose two topics that I wanted to introduce the math teacher participants to: Friedman numbers and happy numbers.

I didn’t want any of the participants to google the topics beforehand (I was hoping to introduce them to some areas of math that they were hopefully not familiar with yet), so I intentionally gave my session a vague title: “Notable Numbers.”

I’m purposefully writing this post in such a way that you can play along. I’m doing my best to avoid giving away too much of the mathematical fun that is to be had. So, if you’re looking for answers, you are out of luck!

Opening Puzzle

We kicked off the session with an opening puzzle. I’ve shared this Perfect Square Puzzle before on the blog. The task is to arrange the numbers from 1 to 15 in a row so that the sum of any two adjacent numbers is a perfect square. I recently saw this puzzle featured in a puzzle book, and they called they puzzle “Square Pairs.” I really liked that name!

If I was leading this session in person, I would have provided sets of laminated cards to each group to manipulate. Since this was a virtual session, I gave participants two options. I sent out a set of cards with the numbers 1-15 beforehand. I thought some of them might want to cut the cards apart so they could move them around. I also sent out a google slides version of the task in case people wanted to virtually manipulate the cards.

The task wasn’t as successful in a virtual setting as I would have hoped because I couldn’t really see how the participants were progressing. This made timing my opening activity a bit trickier. In an in-person meeting setting, I would have this opening puzzle posted before participants entered the room so they could tackle the puzzle at their own leisure before the session started.

I briefly introduced myself to the participants. I told them about the classes I teach as well as my love for puzzles and blogging/tweeting about math.

Next, I kicked things off by putting the number 13 on the screen. I asked the participants to type in the chat any types of numbers that 13 belongs to. I pretty much got the responses I expected: odd, prime, dozen + 1, unlucky, etc.

Friedman Numbers

Our first main notable number of the night: Friedman Numbers.

Before sharing the definition of a Friedman number, I explained that the numbers were named in honor of Dr. Erich Friedman, a retired professor of mathematics at Stetson University. I recommended that the participants check out Dr. Friedman’s website – especially his Puzzle Palace.

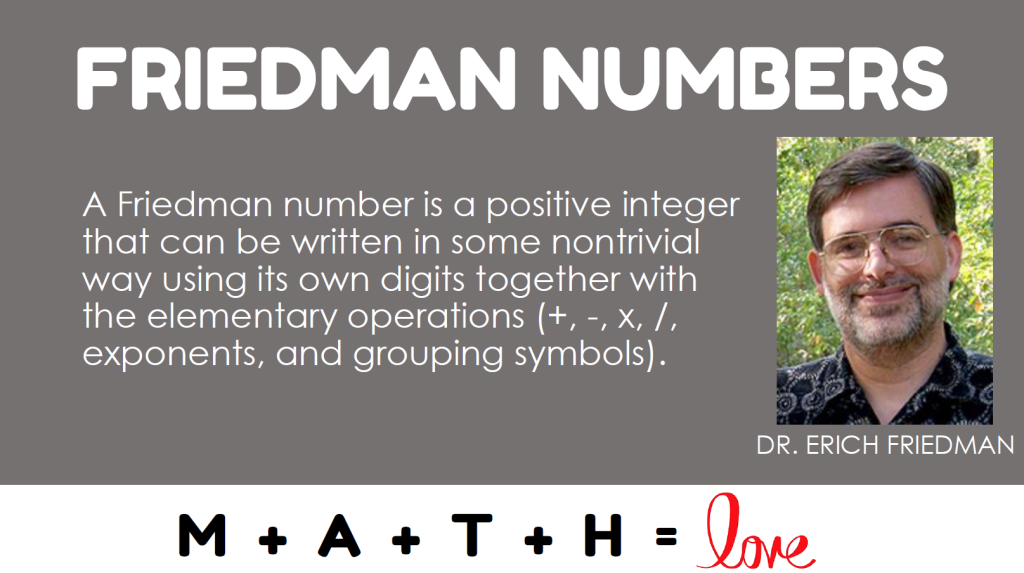
What is a Friedman number?

So, what is a Friedman number? A Friedman number is a positive integer that can be written in some nontrivial way using its own digits together with the elementary operations (add, subtract, multiply, divide, exponents, and grouping symbols.)

Since we’d just discussed the number 13, I posed the question: Is 13 a Friedman number?

We ran through the different mathematical operations that we could apply to one and three to see if we could arrive at a result of 13.

1 + 3. No.
1 – 3. Still no.
3 – 1. Nope.
1 * 3. I don’t think so.
1 ^ 3 or 3^1. Not at all.

Grouping symbols aren’t going to help us since we only have two digits, so I think we have exhaustively proven that 13 is not a Friedman number.

Next, I revealed this fact: There is only one Friedman number under 100.

Originally, my plan was to just go ahead and reveal the only Friedman number under 100. (SPOILER ALERT: Don’t scroll if you want to try to figure it out yourself!) But, I decided in the moment to see if the group could figure out the only Friedman number under 100 themselves before I revealed it.

This was when I realized based on some of the participants’ comments that there was still some confusion about the definition of a Friedman number. This was confusion I had caused myself since I hadn’t yet provided them with an example of a Friedman number. I re-explained the concept, and invited them to spend a minute or so hunting for the only two digit Friedman number.

One participant excitedly shared 25 in the chat. There was visible applause from some of the other participants. This was a fun group!

25 is a Friedman number because the digits in 25 can be rearranged into 5^2 which evaluates to 25.

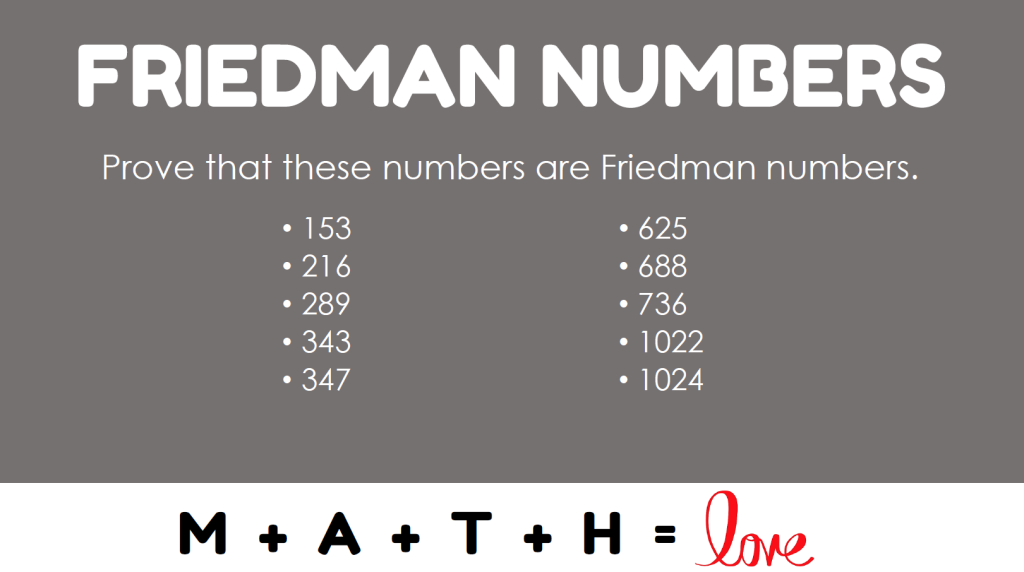
Next, I tasked them with finding the next five Friedman numbers. Even though there is only one two-digit Friedman number, there are five Friedman numbers in the 120s. Can you find all five?

Since there are only 10 numbers in the 120s, participants have a 50% change of picking a Friedman number when they choose a number to try to solve. Participants shared out solutions as they found them. It took us a bit longer than I expected, but we managed to find all five. One participant actually accidentally found that the number 216 was a Friedman number while working on the 120s task.

I provided participants with the next 10 Friedman numbers for them to puzzle through on their own. We didn’t have enough time to tackle these during the session.

I also wanted to take the opportunity to expose the participants to several lovely extensions to Friedman numbers.

Nice Friedman Numbers

Nice Friedman numbers are Friedman numbers in which the digits in the expression can be arranged to be in the same order as in the number itself.

Roman Numerals

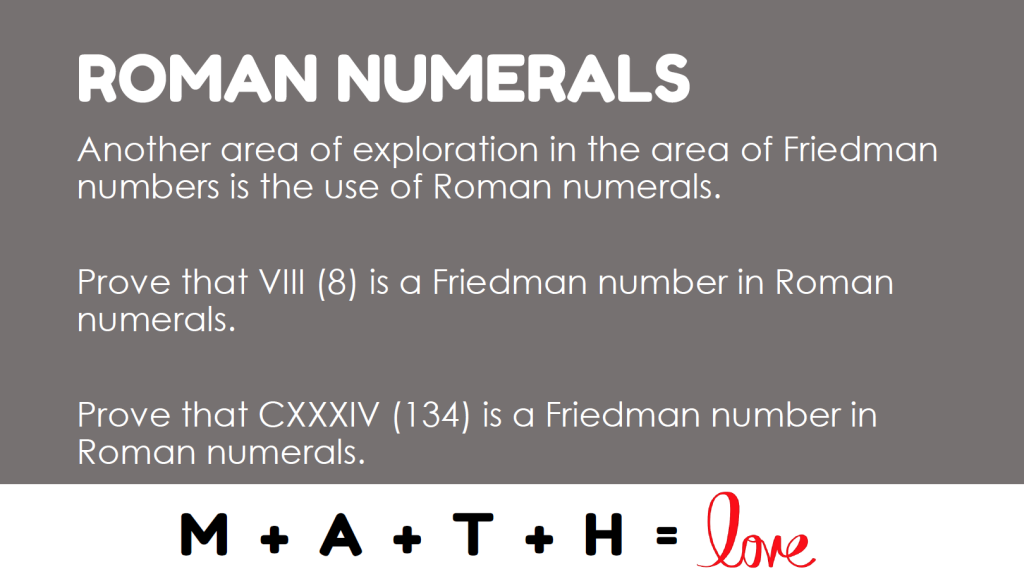
We also discussed Friedman numbers in Roman Numerals.

As a group, we found two different proofs that VIII is a Friedman number in Roman numerals. I was shocked when one participant found a proof that was different than the one I had come up with when I was preparing the session. I left proving that CXXXIV is a Friedman number as an extension task for the participants to complete at home if they wished.

Sources for Friedman Numbers

I provided everyone with sources to read up more on Friedman numbers if they wished.

Here are the links to the sources if you are interested in reading more!

Number Freak: from 1 to 200: The Hidden Language of Numbers Revealed by Derrick Niederman (Penguin, 2009)

NCTM Illuminations Brainteaser

Erich Friedman’s Problem of the Month (August 2000)

Happy Numbers

Next, we shifted our gears to our second notable number of the night – happy numbers.

What is a happy number?

Happy numbers are an interesting breed of number. In all my readings, I could not find a written definition of happy numbers. Instead, happy numbers are always presented as an algorithm to follow.

Pick a number.
Square each digit and add the result together.
Use the new number and do the same again.
If you reach 1, you have a HAPPY NUMBER.
If you never reach 1, you have a SAD NUMBER.

Like before, I used 13 as my example. Is 13 a happy number?

I was pretty happy with how this graphic I made in PowerPoint for illustrating that 13 is a happy number came out.

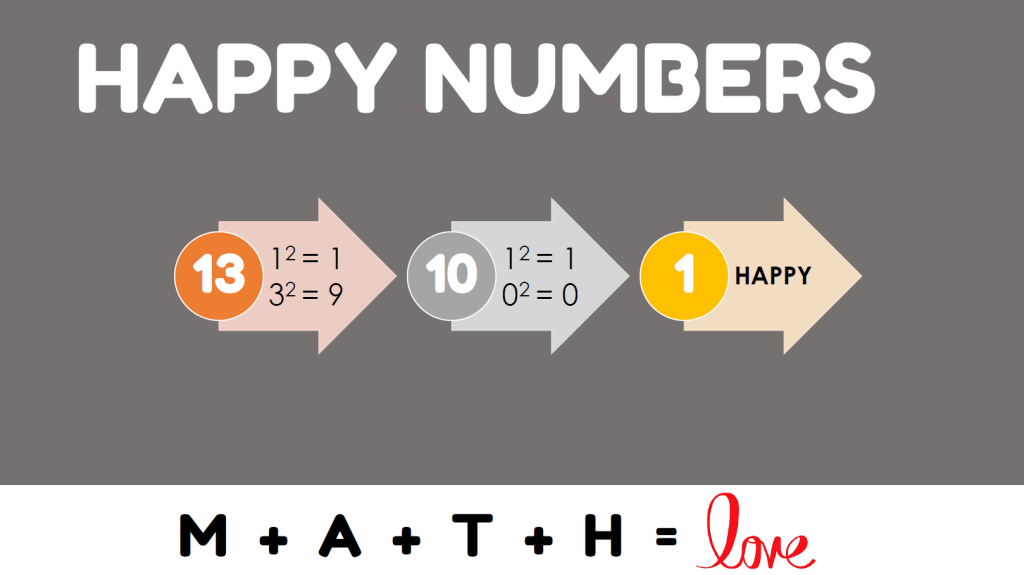
We worked together to answer the next two questions about happy numbers. My purpose in asking these two questions was to make sure everyone had a strong understanding of happy numbers versus sad numbers before breaking off into breakout rooms.

What is the smallest sad number?

What is the smallest happy prime number?

In breakout rooms, participants were tasked with asking this question: What percent of the numbers 1-100 are happy? Just before they were released to the breakout rooms, I asked them to make a prediction of the percentage. The groups guesses ranged from 15-20%.

Participants had been given a hundred chart to print out before the session to help them organize their working.

I left participants in the breakout rooms until there was about 10 minutes left of the session. When they returned from the breakout rooms, there was excitement in the air. I jokingly asked if anyone hated me for posing this task. They immediately started to say how thankful they were that I gave them enough time to really get in and explore and discuss in the breakout rooms. I’m not sure exactly how much time they were in the breakout rooms (maybe 15 minutes max?).

I definitely could have made the entire session about just Friedman numbers or just happy numbers. I intentionally chose to include both in case any of the participants had already heard of one of them. I really wanted them to play with a new mathematical concept. If I were to lead this session again, I think I probably would just pick one and only topic. Though, a longer session time would also help with this issue…

Many of the breakout rooms had started making some great discoveries in the last few minutes they were together that they were excited to share. I am intentionally not writing about these discoveries here because I want to invite you to play around with happy numbers yourself. Grab yourself a hundred chart and start classifying numbers. There are many discoveries to be had! It is not as tedious of a task as it may first seem. And, you definitely don’t have to check all 100 numbers to see if they are happy or sad.

Extension Tasks

I provided participants with the following seven extension tasks to play with at home as they continued to think about happy numbers.

• Is there an infinite number of happy numbers or is there a largest happy number?
• What is the smallest integer greater than 1 that, when multiplied by any happy number yields another happy number?
• Are there any pairs of consecutive numbers that are both happy? Are there strings of more than two consecutive numbers that are happy?
• What digit does not show up in any of the happy numbers under 100? Why is this? What is the smallest happy number containing this digit?

• Does happiness depend on the base in which the number is written?
• What is the maximum number of steps it takes to determine if a number is happy or sad?
• Investigate what happens when you apply the steps but cube the numbers instead of squaring them. What name would you give this type of number?

Sources for Happy Numbers

I will share my sources for happy numbers below.

MathCounts Problem of the Week Archive

Fred and Amy’s Maths Shack

Numbers are Forever by Liz Strachan (Constable, 2014 – Pages 107-108)

There is an article about happy numbers in NCTM’s The Mathematics Teacher journal in November 1972 by Donald C. Duncan. In this article, they are referred to as “Happy Integers.” So far, this has been the earliest mention I have found in literature.

The 2006 article “Happy Lessons” by Luke Robinson in Mathematics Teaching is absolutely fabulous if you are able to get ahold of a copy. This article introduced me to an entirely different way of visualizing happy numbers.

I found one other mention of happy numbers in a 1976 book by Creative Publications called Computer Clippings by Stephen J. Rogowski. The book provides a set of computer programs written in BASIC and FORTRAN for use in the classroom to explore various math topics, including happy numbers.

Overall, it was a great session. I had a ton of fun leading it, and I took two types of numbers that I thought I knew about and learned so much more about them in the process of putting together this Math Teachers’ Circle session on them.

I think everyone left with plenty more math to explore which was my goal. A good math teachers’ circle session leaves you wanting more.