Hands-On Archaeology Geometry Activity

I got to experience this hands-on archaelogy geometry activity during the 5 glorious days I spent last week at NSU-BA as part of the Oklahoma Geometry and Algebra Project (OGAP).  So much fun!  So much learning!

On the first day of the workshop, I walked in the room, and my eyes were immediately drawn to the table along the wall that held the supplies we would be using for the week.  There were thermometers, bags of play sand, work gloves, protractors, compasses, rulers, and SO MUCH CANDY.  I was incredibly curious about how play sand was going to find its way into a math lesson.

Monday passed, and the play sand remained on the table.  Tuesday came and went without any activities involving sand.  Surely we would get to play with the sand on Wednesday!  Nope.  Finally, on Thursday, we entered the classroom to see that a metal bucket had been filled with the sand.

I doubt you know this (unless of course you’re my sister – Hi sis!), but I’ve always thought it would be so much fun to be an archaeologist.  I was that kid who used to wish for a metal detector.  Okay, I still wish I owned a metal detector.  My parents never did give into that request.  I’m pretty sure my mom didn’t want the yard full of holes.  I just think it would be so exhilarating to unearth ancient artifacts!  But, I would not make a good archaeologist.  I’d probably get fired for complaining too much.  You see, I don’t handle dirt well.  I don’t like getting dirty.  So, having a job that involves digging in the dirt probably wouldn’t be the best fit.  Plus, I hate, hate, hate hot weather.  Oklahoma summers can be awful, and the air conditioner is my best friend.  

Since I doubt I’ll ever get a chance to pursue archaeology, I was excited to get to try my hand at an archaeology dig during the workshop.  The workshop facilitators urged us to use caution in this activity to avoid injury.  One person per pair was instructed to come to the bucket, don a pair of work gloves, and use the Mickey Mouse/Cars trowels to unearth an ancient artifact to carry back to their desk and trace.  I actually let my partner do the digging so I could document the process with my camera.  After digging in the sand, my partner unearthed a shard of pottery.  It looked like it was once part of a plate.

Our task was to calculate how big the original plate was that our shard belonged to.  Then, we were to compare the measurement we found to the other groups in the hope that some of our shards might fit together to form a more complete artifact.

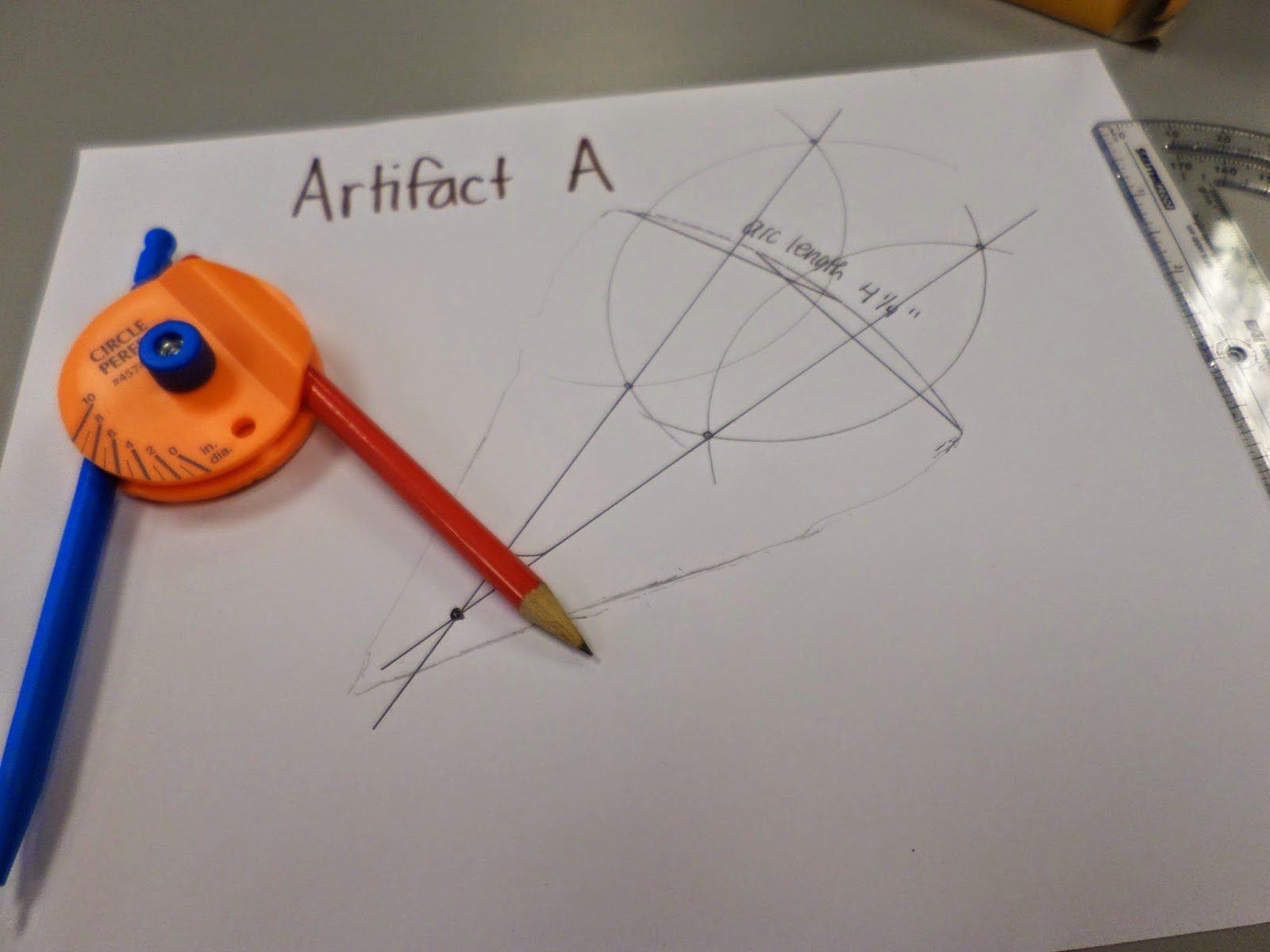
Our first step was to trace our artifact on plain copy paper.  This was a little tricky.  And sandy.  

I didn’t actually remember to take a picture of our artifact.  But, here’s the artifact of the other group sitting at our table.

Once we had our artifact traced, we had to decide on a strategy.  How could we figure out how large the original plate was?  Our first instinct was to measure the arc length.  That wasn’t much help.  We had been assured that the plates were not broken in the center.  So, how could we find the actual center?

This wasn’t the first time I had had this dilemma.  On Monday, we had to take a pre-test.  I usually do quite well on these types of things, but this test had several problems that stumped me.  On the test, there was a drawing of a piece of the top of a broken glass coffee table.  Given this piece of the broken table, instruct your friend what size of circular glass table top he needs to purchase.  After measuring the two sides and finding them to be different lengths, I was stumped.

Here I was again.  Same problem.  Different context.  Still stumped.

I guess my table looked lost/confused/dazed because one of the facilitators came over to give us a little shove in the right direction.  She told us that we needed to draw two chords.

My geometry is a bit rusty, but I can still draw chords.  Now what?  Thankfully one of the others at my table had taught geometry recently and remembered that the perpendicular bisector of a chord always goes through the center of the circle.  Once we found the perpendicular bisector of each chord, all we had to do was find where the bisectors intersected.  Then, it was just a matter of measuring the radius!    

And, yes, I did label my paper “Artifact A.”  I’m cool like that.

How fun is this activity?!?  It kinda even makes me want to teach geometry.  This was, by far, one of my favorite activities from the 5-day workshop.  I’ve always thought constructions were kinda cool, but I’ve never seen them as useful before this activity.

Here’s our reconstructed artifact:

After completing the project, we had a discussion on how to adapt this for our own classroom.  We decided that maybe using an actual broken plate wasn’t the best idea.  A kid could easily get hurt.  One teacher suggested cutting up Chinet plates for the activity.  Another said that you could break the plate and just hand out the tracings.  Keep the artifact in a ziplock bag so the kids could see it but not touch it.

Here are the instructions we were given in our binder:

Materials
* Large, flat, plastic pan for the digging site
* Ceramic plates of varying diameters – broken into 4 pieces with at least 3-4 inches of outside rim and not ending at the center
* Play sand
* Compass
* Protractor
* Pencil
* Plain Paper

Student Instructions
* Each pair of students should dig for a piece of the artifact.
* Trace the shape of the artifact on paper.
* Using any manipulatives or tools in the classroom, find the radius of your object.
* Find the other groups that have the same measurement and fit the pieces together to form the original artifact as best as you can.

Have you ever done anything like this in your classroom?  Can you see yourself using this activity?  Please share!