Point-Slope Form Dice Activity
This blog post contains Amazon affiliate links. As an Amazon Associate, I earn a small commission from qualifying purchases.
I created this point-slope form dice activity for my Algebra 1 students.
This year I did something wrong. Something seriously wrong.
What did I do wrong? I’m not entirely sure.
But, my students are convinced that point-slope form is easier than slope intercept form. This. Has. Never. Happened. Before. My students normally dread point-slope form. They cry. They whine.
They threaten to drop out of school because algebra is too hard. The week or so that we spend actively engaged in working with point-slope form is not a happy week. At least it wasn’t until this year.
So, what was different? I can think of two things.
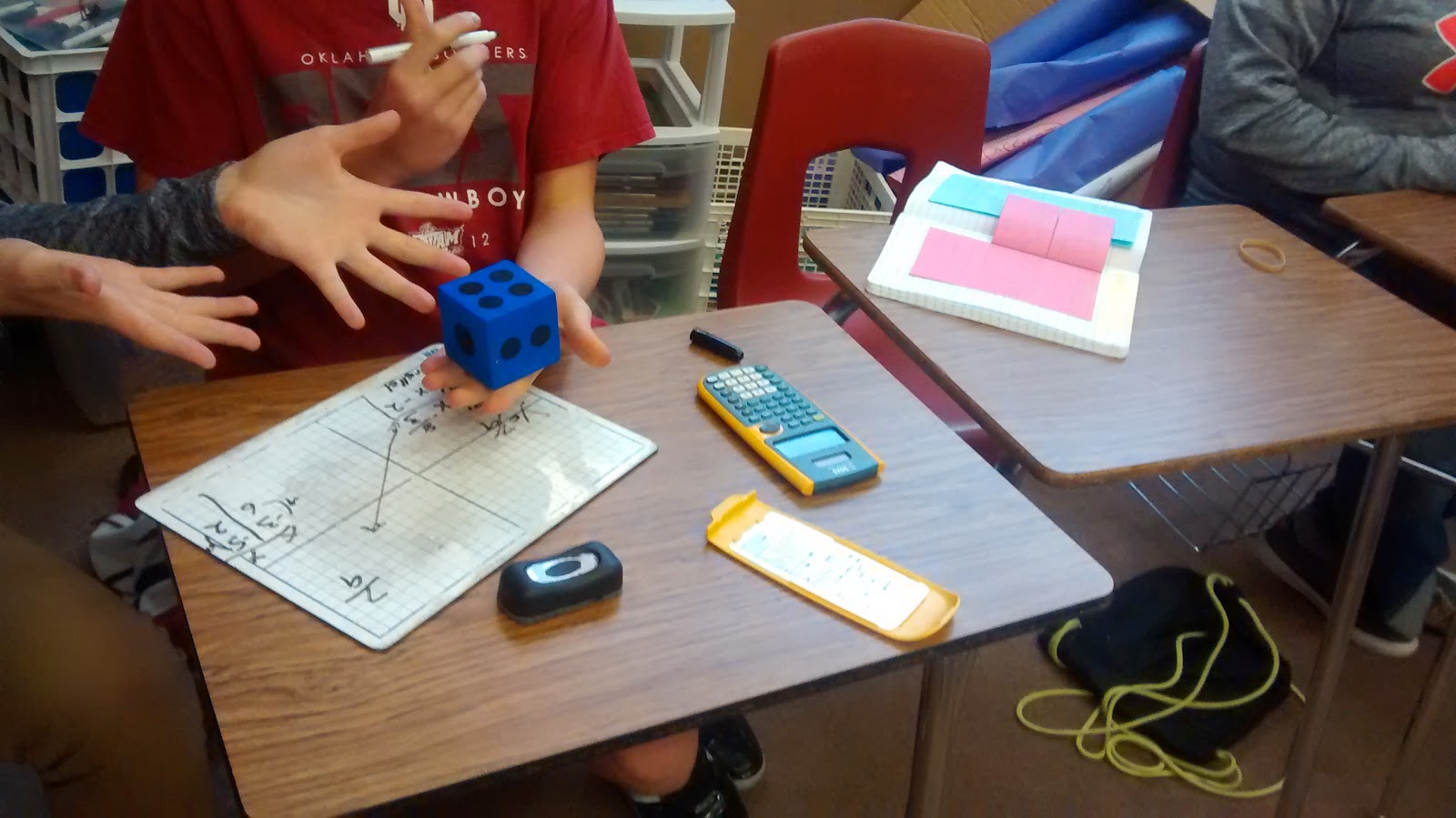
Instead of giving my students problems, I let them create their own problems. Or, I guess I should say the dice created their problems for them. First, students worked together in pairs of two.
Each pair got a giant foam die. My math teacher coworker across the hall gave me these right before school started. They are SO handy!
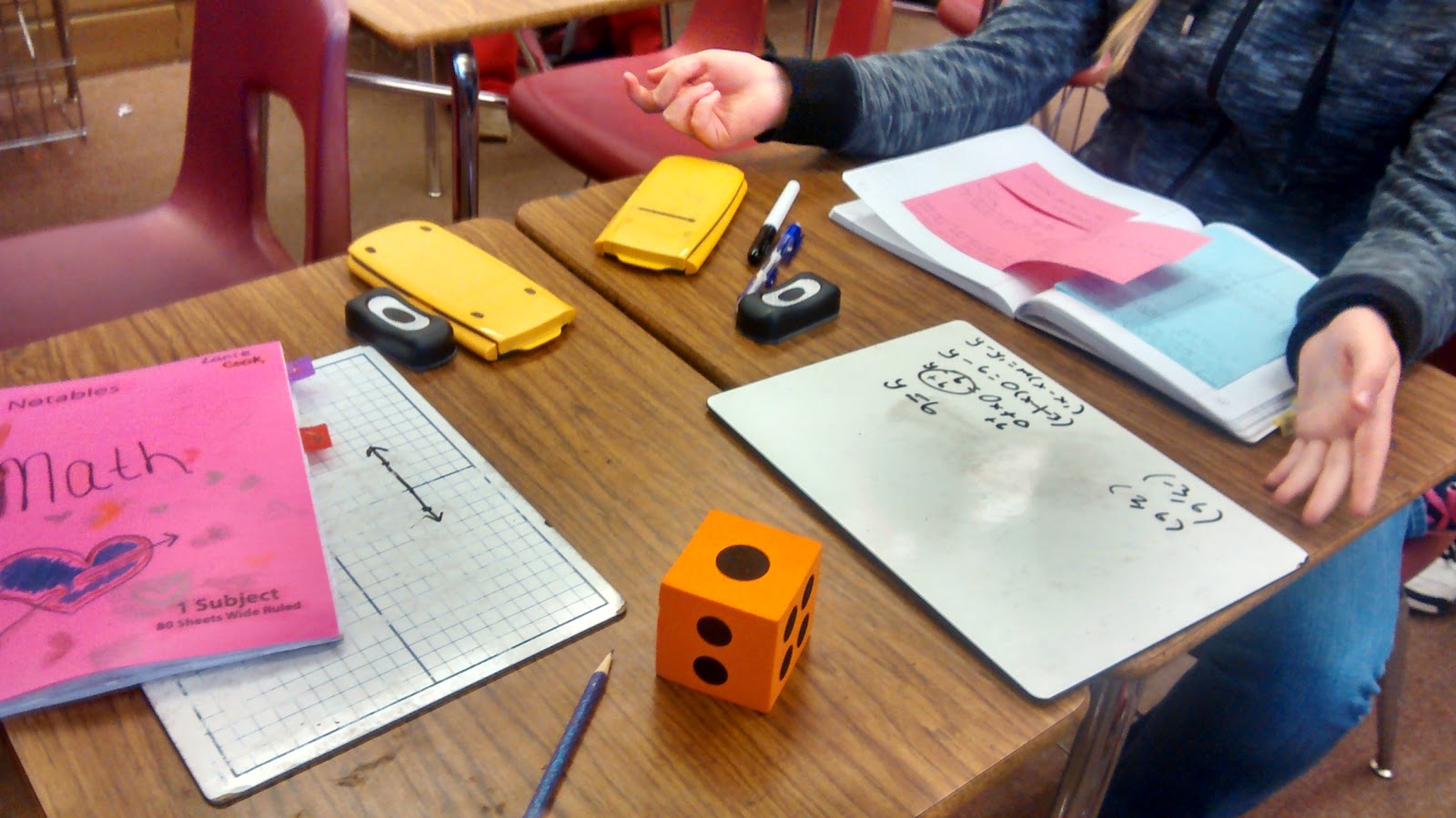
Decide before you start how to determine which numbers will be positive and which will be negative. We decided to make odd numbers negative and even numbers positive. This worked okay.
It led to a lot more horizontal and vertical lines than if you flipped a coin to determine positive/negative. But, I think I’m okay with that.
Usually, I neglect to give my students enough practice with problems where the points form a line with zero or undefined (or Undefiiiiiiiiiinnnnneeeeddd! as Slope Dude would say) slope.
Roll the die twice to form the first ordered pair. Roll the die two more times to form the second ordered pair. At this point, students knew from their notes on point-slope form that they had to determine the slope of the line that went through these two points.
However, my students knew they had two options. They could graph the two points and find the slope that way. Or, they could use a table and their vertical number lines to find the slope.
Students could pick whichever way they felt more comfortable.
It was so good to see kids using their notebooks. It’s a reminder that the notes we take in class really do make a difference.
Having found the slope, students plugged the slope and one of their points into the formula for point-slope form. Then, we converted the equation to slope intercept form for even more practice with slope-intercept form.
This is where things take a radical turn from how I’ve done them in the past. Usually, I would be like “Yay, we found the equation of the line. Go us! Let’s do another one.”
The problem with this is that my students aren’t convinced that this equation actually goes through these points. Assuming we’ve made no errors, I’m convinced. But, then again, I’m an algebra teacher.
The thought of teaching equations is what makes me want to get out of bed in the morning.
My students? Not so much.
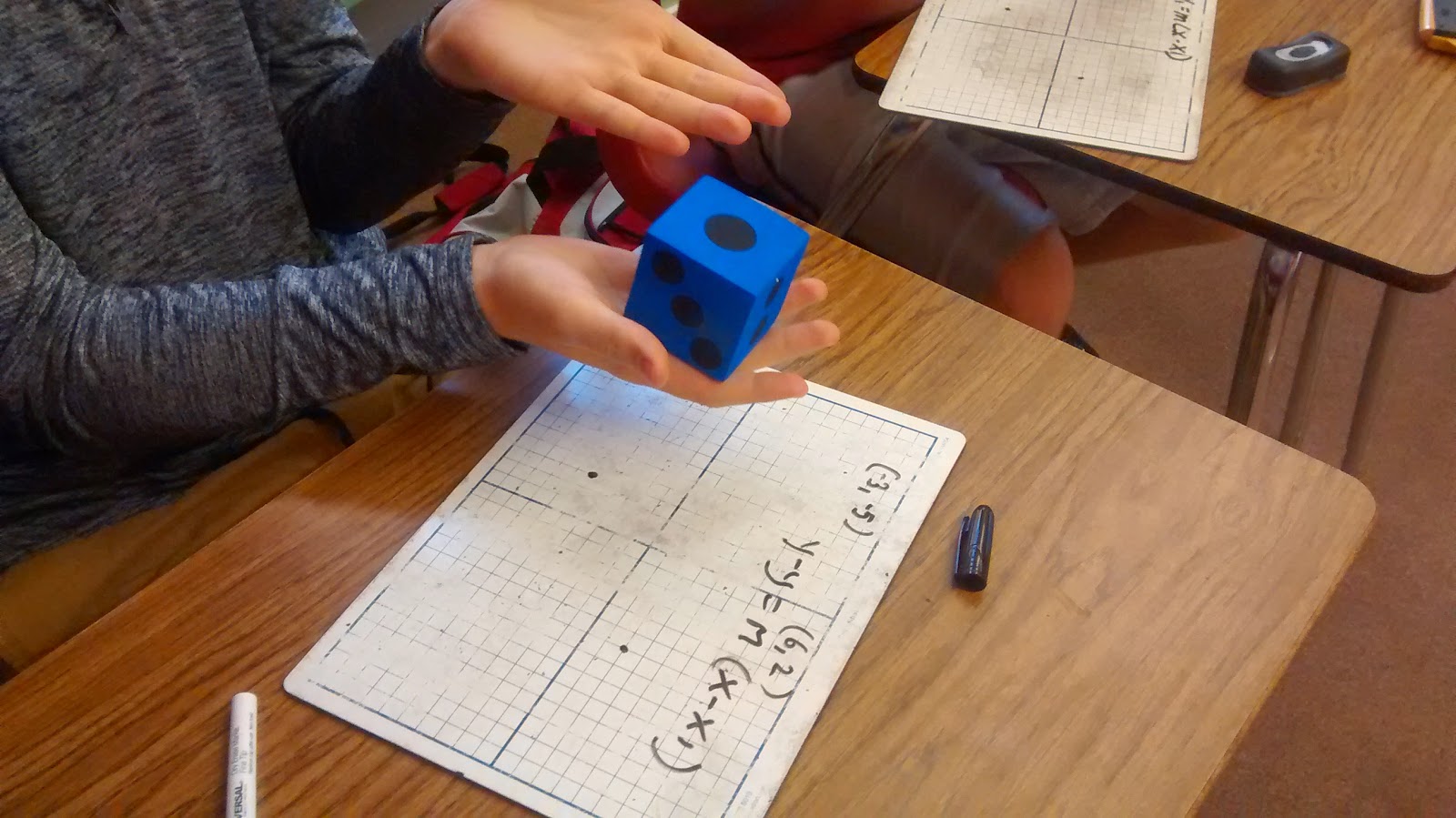
My students need to be convinced, though. I could have them graph the two points and graph the line they found in slope intercept form. But, knowing my students, they would probably just draw a line through the two points and “pretend” it matched the equation.
No, they need better proof. Definitive proof. And, that’s what desmos provided us.
If my school had laptops, or ipads, or wifi access for students, I would let them each check their own work as they went. But, sadly, that’s not the type of school I work in. We don’t even have a computer lab that we can take students to. I see the activities that other teachers are able to do with their students and desmos, and I get super jealous.
Extremely jealous. Life goes on, though. And, I remind myself that I am providing the best education for my students that I know how to with the resources I have been provided with.
Anyway. Let’s get back to how I was able to use desmos in my classroom. I pulled up desmos on my desktop computer. As pairs of students found the equations that went through their pairs of points, I had them bring their dry erase boards up to my desk.
I inserted the two points they had rolled with the dice into desmos. Then, with great fanfare, I typed in the equation they had come up with.
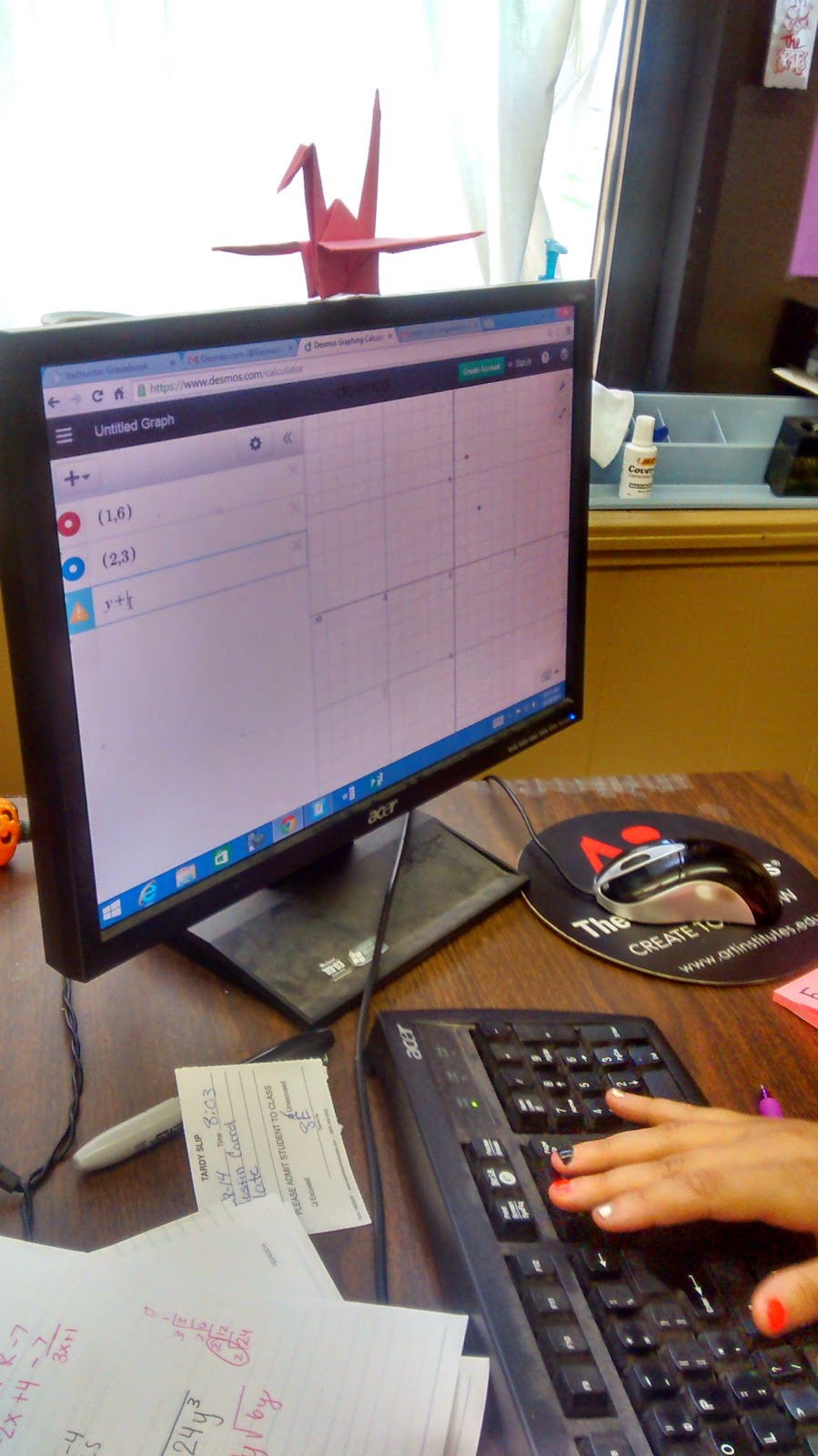
One of two things happened. Cheers because the line very clearly went through both points. Or groans. The students who cheered were sent back to their desks to repeat the process with newly rolled points.
The students who groaned were sent back to their desks to look for their mistake.
The feedback was instant and glorious. Thank you Desmos!
And, there’s just something different about the computer screen telling you you’re right and your math teacher telling you you’re right.
As the year has progressed, students still ask sometimes if we can “desmos” something when they want to check their work. And, of course, I always say yes. 🙂
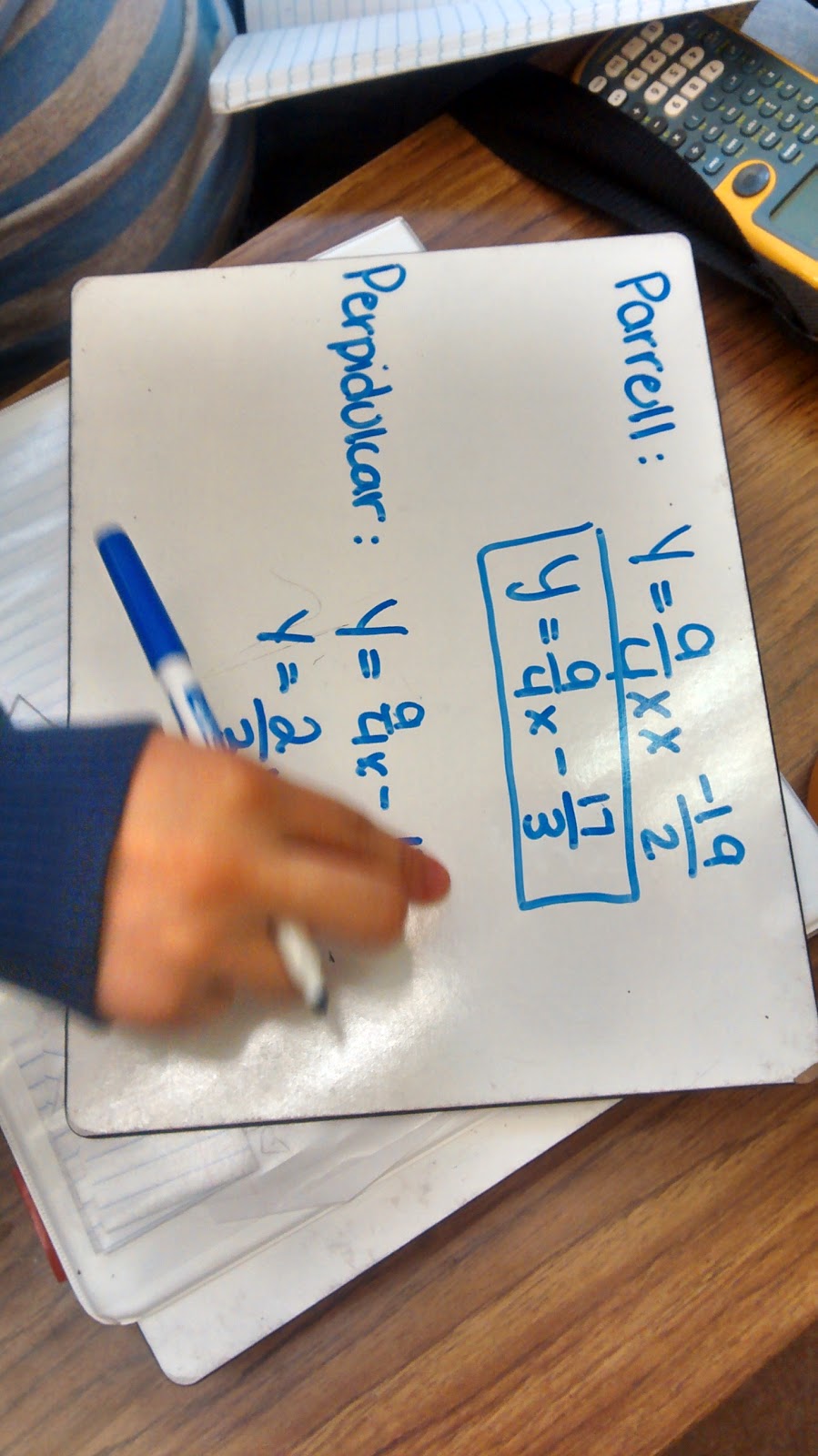
Later on in the chapter, we were studying parallel and perpendicular lines. We pulled out the dice again. Roll the dice. Form two points. Find a line that is parallel to the line that goes through these two points.
Find a line that is perpendicular to the line that goes through these two points. More desmos. More awesomeness.
Though, I guess we do need to work a bit on our spelling of parallel and perpendicular. 😉
More Activities for Teaching Forms of Linear Equations
- Forms of Linear Equations Foldable
- Rearranging Equations for y Foldable
- Converting Equations to Slope Intercept Form Notes
- Point-Slope Form Dice Activity
- Different Forms of Linear Functions Foldable
- Linear Foldable y=a+bx
- Flyswatter Review Game for Different Forms of Linear Equations
- Point-Slope Form Foldable
- Standard Form of a Linear Equation Cut and Paste Activity
- Standard Form of a Linear Equation Foldable
- Slope Intercept Form y=mx+b Foldable
I'm just glad that my students aren't the only ones who can't spell parallel. This is a great lesson!
I LOVE Desmos. I just finished up an activity packet for discovering how linear inequalities are graphed using Demos. My kids love it, too; in fact, they're the ones who discovered there's an app and had it downloaded months before me!
Point-slope form is interesting, huh? I've found my kids have really liked it, too. I don't teach it to my Math 8 students, just my Algebra class, and some of my Math 8 class has actually been begging to learn how since they saw it on a warm-up that I usually use for Algebra only. Kids are so fickle about what they like in school. 🙂
Love this and Desmos! Make sure you send this URL to them, they love feedback! Amy
Thanks Amy!
Hi Sarah,
Thanks for sharing this lesson. I just checked out Desmos and WOW! In the next unit students will be exploring systems of equations. Last year it was a huge struggle to ensure each student had the right tools to check their work. This program is perfect to use in conjunction with the iPad. Is there a way to have students create free accounts and share graphs with me?
Thanks,
Pamela
Glad I could share the desmos love with you! I'm not sure about using it with student accounts, though. Hope you are able to figure it out!
Howdy Sarah my name is Colby,
I heard about your teaching methods via NPR on the drive home from work today. I just wanted to say its awesome you have turned a traditionally disliked subject into a fun learning experience for the students. Keep up the great work : ).
Great idea to use the dice and Desmos in that way! Thanks for the ideas 🙂
Sarah,
I, too, learned of you on NPR. I'm a 34 year old nurse in North Carolina that had to drop out of university and get my degree from a community college because I could not get through the math requirements. I am now facing being required to finish my degree and of course, will also require that math that I skirted around many years ago! I wish I had an amazing teacher like you to have taught me fundamentals so I would've been better prepared going into college.
Thank you for your sacrifice and dedication to these students. The ripple effect is taking place every day all because of teachers like you!
That is fantastic! And I just happen to have a few dice and some whiteboards. Thank you so much for posting your ideas. I've shared your blog with the other math teachers in my school board in Quebec.
Hello from Canada!
Here's how to teach 'em how to spell parallel:
It's like "A pair a LLs" ( para-llel) and the visual can reinforce what parallel lines should look like.
Those kids are lucky to have you!
have the same problem with no tech in my math classroom – your creative problem solvign had the kids come out stronger! thank you
For the students that would rather give up their right arm than their smartphone, Desmos is available as an app. If you can't beat them, join them.
I loved your idea of using dice to get a set of coordinates. Before we got to the writing an equation of a line portion I used the dice to find a slope like you did. The students paired off and rolled the dice. They had to use the slope formula to find the slope and compare it to their partner/opponent to determine if whose slope was steeper. The student with the steeper slope got to rotate to the next seat. They ended up competing with everyone as they rotated around the room. The class then came together to discuss their results that were recorded on their paper. We talked about who had the most wins and losses, who had the steepest slope in the room, the least steep slope (not counting the vertical or horizontal ones), and how many vertical and horizontal lines there were. I provided prizes for the kids with the most and least wins and losses, etc. The kids loved the competition from this activity and they were creating their own coordinates/problems to work out. I used 6,8 & 10 sided dice. Some were in different colors so I made a certain dice color the negative value so they would get practice with negative values too. Thanks for sharing all your great ideas.
Love this idea! Let the students create their problems! It’s more interactive and super adaptable. Thinking of using this activity for Standard form as well. Roll the die for an X intercept and y intercept.
Great suggestion!
You are so amazing. I cannot wait to try this activity with my students tomorrow. Thank you for all that you do to inspire me every day!
Awesome! I hope they enjoyed the activity!