Russian Fable for Marriage Probability Activity

My statistics class recently worked through a probability activity involving a Russian fable regarding marriage from the MEP Demonstration Project.

I’m gonna be honest.  My students and I have way too many conversations about my current relationship status.  I’m not married, and that bothers them.  Students have stopped me in the Wal-Mart parking lot to tell me who they think I should be dating.  They interrupt class to give me dating advice.  I’d love to think that this is because they care so much about me.  But, sometimes I think they’ll talk about anything if it gets them out of talking about math.

Last week, I was walking down the hall during my planning period when a student in the office called out my name.  This was a student I had last year for Algebra 1.  I have students I get along with extraordinarily well.  This student is not one of them.  We tolerate each other, and that’s about as far as it goes.  Last year, he made it very clear, through his actions, that he did not want to be in my class.  I walked in to the office, and this is the conversation that followed:

Student: Do you still eat lunch with the science teacher every week?
Me: Ummm… I’ve never eaten lunch with the science teacher.
Student:  Yes, you have.  I’ve seen you.
Me: No, I haven’t.  I promise.
Student:  Well, maybe you should start.  Where do you eat lunch?
Me: I eat lunch in my classroom by myself.
Student: Why?
Me: I’ve got things to do.
Student: Well, you’re never going to find a boyfriend that way!

The conversation took a turn after this.  Much to my surprise, this student expressed excitement over the fact that he was going to get to have me for Algebra 2 next year.  “You are going to teach Algebra 2 next year, right?”  “I am going to get to have you next year, right?”  “You are going to still be here next year, right?”  “If you’re not here next year, I’m going to hunt you down and find you.”  I’m going to take that threat as a compliment.  This is proof that students do come around.  He isn’t the first student this year to come to me and tell me, in retrospect, how much he enjoyed my class last year.  This is important because it seems like I have a lot more haters this year.  There’s hope, though.  They may just come around, too.  

So, enough of that tangent.  Let’s talk about some math.  Remember the cuboctahedrons?  In the same document that I found the cuboctahedron net in, I also found a reference to a Russian fable that predicted whether a young lady would get married in the next year.  It seemed like an interesting probability problem. This activity is from the MEP Demonstration Project.

Last week, I posed the problem to my statistics students.  Apparently, in some Russian villages, they use a certain method to determine which girls will be married in the next year.  Fold three long blades of grass in two.  Have someone hold them so the loose ends are hanging down.  Tie the ends together in three knots.  The person holding the blades of grass lets go.  If your knots have led to the formation of one large loop, you will supposedly be married in the next year.

Is this true?  I have no clue.  Either way, it still makes for an interesting problem.  First off, what is the probability that you will end up with a large loop?  I wish I had had my students write down their gut instinct regarding the probability before making any calculations.  To me, the likelihood that a large loop would be formed was quite slim.

I set my students loose on this problem, and they were quickly frustrated.  During this time, I had been cutting some pieces of clothesline rope (the only thing I could find in my cabinet that would work!) to model this scenario.  A pair of students acted out the ritual.  And, amazingly, a large loop was formed!  Wedding bells would be ringing!  Another pair of students acted out the ritual.  Wow, another large loop!  Maybe this is more likely than we thought…

I only have five statistics students.  After five trials, we had 3 successes and 2 failures.  It was my turn.  A student held the pieces of rope for me.  I tied the ends together in three separate knots.  By this time, I had made a probability tree model and knew that the probability was over 50 percent that I would be married in the next year.  (I won’t give away the actual probability in case you want to try out this problem on your own.)

What was my result?  I know you are DYING to know.

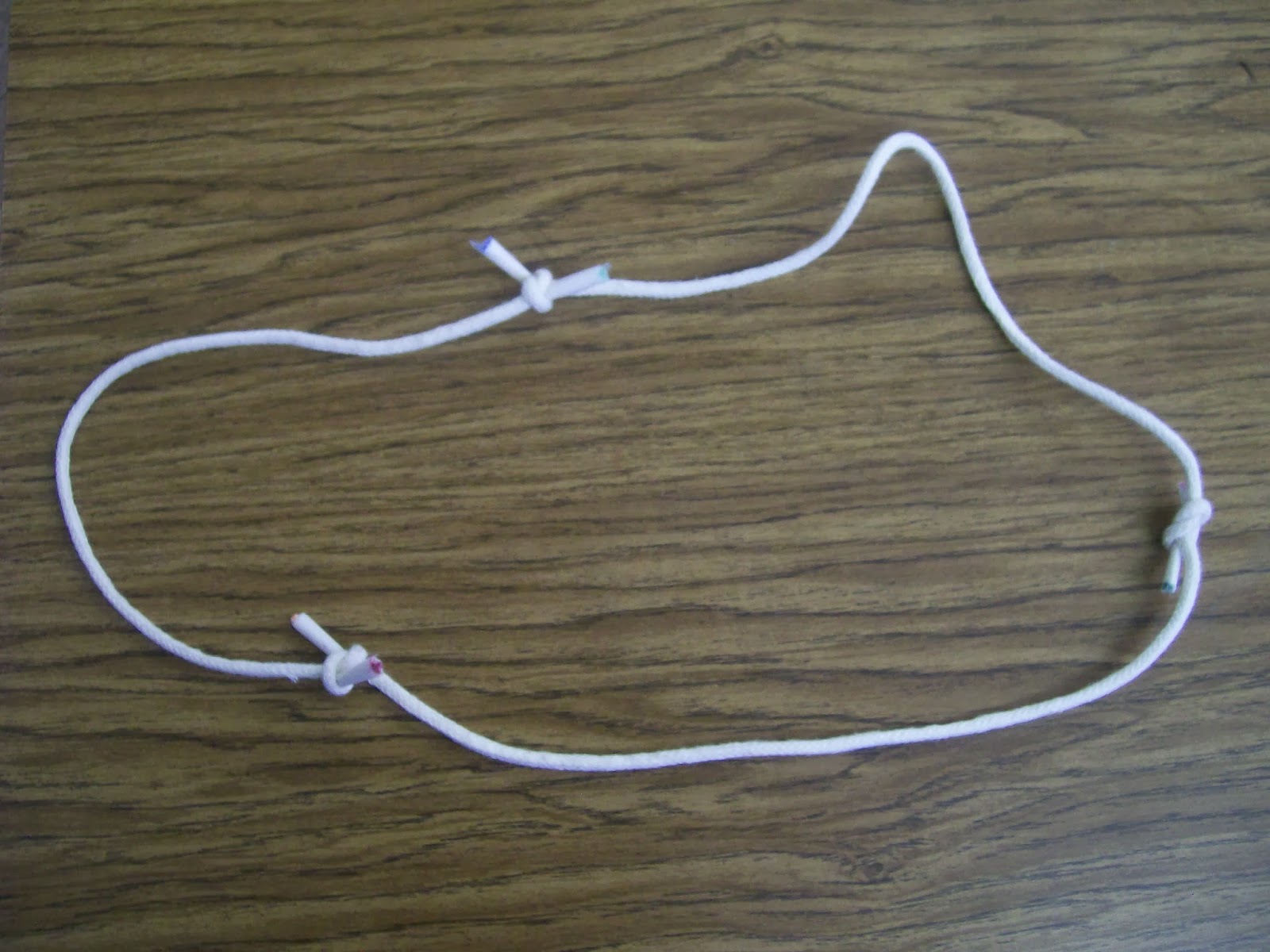
Not surprisingly, I am supposed to be wed in the next 51 weeks according to this piece of rope.

We’ll see…

I ended up having to walk my students through how to make the tree diagram for this problem.  We haven’t done much work with tree diagrams because they aren’t presented in our textbook.  If I ever teach statistics again, I will introduce tree diagrams and then have students walk through this problem with the instruction of making a tree diagram.

One student wondered if this is where the term “tying the knot” originated.  I did some googling, and it doesn’t seem so.  But, I thought that was very creative thinking!