Dice Activity for Reviewing Square Roots

Today I want to share with you the best activity ever for reviewing square roots. This is the first time I have ever taught my students to simplify square roots.  So, it may not be the best activity ever.  But, I can tell you that I have never had more engaged students in my Algebra 2 class than the day we did this activity.

My next lesson to be taught in Algebra 2 is solving quadratic equations by taking square roots.  We’ve already solved by factoring and solved by graphing.

Since my Algebra 2 students are lacking some essential Algebra 1 skills, I decided it would be best to spend a day reviewing square roots before trying to solve by finding the square root.

I decided the best way to review square roots was to do a ton of practice problems.  I created a Smart Board file with page after page after page of practice problems.  Students would copy the problem on their dry erase board and solve.

After making so many slides, I was bored.  And, I knew that if I was bored my students would most definitely be bored.  Ten minutes before class was about to start, I had an epiphany.  What if I made the number under the radical sign random and unpredictable?  There’s no better way to do that than using dice.

I pulled up the multiple dice file from the Smart Board Gallery and stuck it under a radical sign.

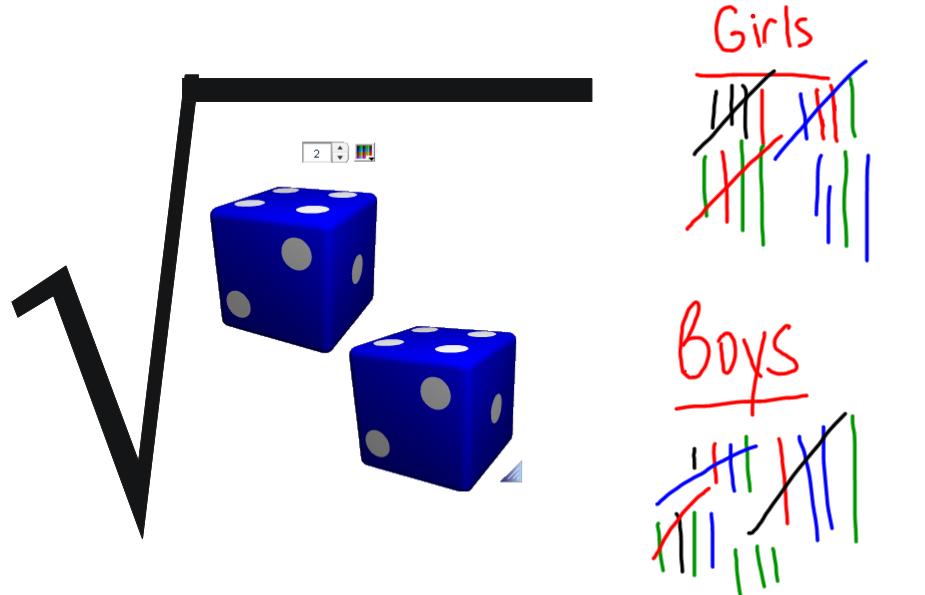
Now, we could roll the dice to find the next number to take the square root of.  Two dice = two digit number.  So, in the above example, the number would be 22.

I love that the students never know if the square root created by the dice will be a perfect square, simplify partially, or not simplify at all.   

We broke up into boys vs. girls.  I would touch the Smart Board to flip the dice.  The two selected players would race to see who could find the square root first.  However, everybody was supposed to solve every problem on their dry erase board.

As you can see from our score tally, the competition was intense.  The girls would pull ahead by one point.  Then the boys would catch up.  The boys would get ahead, and then they would fall behind again.  I have never seen my Algebra 2 students get this engaged in an activity before.   

Things I Would Change for Next Time

• Have a plan for who will compete against who.  I let the students decide, and it didn’t work as well as I intended.  Some students didn’t compete as much as others, but I didn’t really have a way to regulate this because I didn’t have a plan going in.  
• From the start, tell them that the first answer that they hold up is their one and only answer.  Otherwise, students will just hold up the problem, hoping that it won’t simplify any further.  Force them to fully work through the problem before selecting their answer. 
• Come up with a better way to determine which team finishes first.  As the students got better at recognizing the square roots that wouldn’t simplify, I began having trouble determining exactly which team held up their answer first.  This led to some calls that were protested.