Factoring Quadratics Graphic Organizers
Today I want to share some graphic organizers I created to remind students the steps for factoring quadratics. We filled these out and glued them in our algebra interactive notebooks.
This year, I am taking a big risk with my Algebra 1 kiddos. I taught them to factor quadratic trinomials with a leading coefficient greater than one before we ever discussed solving equations.
Last year, I crammed in factoring quadratics at the very end of the school year. I was feeling rushed, and I needed to cover factoring quadratics and simplifying radicals before the end-of-instruction exam. I didn’t feel like I really did either topic justice because I was so rushed.
This year is different. Really different. My Algebra 1 students came in at a much lower level than my students last year.
After spending weeks on integer operations and the order of operations and all that fun stuff that students should already know from middle school, I still had a lot of kids who were just not getting it. That was nothing new.
Last year, my students struggled with these topics, too. And, last year, I moved on to solving equations and HOPED that the rules for dealing with integers and all that good stuff would “click” when they started seeing it in equations. That worked to a certain extent.
This year, I could tell I was more frustrated and my students were more frustrated than normal. The kids who understood integer operations were bored out of their minds.
They wanted to move onto something new. The kids who didn’t understand integer operations still didn’t quite know that they didn’t understand them. So, my constant review as futile.
My students had solved equations in middle school, so that wouldn’t be something new and exciting. After teaching them the distributive property, I had an epiphany.
I made a commitment to myself last year that I would stop teaching students to FOIL. I learned to multiply binomials by FOILing. But, I’m learning that some of the tricks that I was taught in Algebra are just that.
They are tricks that work, but students don’t quite know why they work. If my students can see a problem as an extension of the distributive property, they can solve a myriad of problems in different forms.
If students only know how to FOIL, they are going to be stuck and not know what to do when they are asked to multiply a trinomial and a binomial.
Last year, instead of teaching students to FOIL, I taught students what I called “The Double Distributive Property.” This could be extended to the triple distributive property as well. And, it worked out pretty well.
What if I didn’t teach the double distributive property as a separate property? What if just told my students that when they see two polynomials being multiplied together that it is a distributive property problem?
So, I did just that. Day one of the distributive property featured monomials times binomials. Day two of the distributive property featured polynomials times polynomials. I made no distinction between the two.
When my students see two polynomials being multiplied, they automatically think distributive property. And, that makes me insanely happy.
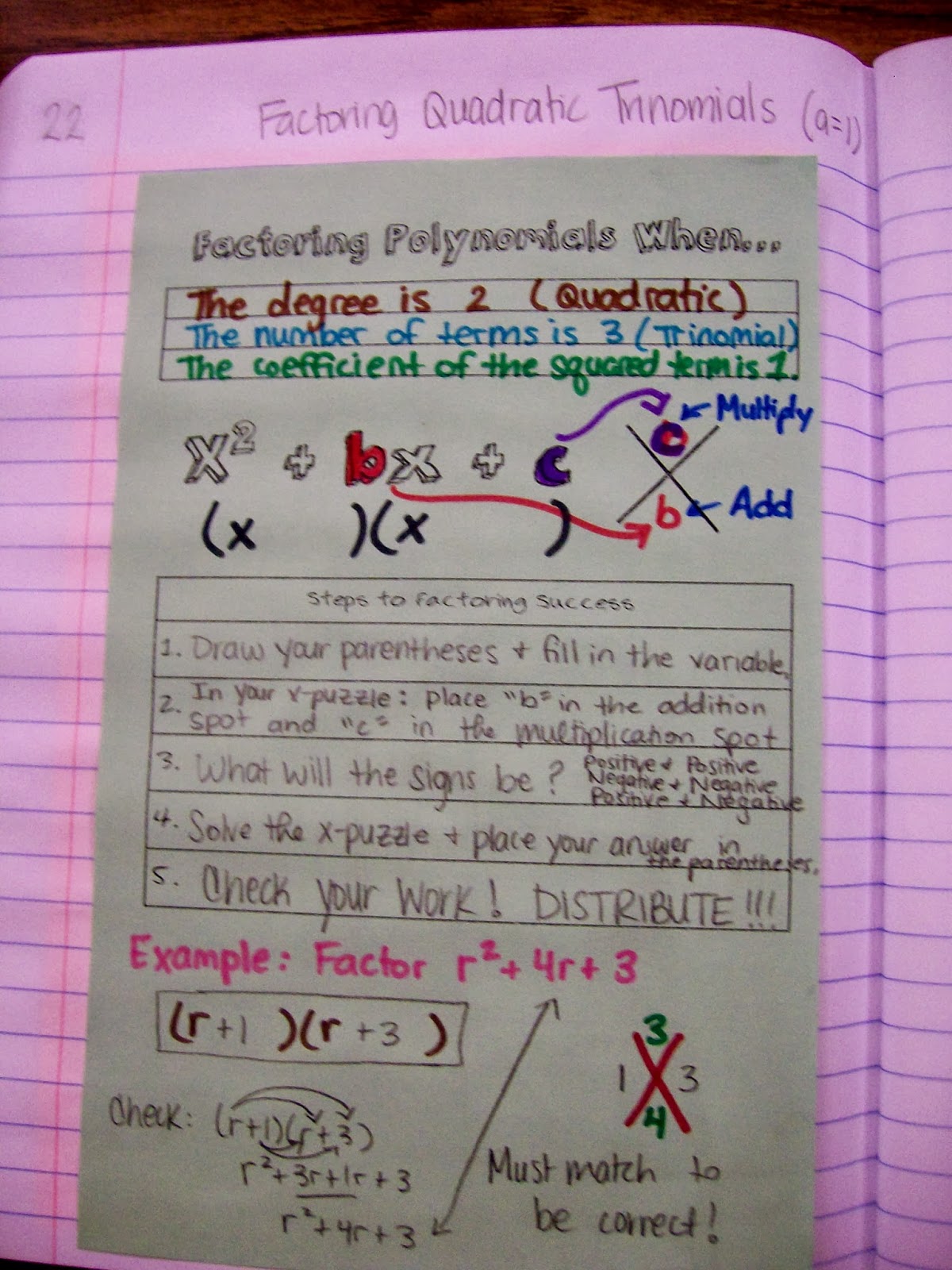
Factoring Quadratic Trinomials with a Leading Coefficient of 1
After teaching students to distribute, the natural thing to teach students is to undistribute, or factor.
When my students were first reviewing integer operations, I gave them a sheet of diamond puzzles or X-puzzles to complete. I taught my students to factor quadratics with a leading coefficient of 1 using the X-puzzle.
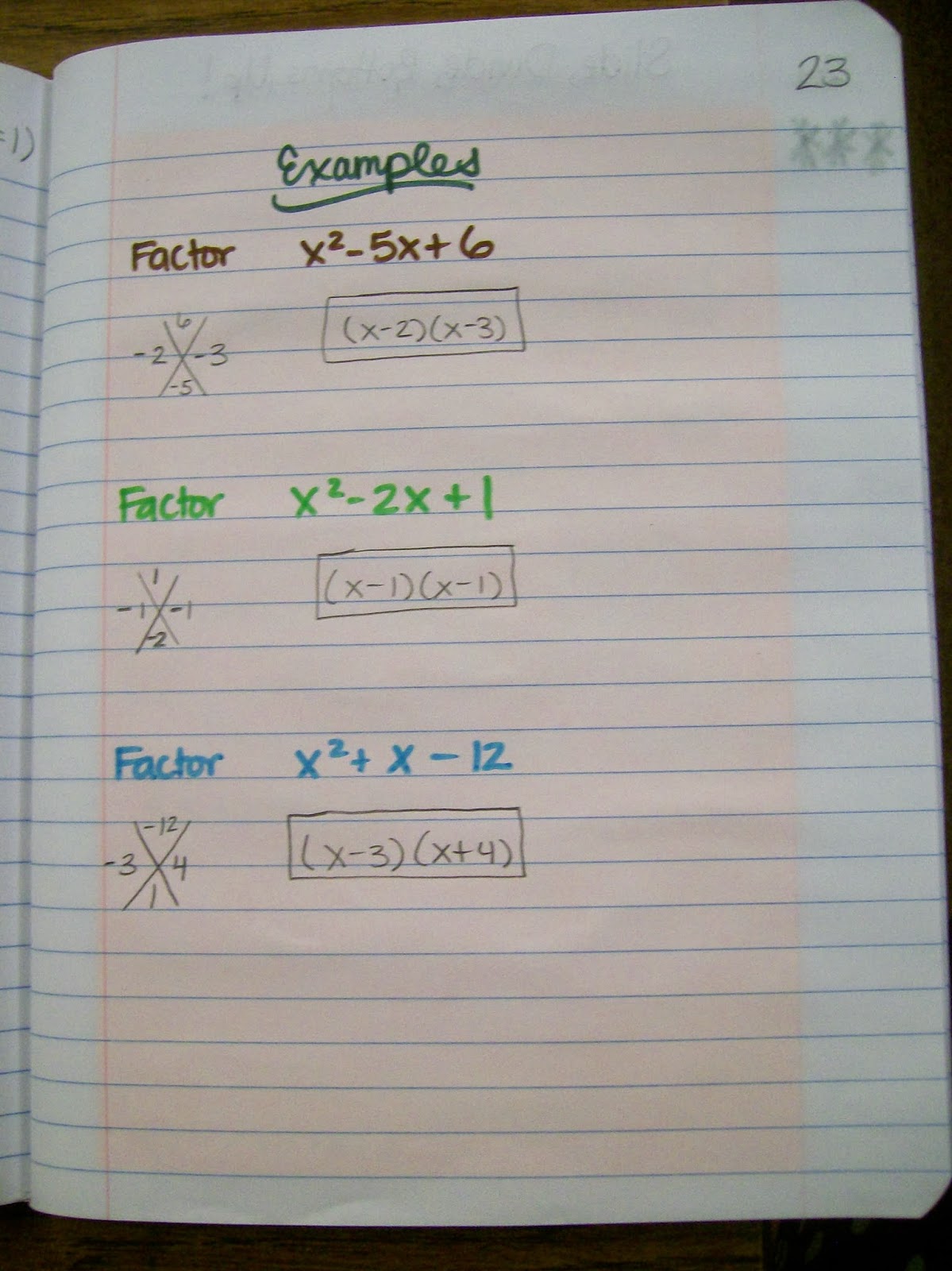
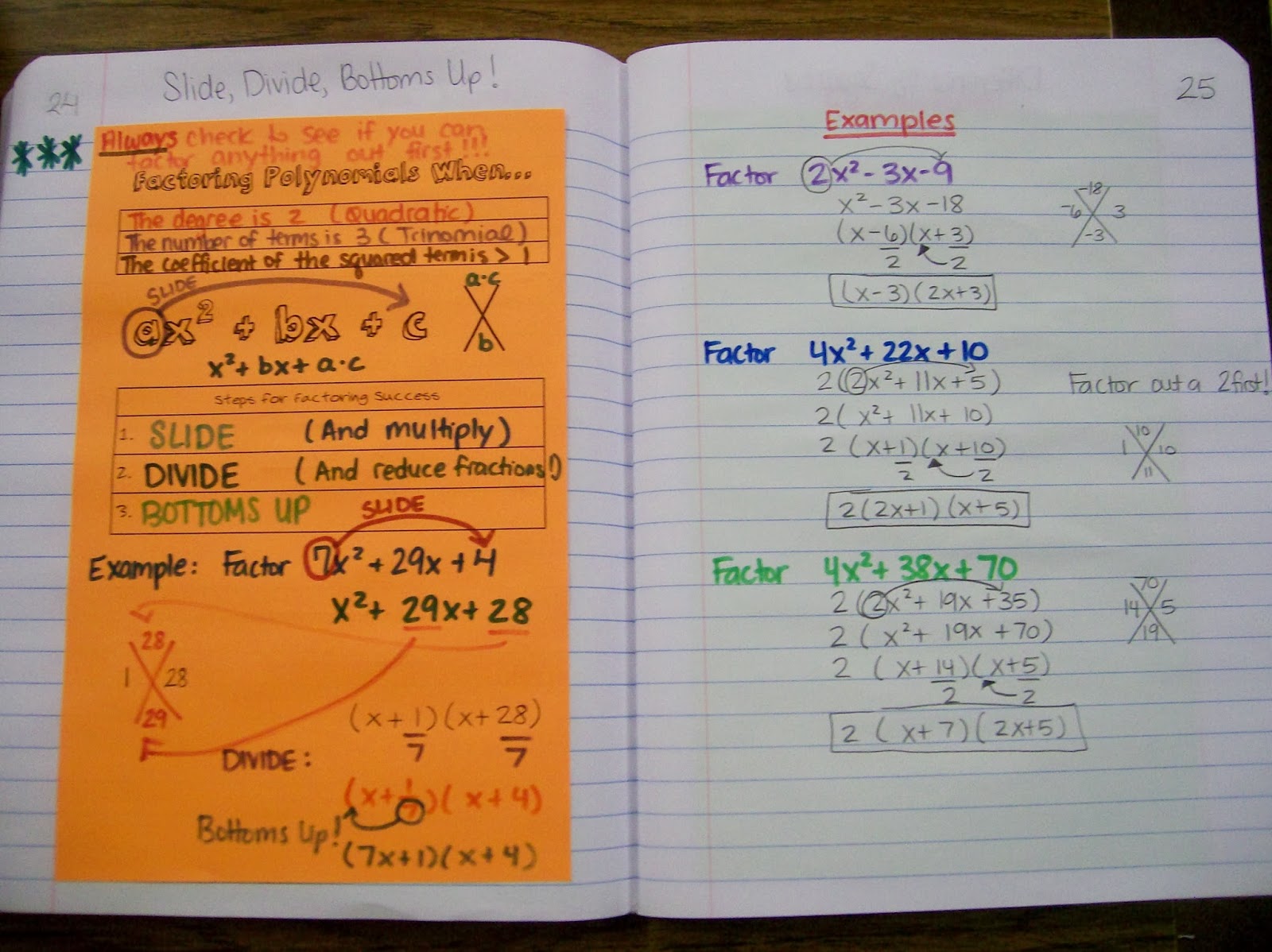
Factoring Quadratic Trinomials with a Leading Coefficient Greater than One
This more affectionately known as Slide, Divide, Bottoms UP!
Again, I changed my teaching approach from last year. Last year, I taught my students to do the Airplane Method. This worked. But, I still had a few students who never caught on.
This summer, at the Common Core conference I went to, I was introduced to the Slide and Divide method of factoring. Another teacher mentioned that she called it the Bottoms Up Method.
I combined these two ideas to create the “Slide, Divide, Bottoms Up!” Method. My students LOVE it.
Yes, this is a trick. But, I haven’t figured out a better way to teach it. When I took Algebra 1, I learned the guess and check method. And, I found that method to be torturous. But, I didn’t know there was a better, faster, easier way. Now that I do know there is a better way, I would never go back.
Please, please, please do not use this slide, divide, bottoms up method with your students. I now know better, and I don’t want you to be led astray by my new teacher enthusiasm for a factoring trick.
I assumed that because the trick was shared at a workshop for teachers that it was a recommended method for using in the classroom. I was wrong. There is a better way if you don’t want to teach the guess and check method.
It’s called the box method (or area model.) It works for ALL types of factoring problems. It is much more conceptual, and I have found that students actually understand what they are doing instead of rotely following an algorithm that you have asked them to memorize.
Plus, the amazing thing about the box method is that you can even use it to divide polynomials!
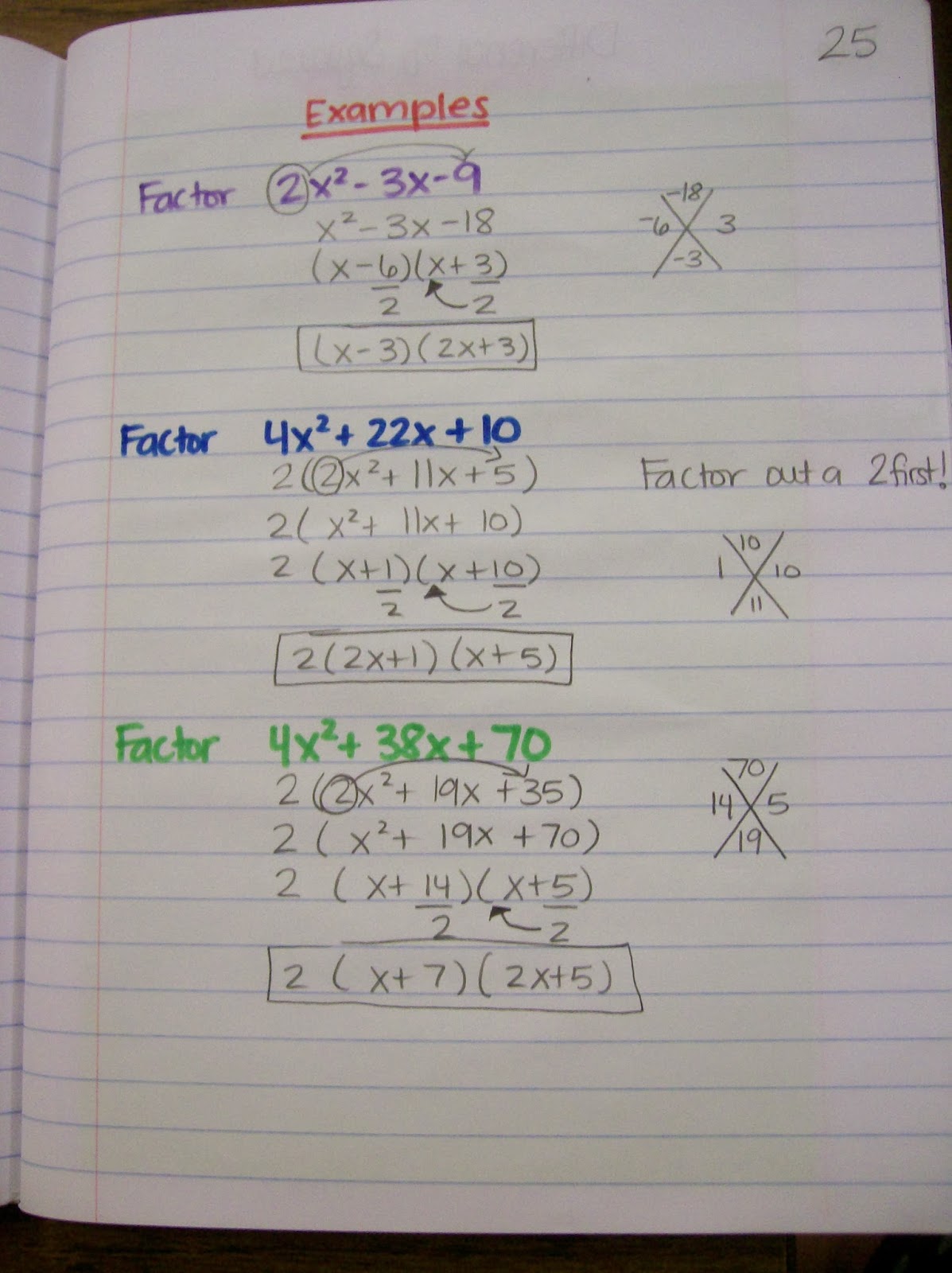
Factoring Difference of Squares
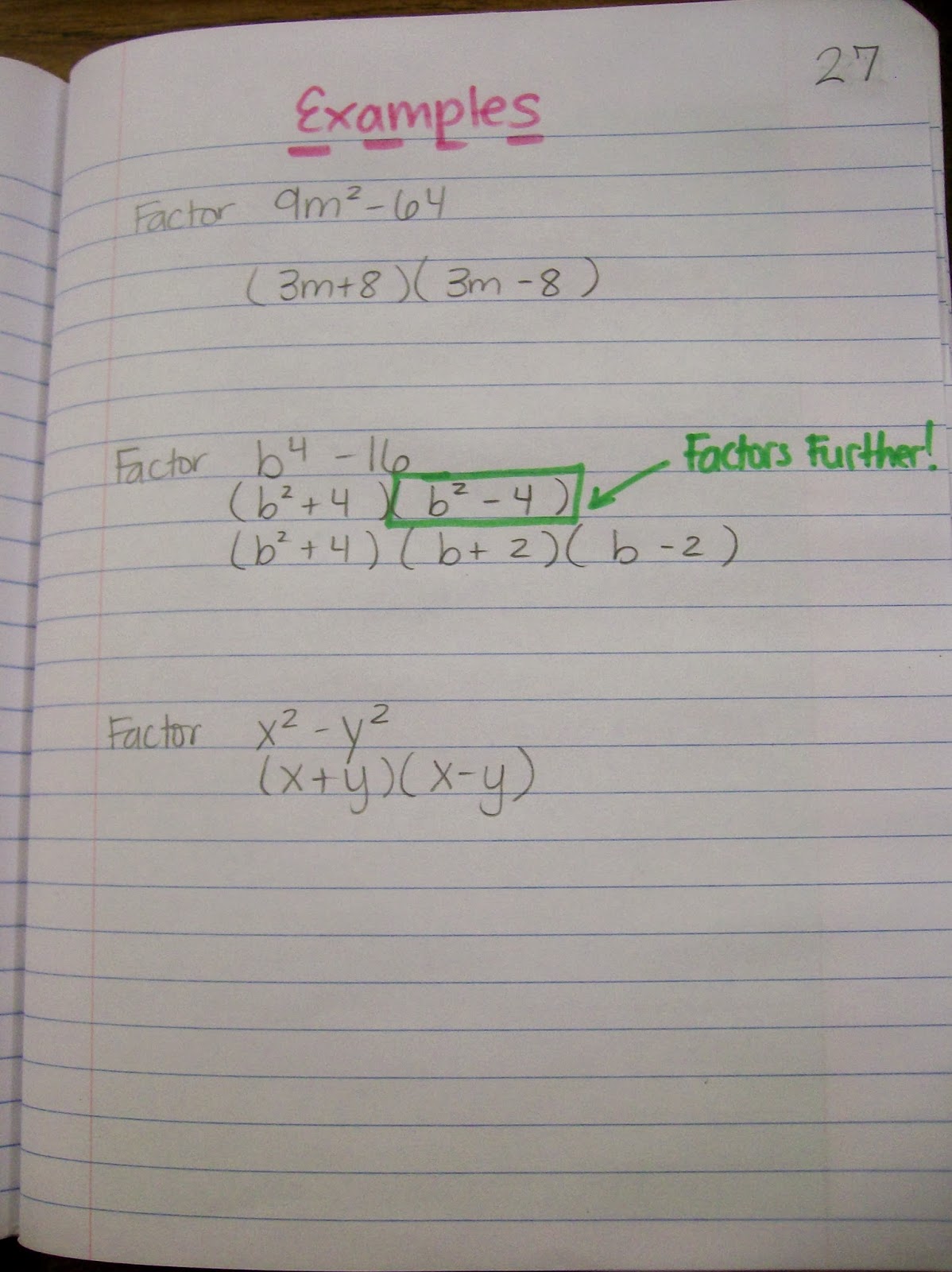
Free Download of Factoring Quadratics Graphic Organizers
Factoring when a is 1 Graphic Organizer (PDF) (2758 downloads )
Factoring when a is greater than 1 Graphic Organizer (PDF) (2645 downloads )
Factoring Difference of Squares Graphic Organizer (PDF) (2171 downloads )
Looking for resources to give students practice with factoring? I recommend checking out this Factoring Puzzle.
More Resources for Teaching Quadratics
- Quadratic Formula Activities
- Factoring Activities
- Speedy Squares Activity for Quadratic Regression
- X Puzzles Factoring Review Game
- Quadratic Area Puzzles
- Factoring Puzzle for Quadratic Trinomials
- Shared Factors – A Quadratics Puzzle
- Factoring Quadratics Practice Activity (When a = 1)
- If the IRS had discovered the quadratic formula…
- Area Model Puzzles from Christie Bradshaw
- ZERO Game to Introduce Factoring Quadratics
- Vertex Form of a Quadratic Card Sort Activity
Have you tried factoring by grouping? It's not a trick and is not guess or check. It is doing double distributive in reverse.
This is how I plan on teaching it next year! A friend showed it to me a few months ago, and I fell in love with it!
Just saw this post — I have always taught by grouping. I teach grouping before doing this and then it is so easy for them. Also I have NEVER in 25+ years used FOIL — it just seemed silly. I have always used the double distributive method since they already understand distributive. Also, I make them draw arrows from the 1st set of parentheses to the 2nd — 1st term, arrows above; 2nd term, arrows below. We call them "rainbows". So now I say "Use your rainbows" and they remember!
Thanks for sharing! I've become a convert to the box method lately.
I love the box method because it is so much more organized than the table method. I also don't use FOIL (though I do show them because I've been "told" I "have to" teach it because "everyone" uses FOIL. I also call it double distribution! I am going to look into grouping more (I do teach it but for specific quadratics).
After I show my kids the box method, I sometimes show them FOIL if someone asks about it. I love when they see right off the bat that it's the EXACT same thing. They usually remark that the box method is so much more organized than all the arrows. Make this teacher's heart happy. <3
As I'm preparing to teach factoring to my 9th grade Algebra students, I stumbled on this post. It sounds like you use the box method for multiplying binomials…have you tried it for factoring as well? It's a nice visual set up of the grouping method. I've tried slide and divide in the past (though I like the "bottoms up" name better!) but I don't actually have them "slide" the a over. I just ALWAYS have them multiply a and c to get the top of the diamond (even if it's a 1). That way, when they look back at the original problem, they don't mistakenly look at the "slide" version and forget to divide. Also, I've found it helpful to have students do the "divide" part with the numbers still in the diamond. They seem less likely to forget that step when we set it up that way.
I've seen on several of your posts comments about factoring by grouping. Do you have any ISNB pages for factoring by grouping? Or a blog post about teaching it? I am getting ready to teach our polynomial unit which includes factoring. Thanks!
Tabitha, I'm not Sarah, but I'd be glad to share with you what I have if you want to share your email address. Mine is teri030709[at]gmail.com.
Or I have posted it on my blog now…
http://coachmrsandlittle.weebly.com/blog/factoring-by-grouping-advanced-organizer
I've used a hybrid of factoring by grouping. I'll see if I can find the handout I have for my class and email it to you. Factoring by grouping is great because it really reflects the "undoing" of the double distributive property. It's more work, but they can SEE it happening.
I would love to see that! One of my co-workers and never heard of the slide and divide method. I am all about learning different methods..
Me too! I've taught factoring differently every single time I've ever taught it. Next year, I'm thinking of doing factoring by grouping. Slide, Divide, Bottoms Up worked great. My kids just can't seem to remember the steps now that it's been a few months. I'm still on the lookout for the perfect way to teach factoring…
Love the emphasis on vocabulary! I taught Algebra 1 to struggling students and noticed how important it was for them to be able to put words with the math steps. P.S. I don't think telling the kids "you'll live" is harsh – I'm always telling my own children "if that's the worst thing that happens to you today, you're having a pretty good day" when they complain about small things. 🙂
I teach grouping, the xbox (which is the x but with the grouping part done in a box), and the method but with just the x but with a slight variation. You may like it because it's a little less confusing. Instead of rewriting the equation because they have to divide the coefficient, just have them only divide the factors INSIDE the x, simplify/reduce those factors, and then set up their parenthesis with the whole numbers but do "bottoms up", as you call it, when writing the binomials, if a one of the factors is not divisible. I hpe you understand this. It's a tiny bit less confusing and less work 🙂
Interesting strategy. I used the box method to teach multiplying polynomials last year, but I've never used it for factoring.
This post is awesome. You clearly have very high standards for your students and that is so good to know! To factor trinomials with a leading factor greater than 1, I use the "magic number" method. 1: Find AC (magic number) 2: find what 2 numbers add to get AC. 3: replace B with these 2 factors. 4: group 5: factor each parenthesis. 6: rewrite as (inside stuff once)(outside stuff). The only weird part is needing to change the sign in the 2nd parenthesis sometimes. I made a silly video that I posted on my blog about it. Excuse the music, I used tho think I had skillz: https://web.archive.org/sry
Love the video! Thanks for sharing!
Great post! I love the "slide, divide, bottoms up" method. I did a similar method, but named it after another teacher from whom I learned it! At least your naming gives the kids clues about the steps! I love your interactive notebook ideas, too. I'm excited to have found you! Keep it up!
Thanks!
Great post! I used the Sum Product puzzles for the first time this year. Digging around in my Math Coach's closet I found this X Factoring book by Michael L. Flowers that I think the puzzles on the internet may be borrowed from him. What I found interesting about the book was that the puzzles were organized "upside down" from the ones online and scaffolded. By Upside Down, I mean the sum is at the top and the product is at the bottom. I first saw the puzzles maybe 5 years ago, but I was turned off by the product at the top. When transitioning to trinomials, the sum is generally in the middle and the product last. So with the puzzles from the book – I was able to wrap my mind around that idea a little easier. Also, the book modifies the puzzles where the side numbers are given and the product section is shaded out. So those pages give students practice with just adding the side numbers. I wish I had opened the book before I used some of the puzzles I found online.
From the puzzle, students can make the puzzle template by putting b on top and c on the bottom and solving the puzzles that have already been practiced!!!
Also, in the book, he addresses when a is not equal to 1. The students – CREATE their own puzzle with ac at the bottom and b at the top. Once they find the side numbers – the author suggests the AC split (of the middle term and factor by grouping). Which is what I teach. I have taught the Slide and Divide method, but felt a little uncomfortable explaining how the "trick" work. There is a method called "Chunking" I believe that I have read about in an NCTM publication on High School Mathematical Reasoning. I have also seen it in some of the Common Core and PAARC materials. Chunking puts me in mind of U-substitution when dealing with quadratics that are reducible to quadratic form. I like the chunking method, but I have only taught it to Algebra 1 students once. Unfortunately, it was 2 or 3 days after a my co-worker taught the Lizzie method which the students seemed to like more.
Thanks for sharing about the book. I'm going to have to check that out!
A friend showed me the chunking method again the other day, and I'm a convert. I've decided this is the way I'm going to teach it next year! In two years of teaching, I've taught factoring 4 different ways. I'm finally glad to find a way I love that doesn't rely on a trick. Thanks for sharing!
One thing you might want to change is saying that the difference of squares is only for quadratics (or degree 2 polynomials). Sometimes the difference of squares shows up in situations with logarithms, imaginary numbers, and higher degree polynomials.
I love your blog, and you create a lot of great materials! I have found that factoring by grouping is really helpful for students that struggle to remember the algorithm. For example, the Algebra 1 and Algebra 2 teachers teach the Divide/slide method. However, by the time the students get to me in Pre-Calculus, they have forgotten how to do it. I found the factoring by grouping tends to stick with them a little longer. I would love to see how you would teach factoring by grouping, or how you would set up a graphic organizer for that.
Great point about the difference of squares! I need to think about how to word that for next year. Hmmm…
And, thanks for your kind words about my blog. They mean a lot to me! A few months ago, I had a friend show me how to factor by grouping, and I fell in LOVE with the method. I've decided that I can never go back to sliding and dividing after learning the other way. In 2 years of teaching, I have now taught factoring 4 different ways. I've decided that factoring by grouping will be the way I teach from here on out.
I'm going to have to spend some time thinking about a graphic organizer for factoring by grouping, though.
Thanks for stopping by my blog!
The difference of squares can be the difference of any 2 squares.
I have been teaching factoring to Algebra students for many years, and nothing has worked better than factoring by grouping. They understand it and retain it! Many of my students last year wanted to use this method to factor trinomials even when a was 1. I actually enjoy teaching factoring now because of this approach! Good luck!
I'm so excited to teach factoring by grouping next year! I can't believe it took me so long to learn this method. Glad to hear that your students have had great success with it!
And, thanks for reading my blog. It means a lot to me!
Gosh, I have probably taught factoring by grouping for over 15 years. I believe the students retain it with this method because they can actually see the process of a reverse distributive property. By the time we reach factoring, they fully understand the distributive property and now with factoring, can see how it actually can be worked backwards.
Hi all, I feel your pain in trying to teach factoring. I teach college level math in a HS dual enrollment program on a college campus. I am not sure you appreciate how very important the skills you teach are in higher math. In all the calculus courses, 1, 2, and 3,and Differential Equations, factoring is an important skill. How many times I have been in the middle of a 3 page problem, and factoring comes up? By this time, most can do it, but even after 11 years of math, at least half of it algebra, some of the brightest kids in the county still can't factor, so they can finish the problem. They understand the calculus, but hang up an algebra skills. This slide divide is interesting, but IMHO it is a "wrote" method, meaning they have to memorize the steps and it does not give them an understanding of the concept. I love the AC method, also called grouping. To me, it is not whether they can get the answer today, it is whether they can understand the process for the long term. After finding you on Pinterest, and going to your blog, I have learned a lot. Cool method, but not sure it has staying power. Thank you for the insight. I will most likely post a handout on the AC method in the next few days. And to tell the truth, when some are stuck, they just go back to trial and error, so they can move on and finish the bigger problem. Visit my TpT store, lots of innovative material for all levels of Algebra and up. https://www.teacherspayteachers.com/Store/Joan-Kessler. If anyone emails me and mentions this blog, I will send for free. joan@distancemath.com. No blog yet, just an old-fashioed website, http://www.distancemath.com. Sorry this was so long, but so is my career 🙂
Thanks for the comment Joan! I'm still definitely learning when it comes to teaching factoring. A few months ago, a friend show me how to split the middle term and factor by grouping. Once I saw this I fell in LOVE. It works every single time and it's not a silly trick for students to remember. I liked Slide and Divide, but it always seemed a bit gimmicky. Still, it was better than the torture of guess and check. But, I'm definitely going to teach splitting the middle term and factoring by grouping next year. In 2 years of teaching, I've taught factoring 4 different ways. Hopefully this fourth way will do the trick!
P.S. Thanks for reading my blog. It means a lot to me!
Thanks for sharing your wonderful ideas!!!! I am working on planning my factoring unit, and was wondering how you teach factoring out the GCF. I haven't really seen much on this concept anywhere 🙁
Thank you!!!!!
I've tried it a couple of different ways, but I haven't found a way I'm happy with. Good luck!
I'm not sure what part of factoring out the GCF from polynomials you're working with, but if your students already know how to get the GCF and they just have a problem taking it out, I saw a video online that says to tell the students:
1) Write the GCF on the outside.
2) Think: (always a good thing 🙂 ) "What can I multiply the GCF by to get each term that I started out with?"
This tends to work with the way students think and doesn't get them mixed up by starting with dividing, etc…
I teach guess and check, factor by grouping, and the box method to factor. I let my students decide which method they prefer. The grouping and box method I teach for trinomials just reinforce those methods when they have to use those to factor higher order polynomials.
In classes past Algebra, I show the slide/divide as a shortcut. Slide and divide is not a trick. It works because (ax + b)(cx + d) = acx^2 + (ad+bc)x + bd = ac(x + b/a)(x+d/c).
Thanks for sharing!
Like others have mentioned in the above comments, I also teach factoring by grouping for factoring trinomials. I think I saw I later blog post where you mentioned that you would be trying it. 🙂 Good luck!
Also, I agree wholeheartedly about the FOIL method. I never teach it. I like teaching them that it's the distributive property because then it's an algorithm easily extended to other situations.
I'm about to start factoring, and I will definitely be using the Halloween Candy Tax story along with these INB pages for factoring notes (what to use when and the steps). I'm curious, would you be willing to share the editable doc of the factoring when a > 1 page so that I can easily add more rows to fit my method?
Thanks for blogging. You are inspiring me, and I'm in year 8 of teaching!
I LOVE YOUR BLOG!!! I'm a first-year teacher and your blog has been so wonderful and inspirational and just SUCH a HUGE help! I love it! Thank you for spending so much time to inform everyone else that is using your blog as resource!
I read some about factoring and the 'slide, divide, bottoms up' method… I saw in your Trig post that you were trying to find a different game plan for teaching factoring… I was just wondering how you decided to teach that! We are working on factoring now… and running out of time before EOCs… and my students will definitely struggle in memorizing the steps.
Hi Sarah!
I was wondering if you wouldn't mind emailing me these files to virginiaaculp[at]gmail.com? I can't seem to view them on your site.
Thanks!
Ginny
E-mail sent!
Thank you so much!!
if the question is 2x to the sixth is the name 6th degree
6th Degree Monomial
Does the slide, divide, bottoms up work for leading coefficients that are not prime numbers? I am having trouble with one where 6x^2 is the first term (and there is no greatest monomial factor). Thanks,
Kristina
I'm not sure! I've converted to just using the box method for factoring. My students seem to be able to remember it a lot better.
Hello!
Are there foldables available for perfect square trinomials?
I've never specifically taught those. Sorry!
I used the mistake method for factoring this year because that is what my department uses but I believe that I will switch back to factoring using the box next year. It is basically factoring with grouping but organizes it a little differently. The students can retain this easier, in my experience, especially if you teaching multiplying using boxes as well. I've decided that there is truly no great way to teach factoring that works for everyone.
My Algebra I students tend to struggle with polynomials in general. It seems to be even more of a struggle this year. I have found that using the foldables for guided notes help. I do show my students distributive, the box method, and foil when multiplying. I have students who find each of them easier than the others.
I have never used the box method for factoring. It is a good idea to have the students multiply a and c when a is 1 to have them form a habit. I waited this year and told them "Now we are going to add another step." I wish I would have done this from the beginning.
Yes! Definitely have them multiply a and c from the start. I didn't do this one year, and I immediately regretted it!
is there any way you could email me some tips on teaching factoring by grouping? it sounds like that is the most popular method to teach factoring trinomials. i've taught factoring by grouping with four terms, but not with 3. any tips or examples?