Linear Functions Stacking Cups Lesson

I need to invest in a t-shirt to wear while shopping.  It should say something along the lines of “Don’t ask.  I’m a math teacher.”

Surprisingly, the cashier at Dollar General did not question my need to buy 84 foam cups.  Maybe she thought I was throwing the party of the century or something???

Though, I did get a weird look from a fellow customer as I stood in the aisle, looking at all my foam cup options.  I may or may not have been measuring the cups in the store to determine how many packages to buy so that I would have enough cups to stack to equal my height.

This was a compilation of a lot of lessons I read about on various math teacher blogs.  I think I first learned about this cup stacking lesson via Dan Meyer.  Before carrying this out in my own classroom, I also read about the experiences of Fawn Nguyen, Andrew Stadel, Simplifying Radicals, and Elissa Miller.     

When students entered my classroom, they saw cups.  Foam cups everywhere.

Student: What are we doing today?  Are we having a party?
Me: No, we’re not having a party.
Student: Oh, I thought we were going to do something fun today.
Me: Ummm, hello!  This is math class; we do something fun everyday.
Student: Oh, Ms. Hagan!  You would say that!
Me: I say it because it’s true!  I have fun every single day.  
Student: Guys!  Don’t ask Ms. Hagan what we’re doing today because she won’t tell you.
Me: You’ll just have to wait and see!

After going over the bellwork, I broke the class up in pairs.  Each pair needed a calculator, dry erase board, marker, and eraser.  No rulers yet!

I held up the cup and asked, “How many cups would you have to stack to equal my height?”

How tall are you?

No questions.  Just write your answer on your dry erase board.

How tall are the cups?

Again, no questions.  Just do your best!

Would it help if I stood next to a cup?

The students said it would, and I’m going to use any reason I can to justify standing on top of the furniture.

Ignore the homecoming t-shirts on the desks.  This was my attempt at sorting homecoming shirts by size until their owners came to pick them up.  Let’s just say that homecoming t-shirts are probably my least favorite part of being in charge of student council.  They are also our major fundraiser of the year.  So much stress!

For the most part, the initial guesses were quite low.  10 cups.  12 cups.

I had each group write their answer on the dry erase board and reveal them all at once to keep groups from changing their guesses based on the guesses of other groups.  This worked well.

By now, students were dying to ask questions.  Dying.

As you can imagine, I was dying with happiness over this.

Now, you may ask questions.  But, be forewarned, I will only answer certain questions.

How tall are you?

I don’t know.

What do you mean you don’t know how tall you are?

There’s a measuring tape in the back of the classroom.  I don’t know how tall I am, but you can certainly measure how tall I am.

Last summer, my mom picked me up several tubs of measuring tools at a yard sale from a teacher who was retiring.  These have been an amazing resource this year!

I taped two of these measuring tapes to the back wall with some electrical tape that I found in my cabinet.  I intentionally chose the centimeter side of the measuring tape.  What can I say?  I’m mean like that.

Centimeters?!?!?  Why can’t we use inches?!?!?!

My response?  You’ll live!

Students also had to take into account that after 150 centimeters, the numbers started back over.  This threw a few of my students for a loop!

Again, I didn’t tell them how tall I was.  Instead, I invited any students who wanted to measure my height to the back of the classroom.  Usually, one student would come.  I would stand with my back to the wall and invite them to tell the class my height.

In case you are wondering, I am 172 cm tall.

After the student would announce my height to the class, I would ask the students if they trusted this student’s measuring skill.  If not, they were welcome to come and confirm my height for themselves.  In every class, at least one student took me up on the offer to confirm.

Okay.  Next question.

How many cups would you have to stack to equal 172 centimeters?

Ha ha.  Nice try.  That’s the question you’re supposed to be answering.

How tall is a cup?

I can do you something better than answering that.  I can give each group one cup.

After giving each group a single cup, they began begging for rulers.  Students begging to do math?  I could get used to this!

You guys know where the rulers are at.  Get busy!

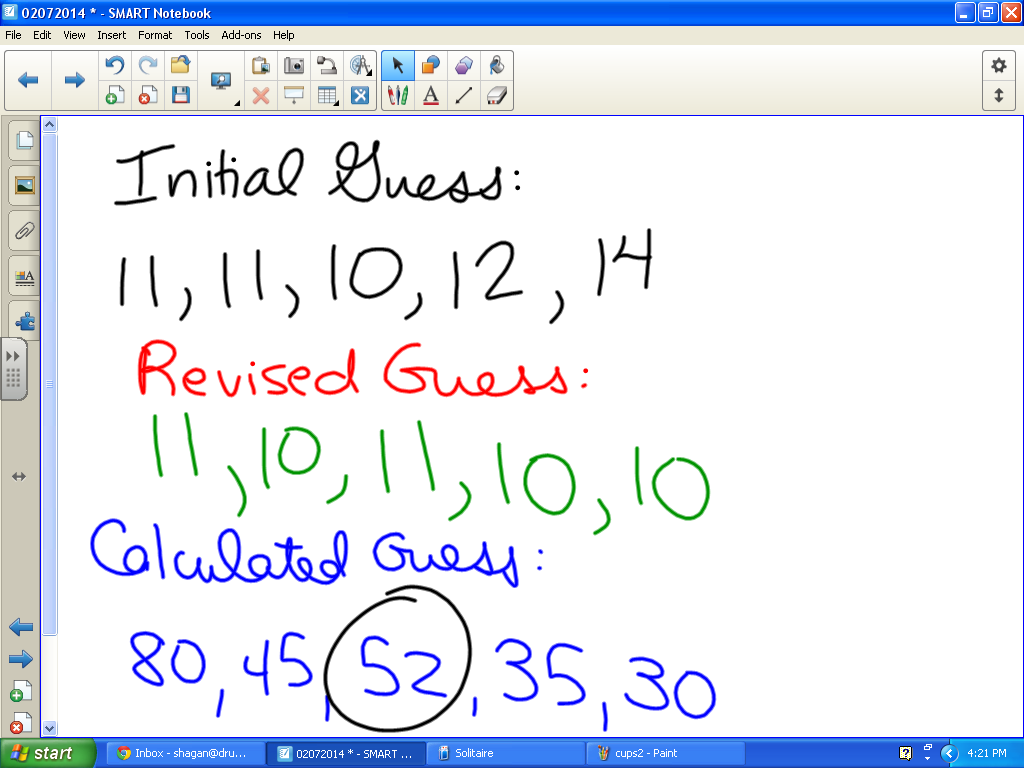
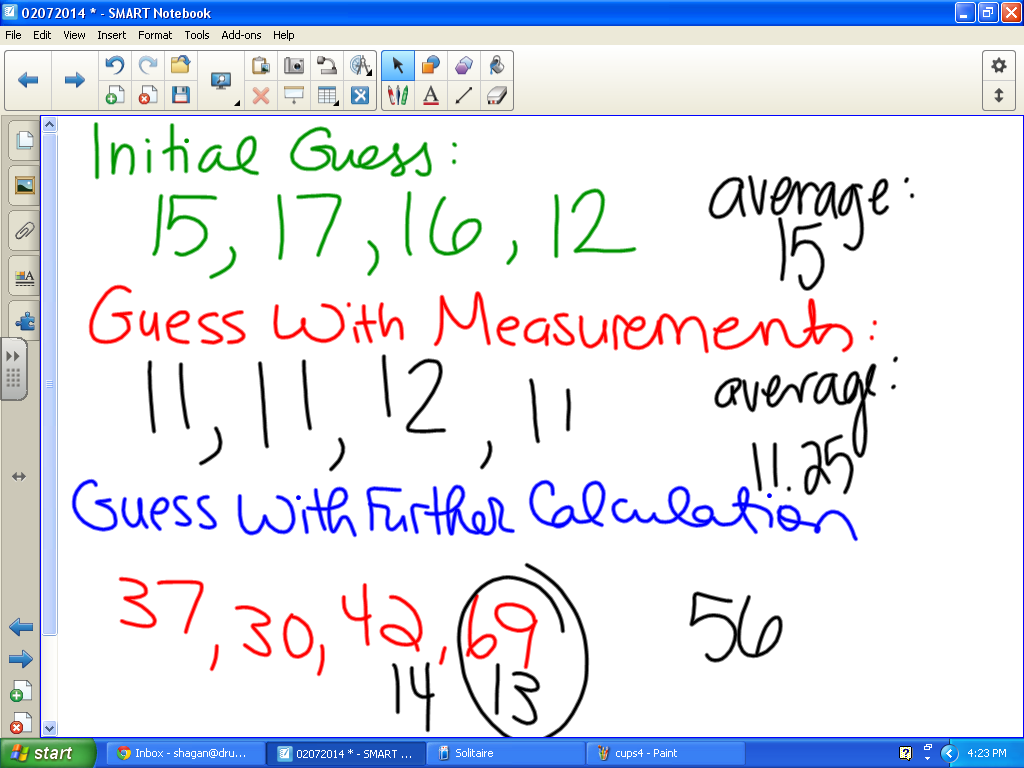
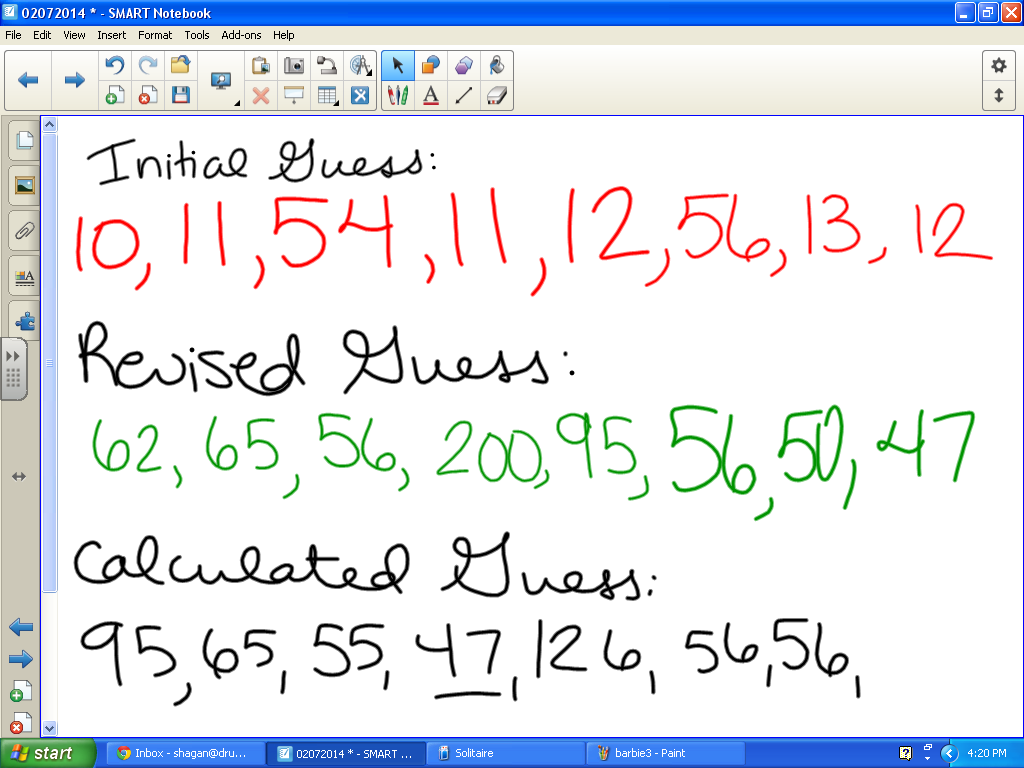
Soon, with the help of a ruler, they came up with their revised guesses.  For two of my classes, their revised guesses were still between 10-12 cups.  My last period of the day had revised guesses between 47 and 200 cups!

After they made their guesses by holding up their boards in unison, I recorded the guesses on the board.  Then, I began stacking cups, one inside another.

Ten cups guys?  You really think I’m ten cups tall?  I’m pretty sure I’m a lot taller than ten cups.

The students were outraged.

That’s not fair!  You didn’t tell us we were stacking them inside of one another.

Do you know how my students got the guesses they did?  Most of them took my height and divided by the height of the cup.  That works great only if you invert every other cup while stacking.

Immediately, they wanted a redo.  This time, I offered them five cups.  I told them this was their last chance.  The pair of students who guessed the closest this time would win their choice of a bottle of pop or a candy bar.

Students quickly got to work measuring and punching numbers in their calculators.

I loved hearing all the debate going on between the partners.  But, every time we add a cup, the height of the stack only goes up by the height of the cup’s lip.

Yes.  Yes.  Yes.

I had to try hard to contain my excitement.

One of my students decided to graph the data.  I was happy to see students using tools that I make available to them!

The calculated guesses were much better, but my students still needed a lot of work with linear functions!  I used this lesson as an introduction to linear functions.  Ideally, my students have worked with slope-intercept form in middle school, but the majority of our students come to high school Algebra 1 with basically zero understanding of slope or graphing lines.  Beyond that, probably over half of my students did not know how to solve a two step equation for the variable.  And, let’s not even get started on integer operations.  In a larger school district, these students would be placed in a pre-algebra class.  But, I teach at such a small school that we offer no high school classes below Algebra 1.  All freshmen take Algebra 1 regardless of their readiness.  (The few students who took Algebra 1 as eighth graders take Geometry.)  It’s up to me to review and reteach pre-algebra concepts in addition to my Algebra 1 standards.  

With the potential transition to common core next year, this should be interesting.

I started out with the lowest final guess and stacked that many cups.  As the lower guesses were dismissed and the stack of cups grew taller, I had a volunteer come to the front to start handing me cups.

This volunteer is very excited because her group’s guess is getting awfully close to my height.

Different classes disagreed on the number of cups to equal my height.  Two classes found it to be 56, but my last class said that 56 cups were technically taller than me so it had to be 55.

Here’s me and my stack of cups.

I convinced a couple of my students to photograph this activity for me.  In addition to the photos of me standing on the desks, they also left me with a couple of selfies and this random picture of me in action at the SMART Board.

Next, we walked through how you could use algebra and slope-intercept form to determine the necessary number of cups.  We discussed and measured the two parts of the cup.  After substituting the heights of each part of the cup and my height, we were ready to solve for x, the number of cups needed.

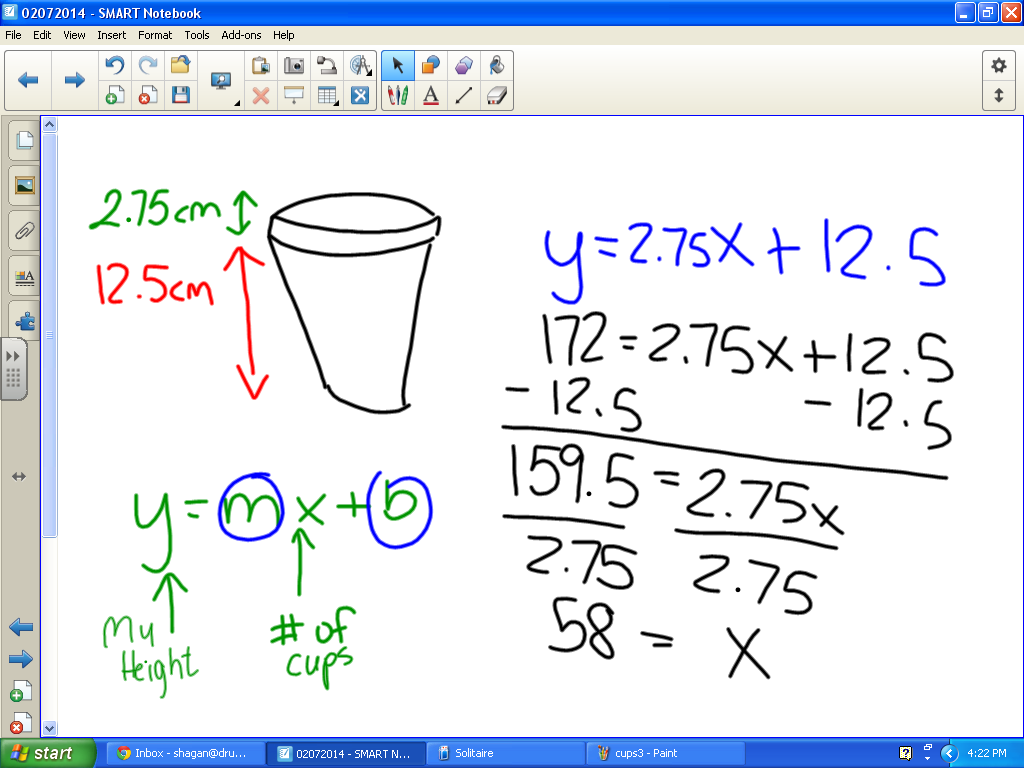
By this time, our fifty minute class period was winding to a close, so I didn’t have time to pose any follow up questions.

I enjoyed this lesson, but I wish I had spent two days on it.  I wish I had students complete some sort of reflection/extension on the project in their interactive notebooks.

Here’s the data from my three classes.

More Activities for Teaching Linear Functions

• 
  Silent Board Game for Finding Function Rules
• 
  Graphing Linear Equations Activity with Transparencies
• 
  Real-World Situations for Linear Functions
• 
  Transformations of Linear Functions Foldable
• 
  Writing Linear Equations Graphic Organizer
• 
  Turkeys in the Oven Game – Writing Linear Equations
• 
  Graphing Linear Equations Practice Book
• 
  Linear Functions Stacking Cups Lesson
• 
  Linear Equation of the Day Routine